IR-UWB系统中不同收发器的TOA估计分析

5星 · 超过95%的资源需积分: 10 9 浏览量更新于2024-09-17 收藏 587KB PDF 举报

"这篇文献探讨了不同类型收发器在IR-UWB（Impulse Radio Ultra-Wideband）系统中用于TOA（Time-of-Arrival，到达时间）估计的方法。研究了存储参考、传输参考（Transmitted-Reference, TR）和能量检测（Energy-Detection, ED）基础的TOA估计算法的性能，尤其是在亚奈奎斯特采样率下的表现。文章首先考虑了一个加性高斯白噪声信道，以此强调这些不同收发器的基本问题。接着，分析了能量收集特性以及决策统计，对每个收发器的准确峰值检测概率进行了分析，并推导出了前沿检测的接收机操作特性。此外，还深入研究了每个符号的脉冲数量和平均符号数量的影响。随后，文章转向了真实的多径信道场景，探讨了各种最大似然估计方法。最后，提出了一种新的估计器设计。" 本篇论文的核心知识点包括： 1. TOA估计：TOA估计是无线通信中的关键技术，用于确定信号从发送端到接收端的传播时间，从而实现定位或时钟同步。在UWB系统中，TOA估计对于提高系统性能至关重要。 2. IR-UWB系统：IR-UWB利用极短的脉冲进行通信，具有低功率、高精度和抗多径干扰的能力，广泛应用于室内定位、无线传感器网络等。 3. 不同类型的收发器：存储参考、传输参考和能量检测是三种不同的收发策略。存储参考利用预存的参考信号进行比较，传输参考依赖于发射端和接收端的共同参考信号，而能量检测则基于接收到的信号总能量进行判断。 4. 亚奈奎斯特采样率：在低于奈奎斯特采样率的情况下工作可以降低系统复杂性和功耗，但也会引入额外的挑战，如信号恢复和噪声放大。 5. 加性高斯白噪声信道：这是通信系统中常见的模型，用于模拟信号在传输过程中受到的随机噪声影响。 6. 能量收集特性：分析接收机如何有效地收集和利用信号能量，这对于TOA估计的准确性至关重要。 7. 决策统计：研究统计方法如何帮助决策过程，例如在检测信号的前沿时，选择最佳的估计值。 8. 峰值检测概率：分析不同收发器在噪声环境中正确检测信号峰值的概率，这是TOA估计的关键步骤。 9. 多径信道：真实环境中的多径传播会导致信号的衰落和多普勒效应，需要采用适当的估计方法来克服这些问题。 10. 最大似然估计：一种常用的参数估计方法，通过寻找最可能产生观测数据的参数值来进行估计，在多径信道中尤为适用。 11. 新提出的估计器：文献中提出的新方法可能是针对特定问题或性能优化的一种改进方案，具体细节需要查阅原文以获取更多信息。这篇论文全面地评估了IR-UWB系统中不同TOA估计策略的性能，对于理解和优化UWB通信系统的时序估计具有重要的理论与实践价值。

1878 IEEE TRANSACTIONS ON MICROWAVE THEORY AND TECHNIQUES, VOL. 54, NO. 4, APRIL 2006

The samples at the output of the square-law device are

(5)

while the samples after correlating with the delayed version of

the signal itself are formulated as

(6)

In the TR case as well, the absolute values of the samples are

used to yield

. The performance can be further im-

proved by having a processing gain from multiple symbol trans-

missions. Note that averaging must be performed prior to abso-

lute-value operation. In what follows, we refer to the number of

symbols as

B. Tradeoffs Between Different Transceiver Architectures

It is very well known that matched ﬁltering, in which an SR

template is correlated with the received signal, is an optimal de-

tection technique when the knowledge of the received waveform

shape is available. However, Nyquist-rate sampling is essential

to match with the received signal, so that perfect alignment with

the template and received waveform can be obtained. If only

lower sampling rates are possible, it is apparent from (4) that the

SR will not be able to collect sufﬁcient energy from the received

multipath arrivals due to timing mismatches (and pulse-shape

distortions).

On the other hand, ED and TR signaling can both effectively

capture the received energy. Even at sub-Nyquist sampling

rates, neither scheme requires the knowledge of the sampling

timing or pulse shapes (assuming accurate delay lines for

the TR case). The existence of the TR pulse yields a 3-dB

transmitted energy loss compared with the other two schemes.

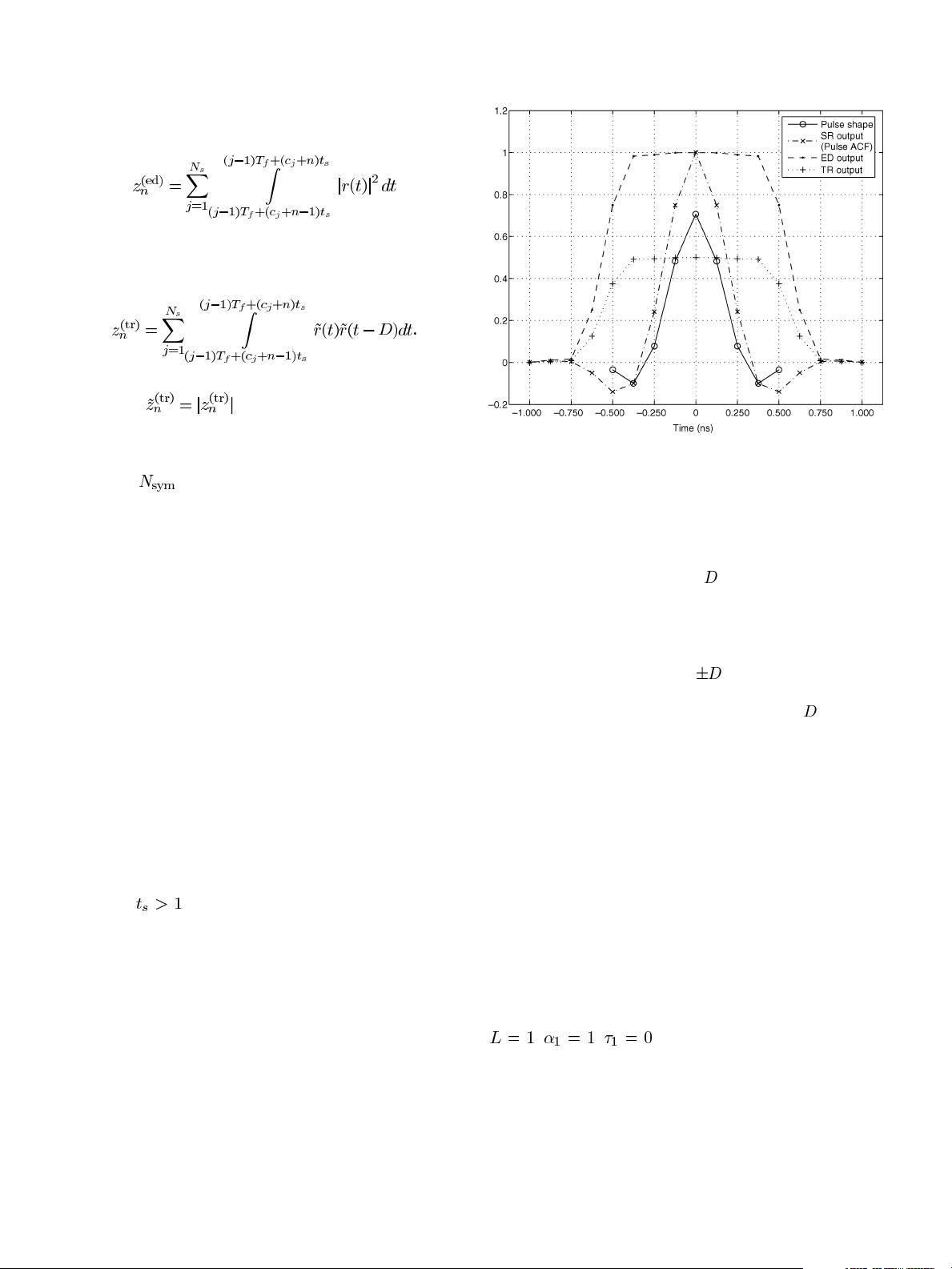
Illustration of the timing susceptibility for SR, TR, and ED are

presented in Fig. 3. If a sufﬁcient sampling rate is available, the

SR will better characterize the peak; however, at low sampling

rates (e.g.,

ns), it becomes more likely for SR to miss

the peak.

Note that in noncoherent approaches the enhanced noise

terms in the low SNR region becomes an issue. In particular,

noise-square terms for the ED and noise-cross-noise terms for

TR seriously dominate and degrade the detection performance.

Therefore, even though a noncoherent approach outperforms

the SR at high SNR due to better energy capture at sub-Nyquist

sampling rates, they have poor performance when the noise

variance is large. Moreover, noncoherent schemes suffer from

degraded SNR when symbol energy is spread over more pulses,

whereas energy in an SR symbol can be distributed over mul-

tiple pulses with no SNR loss. Due to similar reasons, TR and

ED are much more susceptible to interference compared to SR.

A cross-correlation receiver can also be considered instead of

TR (as in [13]), which yields identical energy capture with ED

but a smaller noise variance.

Fig. 3. Received normalized pulse shape and the sampled outputs corre-

sponding to SR, ED, and TR for different timing offsets (1-ns pulse is sampled

at 8 GHz, and energy is collected within 1-ns windows and different time

offsets).

Comparing the transmitted waveforms, TR has a longer time

span compared to ED and SR, and

has to be sufﬁciently large

so that multipath interference between reference and data pulses

is not a serious problem. Also, TR observes enhanced early/

late (E/L) noise terms that arise when either the reference or

data signal samples are correlated with the noise-only samples.

This scales the noise variance at

of the actual TOA by the

signal energy. Finally, the delayed signal from a previous frame

may affect the results for TR receivers, and the

parameter

should be selected carefully considering the frame duration and

the maximum excess delay of the channel.

In the next sections, ﬁrst, an AWGN channel is considered

to highlight certain points related to different transceiver types.

Some of the fundamental tradeoffs discussed in this section

are expanded in detail. Later, under realistic multipath channel

models, maximum-likelihood techniques for TOA estimation

are given.

III. AWGN C

HANNEL ANALYSIS

UWB receivers typically observe dispersive channels that

have hundreds of multipath components. However, it is more

useful to analyze certain fundamental aspects related to signal

acquisition and leading edge detection in less complicated

scenarios. In this section, we consider an AWGN channel

(

, , ) and analyze performance tradeoffs

between different transceiver types. In particular, decision

statistics (for single and multiple pulses per symbol), as well

as energy collection characteristics under timing mismatch

(due to sub-Nyquist sampling) are investigated in detail. Peak

selection performances are compared for the three schemes,

which are closely tied with signal acquisition. Then, leading

edge detection characteristics are analyzed by conditioning

the detection probability on the leading edge energy, which

depends on the transceiver’s energy collection capability.

剩余10页未读，继续阅读

ustc1023

粉丝: 0
资源: 2

IR-UWB系统中不同收发器的TOA估计分析

归一化门限下的IR-UWB系统TOA精确估计算法

时间平均TMMR-TC算法：提升IR-UWB非相干TOA估计精度

优化动态门限的IR-UWB TOA估计算法提升无线传感器网络性能

IR-UWB系统中基于root-MUSIC算法的TOA和DOA联合估计

IR-UWB系统中高效根MUSIC算法的TOA-DOA联合估计

IR-UWB系统的matlab代码

UWB实现技术对比：IR-UWB、DS-UWB与MB-UWB

双门限TOA估计：IR-UWB非视距定位的高精度策略

MIMO MB-IR-UWB系统信道容量优化与仿真研究

MIMO MB-IR-UWB系统中DSTFC的实现与性能分析

最新资源