模态分析入门：操作模态分析原理与工程应用

需积分: 10 122 浏览量更新于2024-07-17 收藏 13.91MB PDF 举报

模态分析是结构工程领域中的关键工具，用于研究在环境振动或正常运行条件下系统的行为特性。操作模态分析(OMA)作为一个专业工程方法，它通过对数学模型的动态特性和从实验测量中获取的实际系统属性进行关联，来识别结构系统的模态性质。本资源深入介绍了模态分析的概念和理论，特别关注于操作模态分析，由Rune Brincker和Carlos E. Ventura两位专家共同撰写，出版于2015年。在本书中，作者首先对模态分析的历史背景和重要性进行了概述，强调了它在设计和维护过程中对于结构系统健康监测、性能评估以及动态响应预测的重要性。他们解释说，通过OMA，工程师能够识别出系统的关键模态频率、模态形状以及模态阻尼，这些都是决定结构稳定性与响应的关键参数。 OMA技术通常涉及实验数据采集，如振动测试或响应谱分析，这些数据被用来构建结构的有限元模型或者频域解析模型。随后，通过比较理论模型和实验数据，可以使用诸如频率响应函数(FFT)、模态识别算法（例如哈密顿方程解法、频率域或时域辨识方法）等技术来估计模态参数。这一过程不仅限于静态结构，还包括动态系统，如机械装置、桥梁、建筑物或航空航天组件。书中详细讲解了各种模态识别技术的实施步骤，包括但不限于频域数据处理、滤波、特征提取、模态参数估计以及不确定性分析。此外，章节还讨论了模态试验设计、噪声和环境影响的考虑，以及如何处理实际应用中可能出现的复杂性，如非线性行为、多模态系统和混杂效应。对于那些希望在结构工程、土木工程、机械工程或航空航天领域从事模态分析工作的专业人士而言，这本书是一本宝贵的参考资源。通过学习本书，读者不仅可以掌握理论知识，还能了解到如何在实践中有效地应用OMA技术，确保结构的安全和优化性能。模态分析是现代工程实践中的一个重要分支，其深入理解和应用对于提升结构工程项目的可靠性和效率至关重要。

Introduction 5

can be used to determine natural frequencies, mode shapes, and damping values for the structure’s

principal modes.

1.4 Vibration Testing of Civil Engineering Structures

What makes testing of large civil engineering structures different than testing mechanical systems? As we

have just discussed, the obvious answer to this question is that the forces are larger and the frequencies are

lower in large structures. But there is more than that. First, in general, analytical models of existing large

structures are based on geometric properties taken from design or construction drawings and material

properties obtained from small specimens obtained from the structure. A series of assumptions are also

made to account for the surrounding medium and its interaction with the structure (such as soil-structure

interaction in the case of buildings and bridges, and soil–water–structure interaction in the case of dams,

wharves, and bridges) and the composite behavior of structural elements. This, in general, is not the

case for mechanical systems. And second, in the eld of mechanical engineering, there are a number

of integrated systems that can handle very efciently the experimental testing, system identication,

and model renement. These integrated systems are very sophisticated as they combine the results of

several decades of research in the eld. Due to their relatively small size, most mechanical specimens

can be tested in laboratories under controlled conditions. There is no such advantage for the verication

of dynamic models of large civil engineering structures.

During normal operating conditions, a building is subjected to ambient vibrations generated by wind,

occupants, ventilation equipment, and so on. As we have argued earlier, a key assumption of the analysis

of these ambient vibrations is that the inputs causing motion have “nearly” white noise characteristics

in the frequency range of interest. This assumption implies that the input loads are not driving the sys-

tem at any particular frequency and therefore any identied frequency associated with signicant strong

response reects structural modal response. However, in reality, some of the ambient disturbances, such

as, for instance, an adjacent machine operating at a particular frequency may drive the structure at that

frequency. In this case, the deformed shapes of the structure at such driving frequencies are called oper-

ational modes or operational deection shapes. This means that a crucial requirement of methods to

analyze ambient vibration data is the ability to distinguish the natural structural modes from any imposed

operational modes.

The integrated systems, developed for mechanical engineering applications are not practical and eco-

nomical to test large civil engineering structures. Bridges form vital links in transportation networks and

therefore a trafc shutdown required to conduct a forced vibration test would be costly. Controlled forced

vibration tests of buildings may disturb the occupants and may have to be conducted after working hours,

thus increasing the cost of the testing. Therefore, routine dynamic tests of bridges and buildings must be

based on ambient methods, which do not interfere with the normal operation of the structure.

1.5 Parameter Estimation Techniques

The methods that have been developed for analyzing data from forced and in-operation vibration tests

range from linear deterministic models to nonlinear stochastic models. The applications range from

improving mathematical models of systems to damage detection, to identifying the input of a system

for controlling its response. Parameter estimation methods using dynamic signals can be classied as

(a) time-domain methods

(b) frequency-domain methods

The theory behind the rst two methods is described in more detail in this book.

6 Introduction to Operational Modal Analysis

1.6 Brief History of OMA

Although very signicant advances in OMA testing techniques have occurred since the early 1990s,

there is a wealth of information about different uses of OMA since the 1930s. Even ancient history

shows evidence of the use of the OMA concepts to better understand why and how structures vibrate.

Pythagoras is usually assumed to be the rst Greek philosopher to study the origin of musical sound.

He is supposed to have discovered that of two stretched strings fastened at the ends the higher note is

emitted by the shorter one. He also noted that if one has twice the length the other, the shorter will emit

a note an octave above the other. Galileo is considered the founder of modern Physics and in his book

“Discourses Concerning Two New Sciences” in 1638: At the very end of the “First Day,” Galileo has

a very remarkable discussion of the vibration of bodies. He describes the phenomenon of sympathetic

vibrations or resonance by which vibrations of one body can produce similar vibrations in another distant

body. He also did an interesting comparison between the vibrations of strings and pendulums in order to

understand the reason why sounds of certain frequencies appear to the ear to combine pleasantly whereas

others are discordant.

Daniel Bernoulli’s publication of the Berlin Academy in 1755 showed that it is possible for a string

to vibrate in such a way that a multitude of simple harmonic oscillations are present at the same time

and that each contributes independently to the resultant vibration, the displacement at any point of the

string at any instant being the algebraic sum of the displacements for each simple harmonic at each node.

This is what is called the Principle of “Coexistence,” which is what we know today as the Superpo-

sition Principle. Today, we also refer to this as the method of Modal Superposition. Joseph Fourier’s

publication “Analytical Theory of Heat” in 1822 presents the development of his well-known theorem

on this type of expansion. Isaac Newton in the second book of his “Principia” in 1687 made the rst

serious attempt to present a theory of wave propagation. John Strutt, 3rd Baron Rayleigh (1842– 1919)

through his investigations of sound and vibration provided the basis for modern structural dynamics

and how mass, stiffness and damping are interrelated and determine the dynamic characteristics of a

structural system.

The rst studies on shocks and vibrations affecting civil engineering structures in the twentieth century

were carried out at the beginning of the 1930s to improve the behavior of buildings during earthquakes.

M.A. Biot introduced the concept of the shock spectrum to characterize the response of buildings to earth-

quakes and to compare their severity. G. Housner, rened the concept by dening it as the shock response

spectrum (SRS) to clearly identify that it characterizes the response of a linear one-degree-of-freedom

system subjected to a prescribed ground shaking. After the 1933 Long Beach earthquake in California,

in 1935, D.S. Carder conducted tests of ambient vibrations in more than 200 buildings and applied rudi-

mentary OMA techniques to determine the natural modes of vibrations of these buildings. The results

of this investigation were used in the design codes to estimate natural frequencies of new buildings. The

seminal work of M. Trifunac in 1972 showed that the analysis of ambient and forced vibrations led to

the same results for practical engineering purposes.

The development of OMA techniques since the mid-1990s can be followed by reading the proceedings

of the annual International Modal Analysis Conference (www.sem.org) and, most recently, those from

the International Operational Modal Analysis Conference (www.iomac.dk).

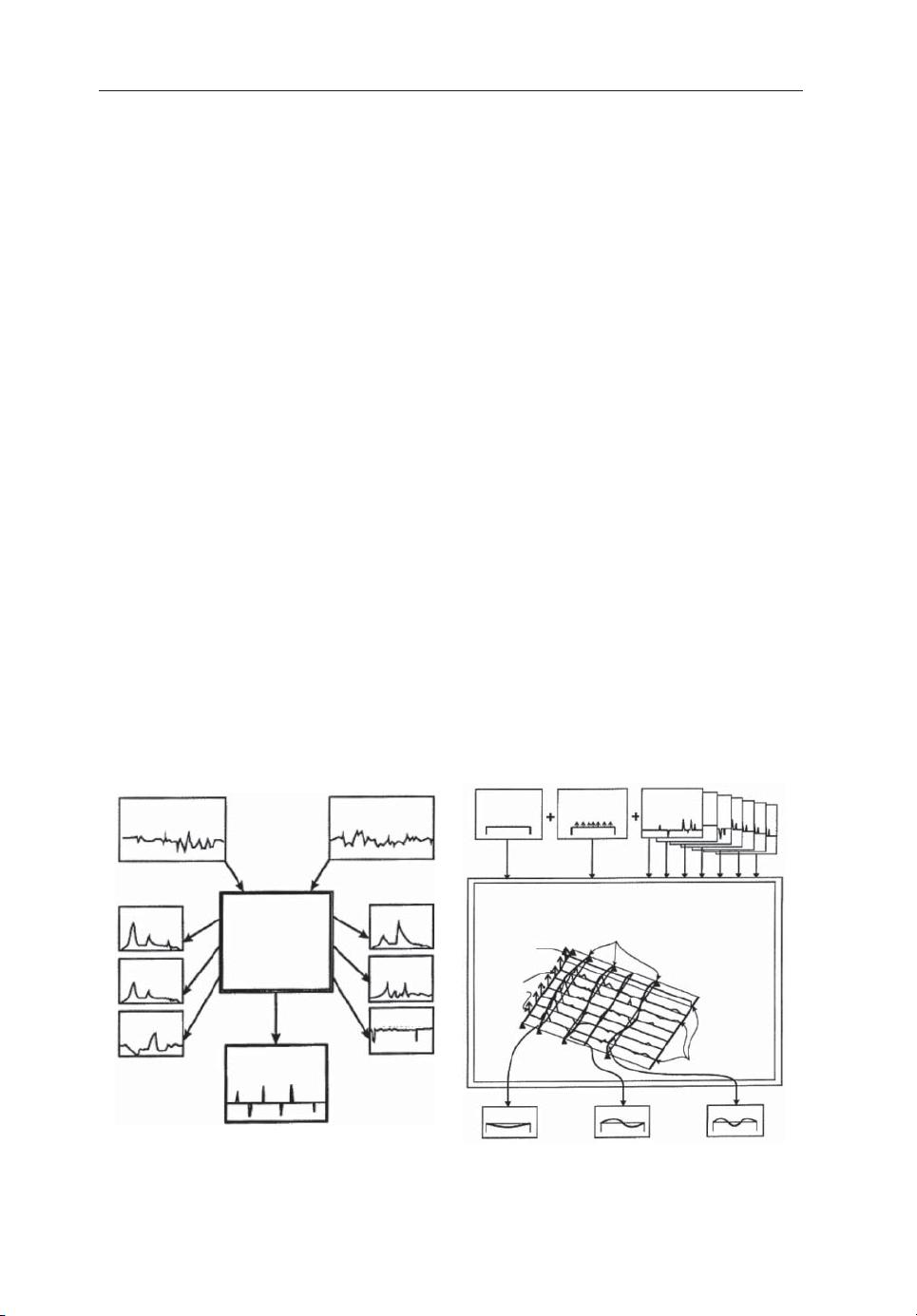
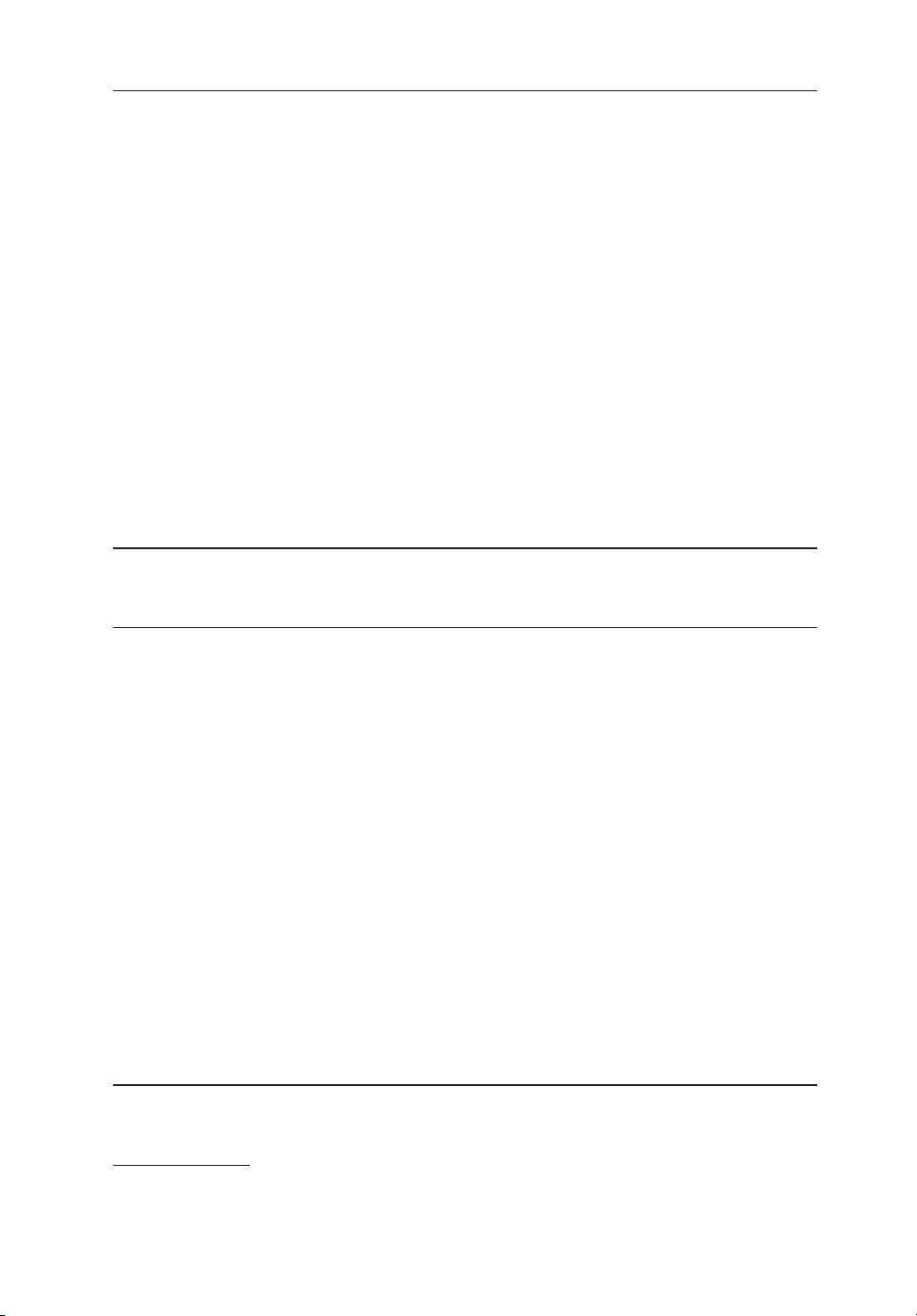
1.7 Modal Parameter Estimation Techniques

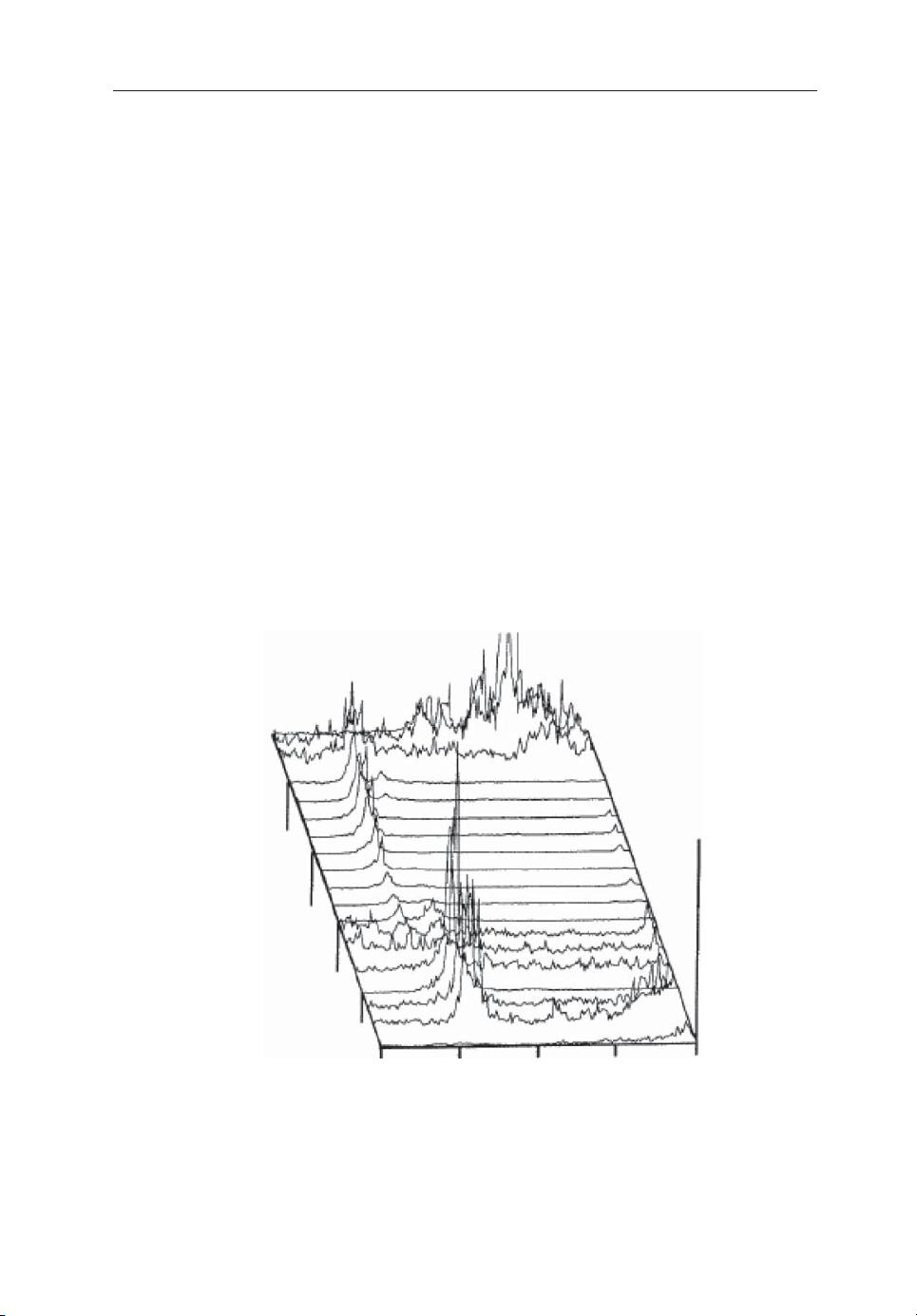
In contrast to EMA, OMA testing does not require any controlled excitation. Instead, the response

of the structure to “ambient” excitation sources such as wind, trafc on or beneath the structure, and

microtremors is recorded. Many existing textbooks provide an extensive overview of input–output modal

parameter estimation methods. See for instance, Heylen et al. [2] and Ewins [3]. In the operational case,

ignoring the need to measure the input is justied by the assumption that the input does not contain any

Introduction 7

specic information, or expressed in other words, the input is approximately white noise. As with EMA,

the measured time signals can be processed in the time domain or in the frequency domain. Since the

forcing function is unknown, frequency response functions between force and response signals cannot be

calculated. Instead, the analysis relies on correlation functions and spectral density functions estimated

from the operational responses.

Further, since OMA sensors and cables can be expensive, a limited number of sensors are used and

some of these sensors are roved over the structure to obtain several data sets. In order to be able to

assemble mode shapes using the parts of the mode shape estimated by the different data sets, some of

the response signals are declared as reference signals. The reference sensors are kept in the same place

when recording all data sets while the remaining sensors are moved progressively over the structure.

A fast method to estimate modal parameters from OMA tests is the rather simple “peak-picking”

frequency-domain technique. This technique has been used extensively over the years for all kinds of

applications. The basic idea of the peak-picking technique is that when a structure is subjected to ambient

excitations, it will have strong responses near its natural frequencies. These frequencies can be identied

from the peaks in the power spectral densities (PSD) computed for the time histories recorded at the

measurement points. This concept is illustrated in the example presented in Figure 1.2. The signicant

peaks of the PSDs for the OMA measurements conducted on a ve-span bridge can be associated with

the natural frequencies of vertical vibrations of this bridge.

The method has been widely used for many years. One practical implementation of this method was

developed by Felber [4] and will be used here as an example of the application of early OMA techniques.

In this implementation, the natural frequencies are determined as the peaks of the averaged normalized

West

abutment

East

abutment

Bent #1

PSD

Frequenc

(Hz)

Bent #2

Bent #3

Bent #4

23 45 6

Figure 1.2 Example of peak-picking technique for identication of natural frequencies of vertical

vibration of a ve-span bridge (Source: courtesy of Felber [4])

8 Introduction to Operational Modal Analysis

power spectral densities (ANPSDs). It is then assumed that the coherence function computed for two

simultaneously recorded response signals has values close to one at the natural frequencies; see Bendat

and Piersol [5]. This also helps to decide which frequencies can be considered as natural frequencies for

the structure. The components of the mode shapes are determined by the values of the transfer functions

obtained at the natural frequencies. It should be noted that in the context of ambient testing, transfer

function does not mean the ratio of response over force, but rather the ratio of the response measured at a

location of the structure with respect to the reference sensor. Every transfer function yields a mode shape

component at a natural frequency. In this method it is assumed, however, that the dynamic response at

resonance is only determined, or controlled by one mode. The validity of this assumption increases when

modes are well separated and when the modal damping is low (see more about this in Section 10.2).

Felber implemented a novel procedure based on this idea to expedite the modal identication of ambi-

ent vibration data, and this seminal work was the motivation for the development of interactive techniques

for fast and efcient implementation of the peak-picking technique. Three programs were used for the

process, one to generate the ANPSDs for the identication of natural frequencies, another to compute

the transfer functions between sensors (program ULTRA) and the third to visualize and animate the

mode shapes (program VISUAL). All three programs were used for preliminary analysis in the eld and

as well as for further analysis in the ofce. Figure 1.3 shows a owchart of the implementation of the

methodology developed by Felber.

Powerful time-domain techniques for operational identication have been developed over the years

and have gained tremendous popularity in the last three decades. The Ibrahim Time Domain (ITD) was

the rst, Ibrahim and Milkulcik [6], and shortly after came the Polyreference, Vold et al. [7] and the eigen

realization algorithm (ERA), Juan and Pappa [8]. The two last techniques were developed for multiple

inputs, and the ITD can also be formulated for multiple inputs (see Section 9.4). What they have in

common is that they assume that a free response function can be obtained from each record, and this is

the basis for the implementation of the algorithms to deal with the responses to either single or multiple

inputs using the correlation functions of the measured operational responses.

During the 1990s two important techniques became available: the stochastic subspace identication

(SSI) technique by Van Overschee and De Moor [9] and the frequency-domain decomposition (FDD)

Acceleration

time history

Acceleration

time history

Structure

geometry

Measurement

setups

Potential

modal ratio

functions

PSD of A

PSD of B

Cross

spectrum

Transfer

response

Coherence

function

Phase

function

Potential

modal ratio

function

Program

Batch mode

menu mode

ULTRA

VISUAL

Assemble and animate potential modal ratios

Reference

sensor

Frequency (Hz)

Other

sensor

locations

Veried experimental

mode shapes

Potential modal

amplitude ratios

Figure 1.3 Example of peak-picking technique (Source: as developed by Felber [4])

Introduction 9

technique by Brincker et al. [10]. And after the millennium, the PolyMax

technique by Guillaume et al.

[11] was introduced to the engineering community.

It is interesting to note that most of the classical input–output methods can be readily modied to be

used for the operational case. For instance, frequency response function (FRF) driven methods can be

converted to spectrum-driven methods and impulse response function (IRF) driven methods can be used

together with correlation functions instead.

The accelerations of a structure associated with ambient excitations are typically very small, generally

in the order of tens to hundreds of milli-g’s as shown in Table 1.2, and can vary considerably during

acquisition, for instance, depending on whether a truck, a car, or no trafc is passing a bridge at a certain

speed. This causes challenges to the sensors, the acquisition system and the identication algorithms that

must be able to extract weakly excited modes from noisy data. The developments in recent years both on

the acquisition side and on the identication side greatly enhanced the use of ambient vibration testing

to estimate the modal parameters of a large civil engineering structure.

Next to hardware and software, the judgment and experience of the modal analyst plays a role in the

success of the modal parameter estimation. Research into the automation of this process, so that any user

interaction could be excluded, is certainly useful.

Table 1.2 General characteristics of structural response

Vibration excitation Frequency

range

(Hz)

Displacement

range

(μm)

Velocity

range

(mm/s)

Acceleration

range

(μg)

Suggested

measuring

quantity

Trafc: road, rail,

ground-borne

1–80 1–200 0.2–50 2–100 Velocity

Blasting vibration

ground-borne

1–300 100–2500 0.2–500 2–500 Velocity

Pile driving

ground-borne

1–100 10–50 0.2–50 2–200 Velocity

Machinery outside

ground-borne

1–300 10–1000 0.2–50 2–100 Velocity/

Acceleration

Acoustic: trafc,

machinery outside

10–250 1–1100 0.2–30 2–100 Velocity/

Acceleration

Air over pressure 1–40 Velocity

Machinery inside 1–1000 1–100 0.2–30 2–100 Velocity/

Acceleration

Human activities

a. impact

b. direct

0.1–100

0.1–12

100–500

100–5000

0.2–20

0.2–5.0

2–500

2–20

Velocity

Acceleration

Earthquakes 0.1–30 10–10

0.2–400 2–2000 Velocity/

Acceleration

Wind 0.1–10 10–10

Acceleration

Acoustic inside 5–500

Source: Adapted from American National Standard: “Vibration of Buildings – guidelines for the mea-

surement of vibrations and their effects on buildings,” ANSI S2.47-1990 (ASA 95-1990).

PolyMax is a trademark of LMS International.

剩余361页未读，继续阅读

augustus93

粉丝: 0
资源: 2

模态分析入门：操作模态分析原理与工程应用

模态空间-NVH工程师的技术结晶

模态空间系列

书籍模态分析技术。。。

多模态深度学习综述.pdf

208TD说明书.pdf

遗传算法任务书.pdf

模态分析理论与应用.pdf

百度知识中台白皮书.pdf

2021百度智慧城市白皮书.pdf

百度大脑AI技术成果白皮书.pdf

最新资源