"解密约束满足问题:形式化与高效算法"
需积分: 0 185 浏览量
更新于2024-03-14
收藏 8.8MB PDF 举报
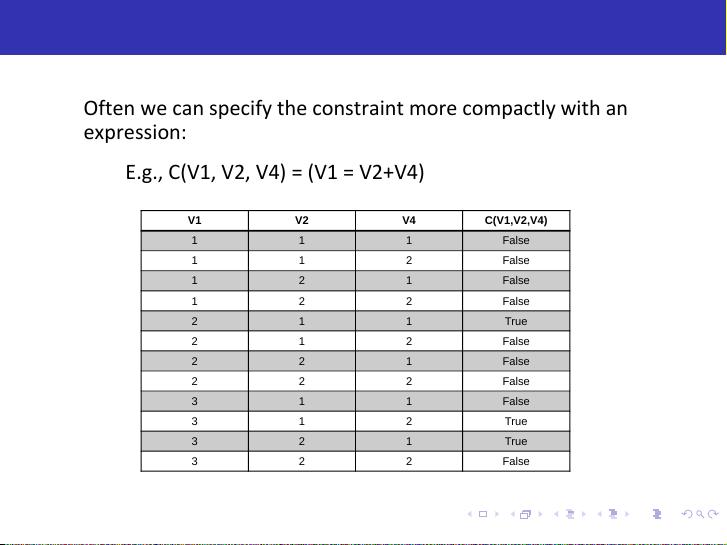
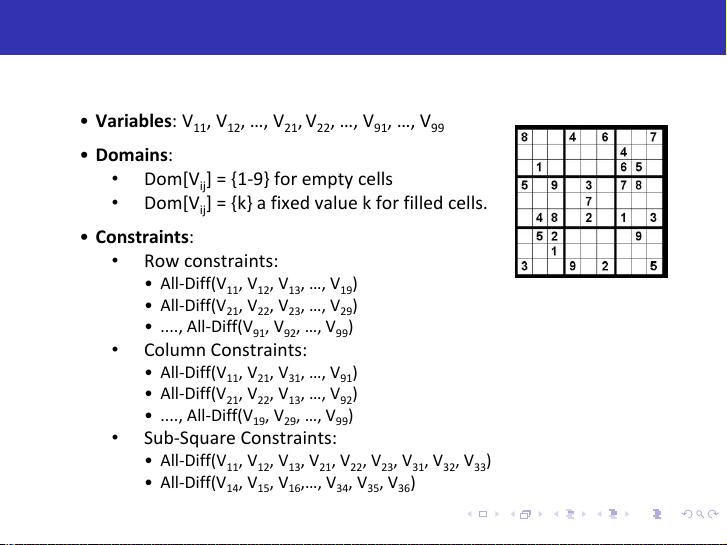
Constraint Satisfaction Problems (CSP) involve finding a solution that satisfies a set of constraints or conditions. These problems are formalized by defining a set of variables, a domain of possible values for each variable, and a set of constraints that specify the relationships between variables. Solving a CSP involves finding an assignment of values to variables that satisfies all the constraints.
There are several algorithms for solving CSPs, including the Backtracking algorithm, the Forward checking algorithm, and the Generalized Arc Consistency (GAC) algorithm. The Backtracking algorithm is a fundamental search algorithm that explores possible solutions by trying different values for each variable and backtracking when a constraint is violated. The Forward checking algorithm is an improvement on backtracking that prunes the search space by checking for inconsistencies as variables are assigned values. The GAC algorithm is a more advanced approach that enforces consistency by propagating constraints and removing values that are not possible.
These algorithms are based on the work of Sheila McIlraith and Y. Liu, and are designed to efficiently solve CSPs with a general state representation. By abstracting the problem into a set of variables, domains, and constraints, these algorithms can find solutions for a wide range of problems without needing to know the specific details of each problem.
In conclusion, Constraint Satisfaction Problems are a powerful tool for modeling and solving problems with complex constraints. The algorithms discussed in this lecture provide efficient ways to search for solutions that satisfy all constraints, making them an important part of artificial intelligence research and problem-solving techniques.
点击了解资源详情
点击了解资源详情
点击了解资源详情
2022-08-03 上传
2022-08-03 上传
2022-08-04 上传
2015-12-26 上传
2021-09-19 上传
2021-10-07 上传
傅融
- 粉丝: 31
- 资源: 333
最新资源
- 基于Python和Opencv的车牌识别系统实现
- 我的代码小部件库:统计、MySQL操作与树结构功能
- React初学者入门指南:快速构建并部署你的第一个应用
- Oddish:夜潜CSGO皮肤,智能爬虫技术解析
- 利用REST HaProxy实现haproxy.cfg配置的HTTP接口化
- LeetCode用例构造实践:CMake和GoogleTest的应用
- 快速搭建vulhub靶场:简化docker-compose与vulhub-master下载
- 天秤座术语表:glossariolibras项目安装与使用指南
- 从Vercel到Firebase的全栈Amazon克隆项目指南
- ANU PK大楼Studio 1的3D声效和Ambisonic技术体验
- C#实现的鼠标事件功能演示
- 掌握DP-10:LeetCode超级掉蛋与爆破气球
- C与SDL开发的游戏如何编译至WebAssembly平台
- CastorDOC开源应用程序:文档管理功能与Alfresco集成
- LeetCode用例构造与计算机科学基础:数据结构与设计模式
- 通过travis-nightly-builder实现自动化API与Rake任务构建