COL 12(3), 031405(2014) CHINESE OPTICS LETTERS March 10, 2014
Pulse dynamics controlled by saturable absorber in a
dispersion-managed normal dispersion Tm-doped
mode-locked fiber laser
Xiaoliang Yang (), Yu Chen ( ), Chujun Zhao ()
, and Han Zhang ( )
Key Laboratory for Micro-/Nano-Optoelectronic Devices of Ministry of Education, College of
Information Science and Engineering, Hunan University, Changsha 410082, China
∗
Corresponding author: cjzhao@hun.edu.cn
Received Nov ember 20, 2013; accepted January 15, 2014; posted online February 28, 2014
Based on the coupled Ginzburg-Landu equation, we numerically investigate the pulse dynamics in a
dispersion-managed normal disp ersion Tm-doped mode-locked fiber laser. The influences of the mod-
ulation depth and saturation power of saturable absorber on the pulse dynamics are presented. The
simulation results show that these parameters are crucial to achieve high pulse energy and high pulse peak
power pulsed laser near 2-µm wavelength.
OCIS codes: 140.3510, 320.7090, 190.4370.
doi: 10.3788/COL201412.031405.
High power, ultra-short fiber lasers operating near 2 μm
have attracted much attention because their wide range
of application in light detection and ranging (LIDAR),
optical communication, spectroscopy, and efficient at-
tainment of mid-infrared laser
[1−4]
. Among all the op-
tions, passive mode-locked Tm-doped fiber lasers oper-
ating in the range of 1800−2100 nm is one of the most
prevalent ones
[5−7]
. Moreover, energy scaling in mode-
locked fiber lasers depends strongly on the cavity disper-
sion management
[8−12]
, and in particular in the normal
dispersion regime
[13−15]
.
To construct a mode-locked fiber laser, saturable ab-
sorber plays a key role in stabilizing and shortening
mode-locked fiber laser. Therefore, how to select the
saturable absorber (SA) and control its parameters are
very important
[16,17]
. Semiconductor saturable absorber
mirrors (SESAMs) was considered as one of the most suc-
cessful passive mode-locker
[18]
. However, SESAMs have
relatively narrow operation bandwidth, usually a few tens
nm, and their parameters are not easy to control. With
the development of novel SAs, such as carbon nanotube
(CNT) and graphene, controlling the SA parameter be-
comes relatively easy
[19−21]
. For example, the modula-
tion depth and saturable power of graphene can just be
tuned by varying the thickness of graphene film
[22]
.Re-
cently, a novel saturable absorber with high modulation
depth, topological insulator, has been studied
[23]
.Itis
expected that these saturable absorbers will make a big
difference for laser performance compared with conven-
tional saturable absorber. Therefore, studying the SA
parameters in the fiber oscillator numerically may be
beneficial as well as it allows of investigating a wide range
of independent SA parameters, which would be difficult
to perform in experiments. In this letter, we numeri-
cally investigated the effect of saturation absorber pa-
rameters on the pulse dynamics of the normal dispersion
Tm-doped fiber laser. A dispersion managed normal dis-
persion cavity is modeled which is useful to generate high
power and ultra-short pulse output.
The proposed dispersion-managed normal dispersion
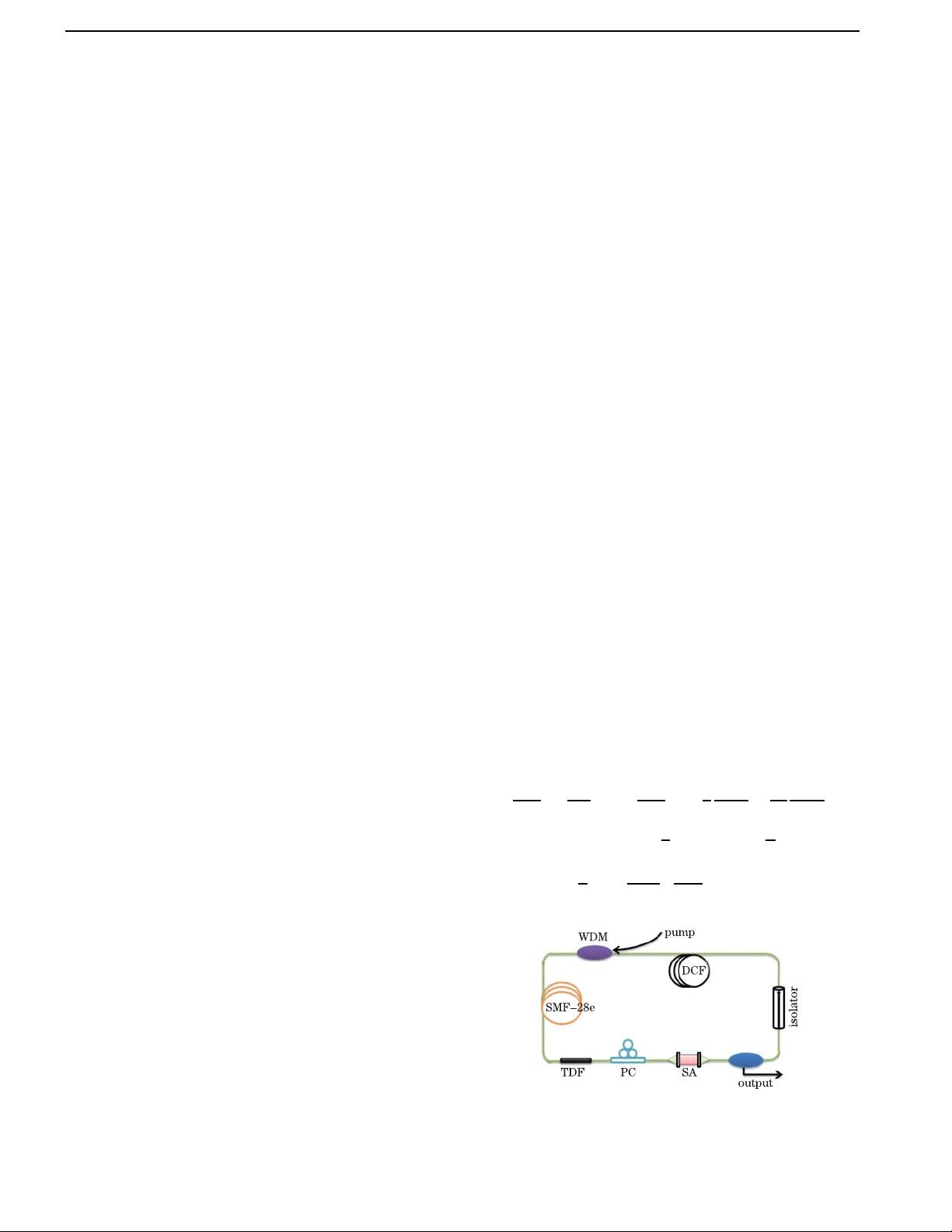
Tm-doped fiber laser is schematically shown in Fig. 1.
The oscillator is made up of a wavelength-division multi-
plexing (WDM) coupler, an isolator, a polarization con-
troller (PC), an output coupler (OC), a fast saturable
absorber (SA), and three segments of fiber. The total
length of the laser cavity is 6.9 m, including a 3-m-long
passive single-mode fiber (SMF-28e), a 1.0-m-Tm-doped
gain fiber. To make dispersion management at 2 μm
wavelength is not easy because standard single mode
fibers (SMF) at 1 and 1.5 μm exhibit large anomalous
dispersion at 2-μm. Here, a 2.9-m-long high numeri-
cal aperture SMF with large normal dispersion at 2 μm
used for dispersion compensation
[24,25]
. The unidirec-
tional propagation of intracavity pulse is controlled by a
polarization independent isolator.
The pulse propagation was modeled in the weakly bire-
fringence fibers based on asymmetrical spit-step Fourier
algorithm that solves the well-known coupled Ginzburg-
Landu equation
[26]
:
∂F
x
∂Z
=j
Δβ
2
F
x
− δ
∂F
x
∂T
− β
2
j
2
∂
2
F
x
∂T
2
+
β
3
6
∂
3
F
x
∂T
3
+ jγ
|F
x
|
2
+
2
3
|F
y
|
2
F
x
+ j
γ
3
F
∗
x
F
2
y
+
g
2
F
x
+
g
2Bw
·
∂F
2
x
∂T
2
, (1)
Fig. 1. Dispersion-managed normal dispersion Tm-doped
f iber laser. TDF: Tm-doped f iber; DCF: dispersion compen-
sation fiber.
1671-7694/2014/031405(4) 031405-1
c
2014 Chinese Optics Letters









