弹道目标跟踪综述：第二部分—运动模型详解
需积分: 21 151 浏览量
更新于2024-07-16
收藏 274KB PDF 举报
本文是一篇深入研究的目标追踪综述的第二部分，专注于弹道目标模型在机动目标跟踪中的应用。作者X.Rong Li 和 Vesselin P. Jilkov来自美国新奥尔良大学电气工程系，他们针对机动目标跟踪问题及其技术，特别关注了在排除所谓的测量起始不确定性的情况下，弹道目标运动模型的探讨。
标题"Survey of Maneuvering Target Tracking: II. Ballistic Target Models"明确指出，本研究旨在系统地梳理机动目标跟踪领域的挑战和技术，特别是当目标在飞行的不同阶段（助推、滑翔和再入）表现出复杂轨迹时，如何构建和应用适合的弹道模型。这些阶段包括：
1. **助推阶段**：在这个阶段，目标通常经历初始发射和推进阶段，需要考虑推力的影响、加速度变化以及可能的轨道修正。模型应能预测由于火箭引擎活动导致的高动态行为。
2. **滑翔阶段**：目标在大气层中自由飞行，受到重力和空气阻力的影响。模型必须考虑到空气动力学效应，如姿态控制、气动参数变化，以及可能的外部干扰。
3. **再入阶段**：当目标返回地球大气层并重新进入时，其轨迹会受到大气阻力、重力偏斜和可能的热防护系统的影响。模型需处理这些复杂的减速和不稳定过程。
文章的重点在于提供一个全面的方法论框架，以便工程师们在设计跟踪算法时，可以根据弹道目标的具体运动特性选择合适的数学模型，如惯性模型、多体动力学模型或者基于卡尔曼滤波的估计方法。此外，文中还可能涉及模型参数辨识、不确定性分析以及对抗机动策略等关键话题。
在撰写这份综述时，作者参考了国际光学科学与技术会议的论文，该会议于2001年在圣地亚哥召开。通过回顾和比较不同文献中的研究成果，作者为读者揭示了机动目标跟踪领域的最新进展和挑战，有助于学术界和业界专业人士更好地理解和应对机动目标的复杂动态行为。
这篇论文是机动目标追踪领域的重要参考资料，对于理解弹道目标模型在实际跟踪系统中的应用具有很高的价值。它不仅概述了理论基础，也包含了实践中的关键技术讨论，是从事相关研究或开发工作的理想起点。
3 Ballistic (Coast) Flight
After the thrust cut-off or burnout and leaving the atmosphere a BT enters the free-flight portion of its trajectory — no
thrust is applied and no drag is experienced. The motion may be considered governed by the gravity alone — other factors
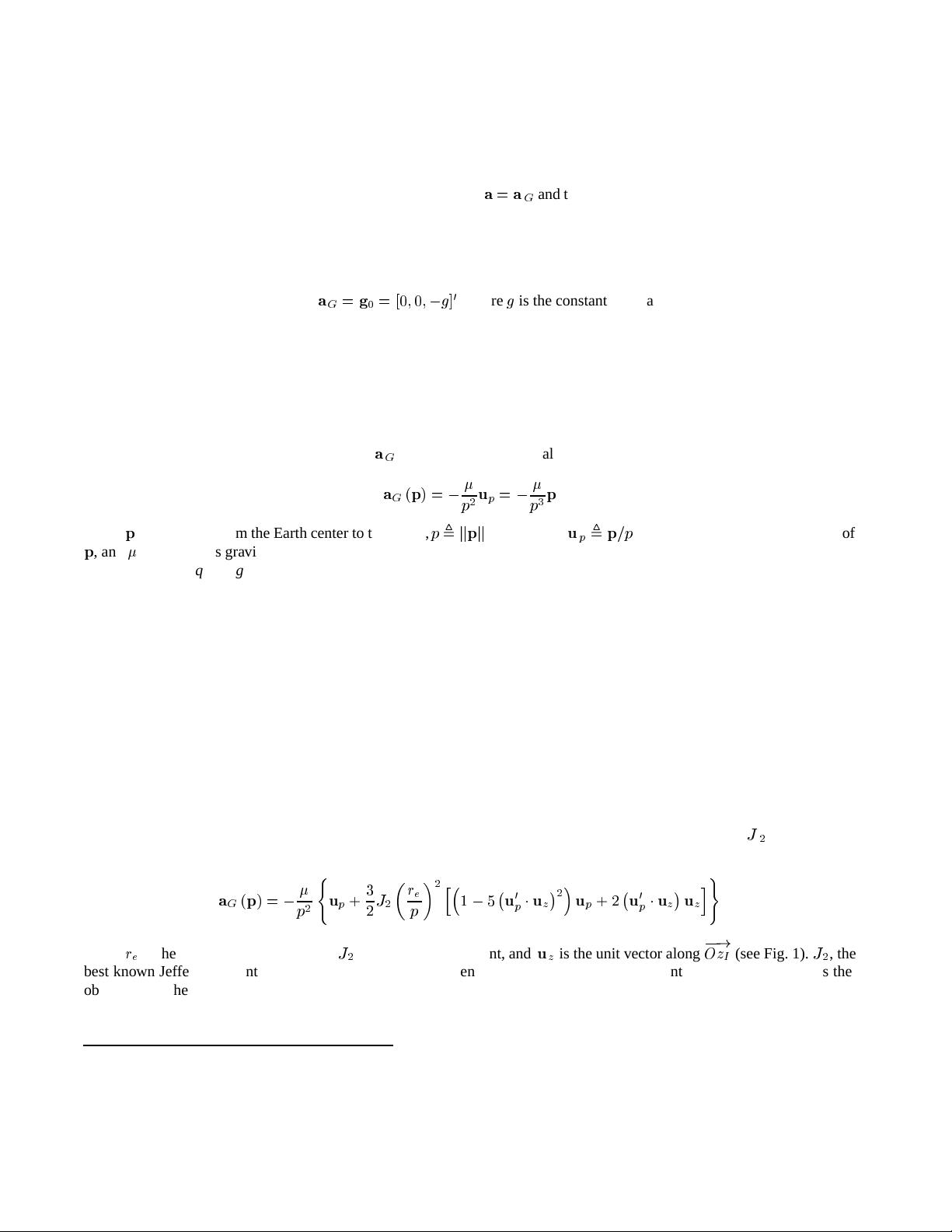
(e.g., perturbations) are neglected. By (2) the total acceleration is
a
=
a
G
and thus obtaininga coast model of the BT amounts
to selection of an appropriate gravity model.
3.1 Gravity Models
Flat Earth Model. The simplest possible model of gravity assumes a flat, nonrotating Earth. In this model, the gravity
acting on the target in the ENU-CS is
a
G
=
g
0
=[0
;
0
;
g
]
0
, where
g
is the constant gravitational acceleration of the Earth.
The boost and reentry portions of a BT trajectory are relatively short in range compared to the Earth radius and take place
in a close vicinity of the Earth. Thus a model of flat, non-rotating Earth may be adequate, particularly in view of the presence
of other more dominating uncertainties. On the contrary, the coast flight comprises much greater ranges usually and thus
accounting for the Earth sphericity (and even ellipticity) and rotation is essential.
Spherical Earth Model. Assume that the Earth and the BTs can be represented as point masses at their geocenters
5
and
that the gravitational forces of the moon (and other stars) can be neglected. Since the target has a negligible mass relative to
the Earth’s mass, the gravitational acceleration
a
G
is the solution of a so-called restricted two-body problem, obtained by the
Newton’s inverse-square gravity law [4] as
a
G
(
p
)=
p
2
u
p
=
p
3
p
(4)
where
p
is the vector from the Earth center to the target,
p
,
k
p
k
is its length,
u
p
,
p
=p
is the unit vector in the direction of
p
, and
is the Earth’s gravitational constant
6
.
This inverse-square gravitational acceleration model (4) is classical and has been commonly used in a variety of BT
tracking applications [12, 13, 14, 15, 16, 17, 18, 19, 9]. It is attractive for its simplicity. It has been proven satisfactory for
tracking of BTs over a short range and/or period, for example, as a model of the gravitational acceleration as an integral
part of the total acceleration within a boost (boost-to-coast) motion model [15, 16, 19, 9] or for track initiation purposes
[17]. However, its underlying assumptions are rather idealistic, especially the one that the Earth can be treated as a point
mass. This may make it inadequate for other BT tracking applications, in particular, precision tracking of coast targets over
a long time period or at a low data rate, such as the ballistic flight portion of a long-range missile or a satellite. Clearly,
these simplifying assumptions becomes less accurate when the targets being tracked travel over a larger geographical region
and a longer time period. Also, during the exo-atmospheric ballistic flight, gravity is either the only practical or at least the
dominating acceleration acting on the targets and thus needs to be modeled more accurately. That is why the employment of
more precise gravity models have been proposed.
Ellipsoidal Earth Model. More accurate expressions for the gravitational acceleration can be obtained by replacing the
above spherical Earth model with an ellipsoidal (or more precisely, spheroidal) Earth model. Such a more precise approx-
imation — accounting for the Earth oblateness by including the second-order gravitational harmonic term
J
2
of the Earth’s
gravitational field model — is [4, 11]
a
G
(
p
)=
p
2
(
u
p
+
3
2
J
2
r
e
p
2
h
1
5
u
0
p
u
z
2
u
p
+2
u
0
p
u
z
u
z
i
)
(5)
where
r
e
is the Earth’s equatorial radius,
J
2
is a correction constant, and
u
z
is the unit vector along
!
Oz
I
(see Fig. 1).
J
2
, the
best known Jeffery constant, represents the difference between the polar and equatorial moment of inertia. It quantifies the
oblateness of the Earth because it is approximately equal to one third of the ellipticity of the Earth, hence also known as the
oblateness term. This model is usually considered sufficiently accurate for most BT tracking applications [11], at a cost of
considerable nonlinearity.
5
This holds if the Earth and the targets are spherically symmetric with an even distribution of their masses.
6
For the values of well-known constants used in this survey, the reader is referred to [4].
Proc. SPIE Vol. 4473
562
Downloaded From: https://www.spiedigitallibrary.org/conference-proceedings-of-spie on 24 Nov 2019
Terms of Use: https://www.spiedigitallibrary.org/terms-of-use
剩余23页未读，继续阅读
2013-07-23 上传
2022-07-15 上传
2019-08-19 上传
2021-02-08 上传
2021-02-07 上传
2021-02-10 上传
2021-02-08 上传
2019-12-12 上传
2022-07-15 上传
wyhbill_xjy
- 粉丝: 0
- 资源: 1
我的内容管理
展开
最新资源
- 黑板风格计算机毕业答辩PPT模板下载
- CodeSandbox实现ListView快速创建指南
- Node.js脚本实现WXR文件到Postgres数据库帖子导入
- 清新简约创意三角毕业论文答辩PPT模板
- DISCORD-JS-CRUD：提升 Discord 机器人开发体验
- Node.js v4.3.2版本Linux ARM64平台运行时环境发布
- SQLight：C++11编写的轻量级MySQL客户端
- 计算机专业毕业论文答辩PPT模板
- Wireshark网络抓包工具的使用与数据包解析
- Wild Match Map: JavaScript中实现通配符映射与事件绑定
- 毕业答辩利器：蝶恋花毕业设计PPT模板
- Node.js深度解析：高性能Web服务器与实时应用构建
- 掌握深度图技术：游戏开发中的绚丽应用案例
- Dart语言的HTTP扩展包功能详解
- MoonMaker: 投资组合加固神器，助力$GME投资者登月
- 计算机毕业设计答辩PPT模板下载
