The centre of the Earth o is the origin. The y-axis is in the
direction of the tangent velocity, and the x-axis is perpen-
dicular to the y-axis and points toward the object from
the Earth’s centre. The z-axis is defined according to the
right-hand rule.
2.1.3. Object-fixed coordinate system
The object-fixed coordinate system is the coordinate sys-
tem that is rigidly connected to the object. It is often uti-
lised to describe inherent feat ures of the object. The
analysis of the changes in the image plane must be per-
formed in the object-fixed coordinate system. In the initial
positioning orientation, we assume that the reference-orbit
coordinate system coincides with the object-fixed coordi-
nate system. In the absolute orientation, the object-fixed
coordinate system can be regarded as identical to the
satellite-based coordinate system based on the assumptions
that the orbital eccentricity is zero and neglecting the effects
of Earth’s rotation.
2.1.4. Reference radar coordinate system
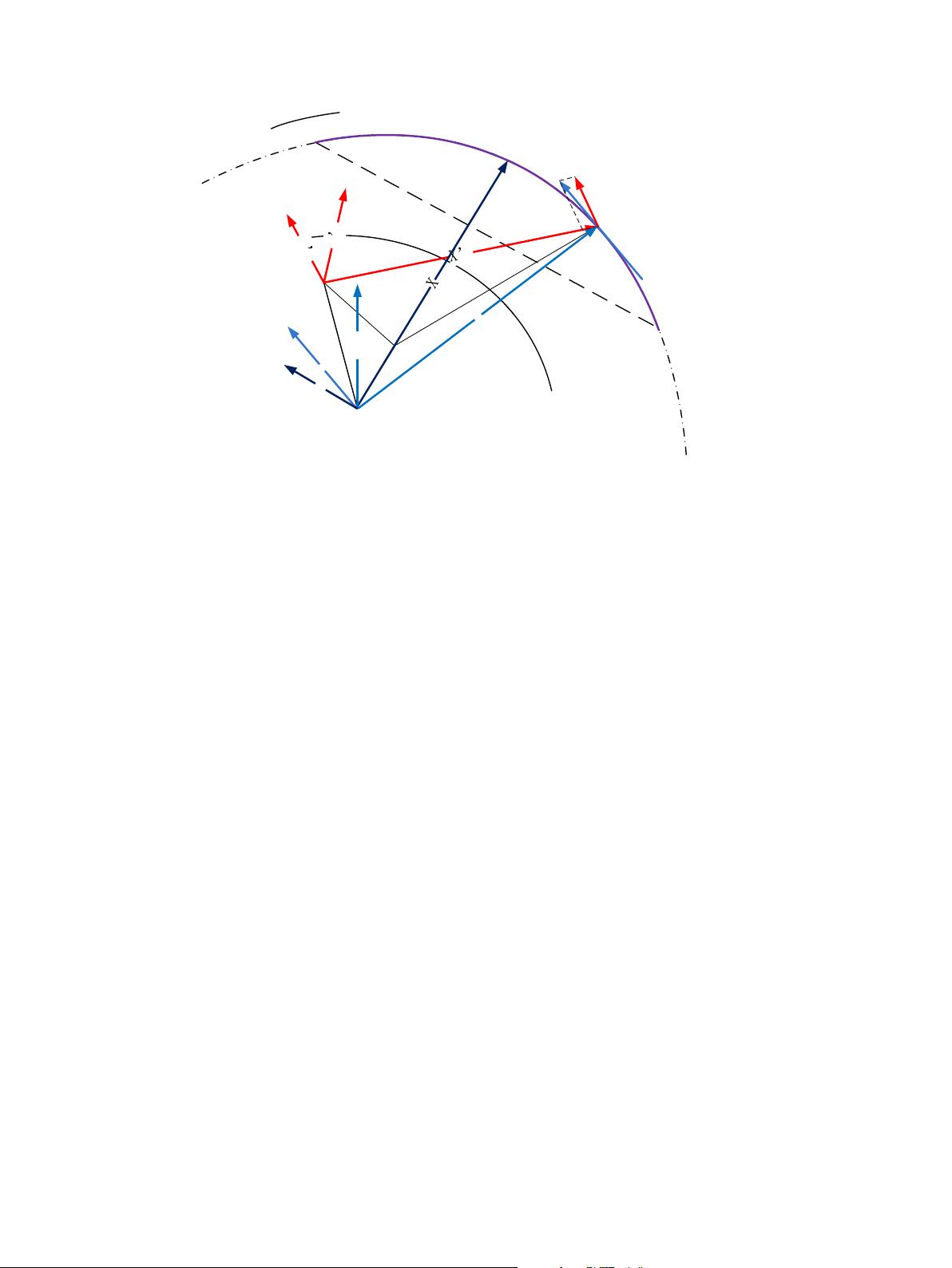
The reference radar coordinate system in Fig. 2, one
coordinate direction X
0
of which always coincides with
the LOS and another coordinate Y
0
of which always lies
in the plane spanned by the changing LOS, is marked in
red lines. The rotation axis Z
0
is then given by the cross pro-
duct of two LOS directions at tw o distinct time instances.
2.2. Definition of observation geometry
During the flight of many satellites, the three perpendic-
ular axes of the satellite must be controlled simultaneo usly
to prevent any axis from exceeding specified values for
rotation and swing. This method of stabilisation is called
three-axis attitude-stabilisation for satellites. Presently,
almost all satellites in LEO adopt the three-axis attitude-
stabilisation approach for attitude control because it is
applicable to satellites with multiple types of orbits with
various orientation requirements. This approach can also
be applied to other satellite processes, such as rendezvous,
docking, return and orbital transformation.
This study analyses two main three-axis attitude-
stabilisation methods for LEO space objects, which are
shown in Fig. 3. One stabilisation method keeps the axes
in the object-fixed coordinate system parallel to fixed vec-
tors in the inertial system; therefore, this method is called
inertial positioning, as shown in Fig. 3(a). The other stabil-
isation method called absolute orientation maintains one of
the axes in the object-fixed coordinate system pointing at
the centre of the earth, as shown in Fig. 3(b).
The geometric model for LEO space object ISAR
imaging based on these two attitudes (Katayama et al.,
1992; Ali et al., 1998), assuming that the orbital eccentricity
is zero and neglecting the effects of Earth’s rotation, is
shown in Fig. 4.
In Fig. 4, A is the position of the radar, O is the Earth’s
centre, R
e
is the radius of the Earth, and R
A
is the distance
between the radar station and the centre of the Earth. The
height of the object’s orbit is H , and R is the distance
between the object and the centre of the Earth. The horizon
of the radar station and the object’s orbit intersect in the
chord PQ. The orbit drawn in the solid red line is the vis-
ible orbit section when it ascends above the horizon of the
radar station. D is the apse of the visible orbit section, n is
half of the central angle of the visible orbit, and hðtÞ is the
central angle of the object’s apse. If E is set as the projec-
tion of the radar station in the orbit plane, the distance
between the radar station and the orbit plane is AE ¼ h.
Radar A
O center of the Earth
great circle of Earth’s
orbit plane
satellite’s orbit
D
E
T
Z(z)
Y
Z
y
Y
Satellite-based
coordinate system
Reference Orbit
coordinate system
Radar reference
coordinate system
Fig. 2. Schematic of the coordination systems.
32 J. Chen et al. / Advances in Space Research 58 (2016) 30–44









