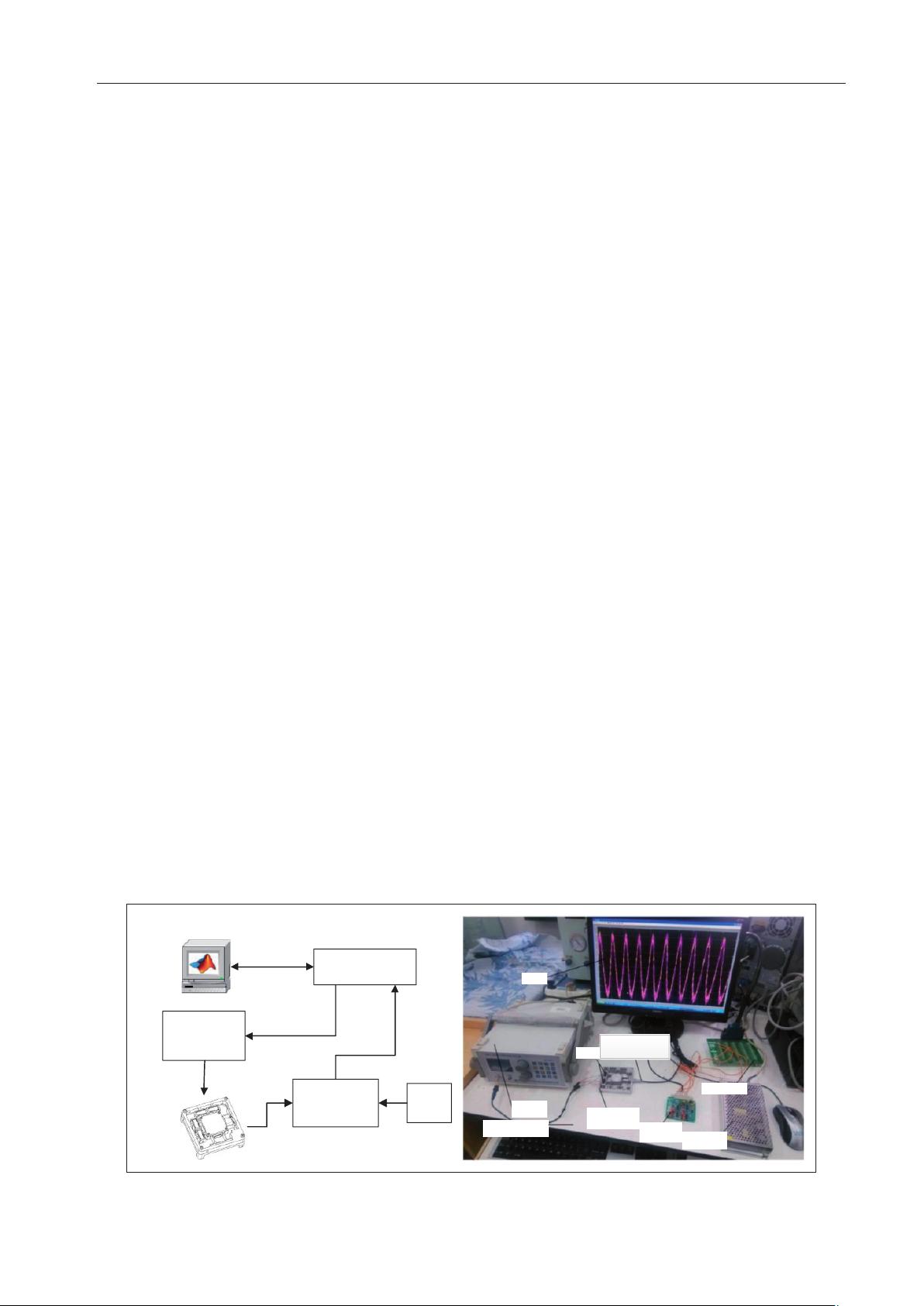
Experimental setup
The control flow and experimental setup is graphically
shown in Figure 2. According to the consideration of
the large-workspace requirement, the driver power
supply (HPV-1C0300A0300 from Suzhou MAT,
Inc.) and four PZTs (SZBS150/5 5/20 with open-
loop travel of 20 mm from Suzhou MAT, Inc.) are
selected to drive the microposition stage. To accur-
ately obtain the output displacement of the platform
centric at two axes, two high-resolution capacitance
displacement sensors (MA-0.5, from BJZD, Inc.) and
a data acquisition card (PCI-6221, from NI, Inc.) are
adopted in the research. To minimize the external
interference, all the units are mounted onto a high-
performance vibration isolation table.
EUPI hysteresis compensator
The hysteresis phenomenon of piezoactuated
microposition stage
First, a periodic sine signal with time-varying amplitude
and a constant rate (u ¼ absðcosð0:1tÞ1Þe
0:004t
)
and a triangular wave (u ¼ absðsawtoothð0:04t,
0:5Þ1Þe
0:004tðÞ
)areappliedtothePZTsbytheamp-
lifier power supply (HPV-1C0300A0300). Acquiring the
input signal and the output displacement with the fixed
sampling time (0.001 s) by the experimental setup, the
related results are shown in Figure 3. The hysteresis
nonlinearity of this system is obvious (the maximum is
approximately 21.8%). Moreover, the microposition
stage cannot easily satisfy the cell injection and IC man-
ufacturing precision requirements with large nonlinear
characteri stics.
Before the design of the controller, the dynamics of
the microposition stage are studied. In general, a
Hammerstein-like model can describe the dynamic
behavior of the stage.
32–34
Figure 4 schematically
shows the Hammerstein-like model, where H
represents the hysteresis nonlinearity, and G repre-
sents the stage linear model.
EUPI model and compensator
The inherent hysteresis effect of the PEAs will intro-
duce nonlinearity into the microposition system;
this nonlinearity should be eliminated to guarantee
the precision positioning accuracy. In recent years,
many research groups have focused on compensating
the hysteresis nonlinearity by depending on the
inverted model that will closely describe the hysteresis
behavior. In the above models, the EUPI method is a
new hysteresis compensation algorithm and is derived
from the PI model, which can be found in our recent
study.
15
The hysteresis operator mathematic form of EUPI
can be described as follows
F
r,
j
½uðtÞ¼max fuðtÞr, minf
j
uðtÞþrðÞ, F
r,
j
ðt
Þgg
j
4 0, r50 t
¼ lim
!0
ðt Þ
ð1Þ
where F
r,
j
½uðtÞ is the hysteresis operator output; u(t)
denotes the drive signal at time t; r is the dead zone,
and
j
is the jth angle assigned to tilt the descending
edge, including
j
5 1,
j
¼ 1 and
j
5 1.
And three parts of the complex hysteresis nonlinear-
ity effect can be considered, i.e. symmetric hysteresis,
asymmetric hysteresis, and memory-less polynomial.
The model is expressed as follows
H½uðtÞ¼
CPI
½uðtÞþ
UPI
½uðtÞþP½uðtÞð2Þ
where
CPI
u½ðtÞ and
UPI
½uðtÞ are the symmetric hys-
teresis part and the asymmetric hysteresis part; P[u](k)
PC
PCI 6221
DAQ Card
PZT Driving
Power
Capacitance
displacement
sensors
Micro
position
stage
24V
Power
Driver
Power
PZT
Micro
position stage
Capacitance sensors
NI PCI6221
24V DC
Power
Condition
circuit
PC
Vibration isolation
table
Figure 2. The schematic diagram of the experimental setup.
Hao et al. 2869









