COL 11(6), 061201(2013) CHINESE OPTICS LETTERS June 10, 2013
Polarimetric interferometer for measuring nonlinearity error
of heterodyne interferometric displacement system
Su’an Xu (
NNN
SSS
)
1∗
, Luc Chassagne
2
, Suat Topcu
2
, Le Chen (
WWW
)
1
,
Jian Sun (
jjj
)
1
, and Tianhong Yan (
îîî
UUU
÷÷÷
)
1
1
College of Mechanical and Electrical Engineering, China Jiliang University, Hangzhou 310018, China
2
LISV, University of Versailles Saint-Quentin, Versailles Cedex, 78035, France
∗
Corresponding author:zjxusuan@126.com
Received November 14, 2012; accepted February 25, 2013; posted online May 30, 2013
This letter presents a polarimetric interferometer (PI) that can measure the ellipsometric parameter θ with
an accuracy of 0.01
◦
leading to a potential accuracy of 17 pm. The PI is constructed and compared with
a commercial heterodyne interferometer (HI). Given its low nonlinearity, the PI is used to measure the
residual nonlinearity of a heterodyne interferometric displacement system. A rotating half-wave plate is
used to compensate for a part of the nonlinearity error caused by the misalignment of the axis between
input polarizing states and beamsplitter.
OCIS codes: 120.2130, 060.4370, 060.2840, 000.3110.
doi: 10.3788/COL201311.061201.
The production of semiconductors, photolithography
steppers, and metro logical instruments rely o n interfer-
ometric displacement systems to improve po sition accu-
racy. Reduced minimum feature size and increased dis-
placement range in industry impo se more stringent per-
formance requirements for interferometric systems. Con-
sidering its high signal-to-noise ratio (SNR) and easy
alignment, heterodyne interferometers (HIs) are widely
used in precision length measurement and positioning
systems. However, the accuracy of HIs is limited by
their intrinsic nonlinearity error. The methods for mea-
suring or reducing the nonlinearity error of displacement
measurements by HIs have been studied by numerous
researchers
[1−12]
. Wu et al.
[1−3]
constructed detailed
mathematical models of nonlinearity errors in HIs. Lo-
rier et al.
[4]
developed two independent methods for mea-
suring the polarization state properties o f laser beams
to determine the non-linearity error in HIs. Wu et
al.
[5−7]
, developed spec ific interferometers without fre-
quency mixing. In 2006, Hou
[8]
found that the nonlin-
earity of HIs could b e subdivided by the multiple of the
optical path. Her research group
[9]
developed a simple
technique for eliminating the no nlinearity of a HI using
a simple phase compensatio n system with a rotated po-
larizer before the detector. Patterson et al.
[10]
exp eri-
mentally demonstrated that the nonlinearity error could
be reduced by subtracting a small amount of the refer-
ence signal from the meas urement signal. Eom et al.
[11]
developed a phase-encoding electronics capable of com-
pens ating for the nonlinearity error in HIs.
In this letter, a polarimetric interferometer (PI) capa-
ble of interpolating the optical fringe into 1/36 000 using
polarization state measurement is proposed. The pro-
posed P I is constructed and compared with a commer-
cial HI by measuring the displacement of the same target
mirror. The latter is displac e d stepwise with a step value
of 20 nm using a high-accuracy position control method.
The PI is calibrated with a commercial capacitive se n-
sor equipped with a piezoelectric (PZT) nano-positioning
system, and its nonlinearity is approximately 0.5 nm.
Given its low nonlinearity, the proposed PI is considered
as a reference for detecting the residual nonlinearity of
a heterodyne interferometric displacement system. We
also show that a ro tating half-wave plate can be used to
compensate for a part of the nonlinearity error caused
by the misalignment of the axis between input polarizing
states and beamsplitter by monitoring the intensities of
the residual optical beat signal in the HI. Only one cycle
with re sidual nonlinearity error of approximately 3.5 nm
in the heter odyne interferometric displacement system is
achieved.
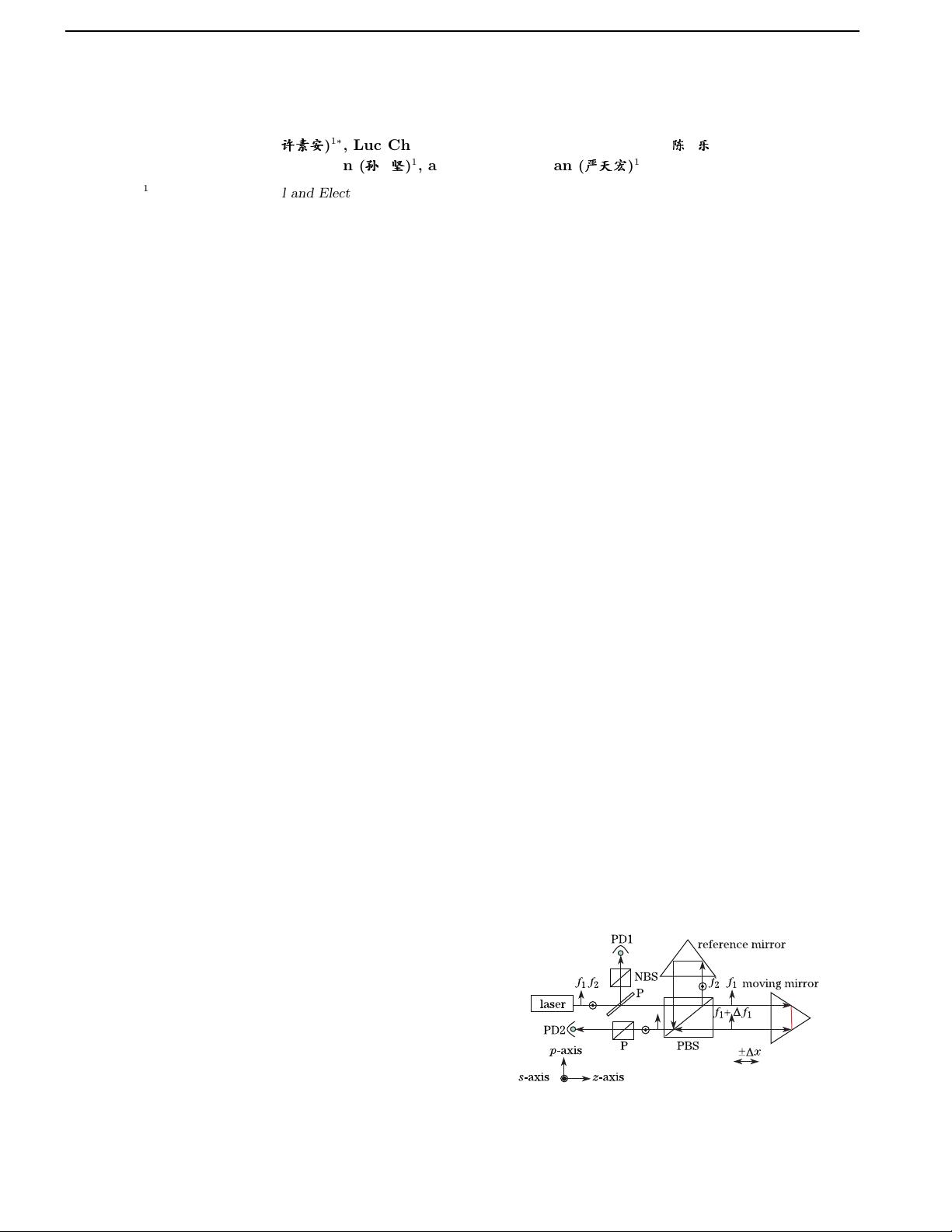
An ideal HI with two orthogonal linearly polarized laser
beams and different frequencies (f
1
, f
2
) is illustrated in
Fig. 1. The waves are supposed to propagate along the
positive direction of the z-axis of an s-p-z orthogonal co-
ordinate sys tem. The electric field components of the
laser beam are as follows. The initial phase is omitted
here to simplify the calculation.
E
s
= A exp[−i(2πf
2
t)]
E
p
= B exp[−i(2πf
1
t)]
, (1)
where A and B are the a mplitudes. According to the
principle of the HI, the intensity of the reference signal
from the photodetector PD1 is
I
ref
∝ AB cos(2π∆ft), (2)
Fig. 1. Principle of the HI. P:polarizer; PBS:polarizing beam-
splitter; PD:photodetector.
1671-7694/2013/061201(5) 061201-1
c
2013 Chinese Optics Letters





































