3592 IEEE TRANSACTIONS ON WIRELESS COMMUNICATIONS, VOL. 9, NO. 11, NOVEMBER 2010
same pilot sequence. We obtain simple expressions for the
signal-to-interference ratio (SIR) which are random due to
their dependence on the slow fading, and which are equal for
all OFDM tones. We numerically determine the cumulative
distribution functions of the forward- and reverse-SIRs. We
translate the SIR expressions into capacities by assuming that
Gaussian signaling is employed, and by treating the inter-
cellular interference as noise. We are interested in the mean-
throughput per cell, the number o f terminals which can be
advantageously served in each cell, the mean-throughput per
terminal, and the .95-likely throughput per terminal. Our quan-
titative results depend on only a few modeling parameters: the
log-normal shadow fading standard deviation, the geometric
attenuation exponent, and the ratio of the radius of the disk
from which terminals are excluded to the radius of the cell.
In addition to the nu merically-derived conc lusions, we
obtain several mathematically-exact conclusions (subject, of
course, to the limitations of the model): the throughput per
cell and the number of terminals per cell are independent of
the size of the cell, the spectral efficiency is independent of
the bandwidth, and the required transmitted energy per bit
vanishes.
Several approximate conclusions hold: the optimum number
of terminals to serve (from the standpoint of maximizing the
mean throughput) is equal to one-half of the duration of the
coherence interval divided by the delay-spread, the throughput
per terminal is independent of the coherence time, the effect
of doubling the coherence time is to permit twice as many
terminals to be serviced, and the reverse-link performance is
nearly identical to the forward-link performance, although the
statistics of the SIRs are slightly different.
For a scenario in which the coherence time is 500 mi-
croseconds (which could accommodate TGV - Train
`
a Grande
Vitesse - speeds), a delay spread of 4.8 microseconds, and a
bandwidth of 20 megahertz, with frequency re-use o f seven
the forward link h as the following performance: each cell can
serve 42 terminals, the mean net throughput per terminal is
17 megabits/sec, the 95%-likely net throughput per terminal
is 3.6 megabits/sec, and the mean net throughput per cell is
730 megabits/sec (equivalent to a spectral efficiency of 36.5
bits/sec/Hz). More aggressive frequency reuse (factors of three
or one) increase the mean-throughput, but decrease the 95%-
likely throughput.
D. Outline of Paper
Section II describes the mu lti-cell scen ario and propagation
model. Section III discusses the reverse-link pilots. Sections
IV and V analyze the multiuser reverse and forward data
transmission as the number of base station antennas becomes
infinite. The only remaining impairment is inter-cell interfer-
ence due to pilot contamination. The multi-cell analysis is par-
ticularly simple, and certain parameters, including the absolute
transmit powers and the absolute size of the cells, disappear
from the formulation. This analysis produces closed-form
expressions for effective signal-to-interference ratios (SIRs)
which depend only on the random positions of the terminals
and shadow-fading coefficients. In turn the SIRs translate
directly in to capacity expressions. Section VI numerically
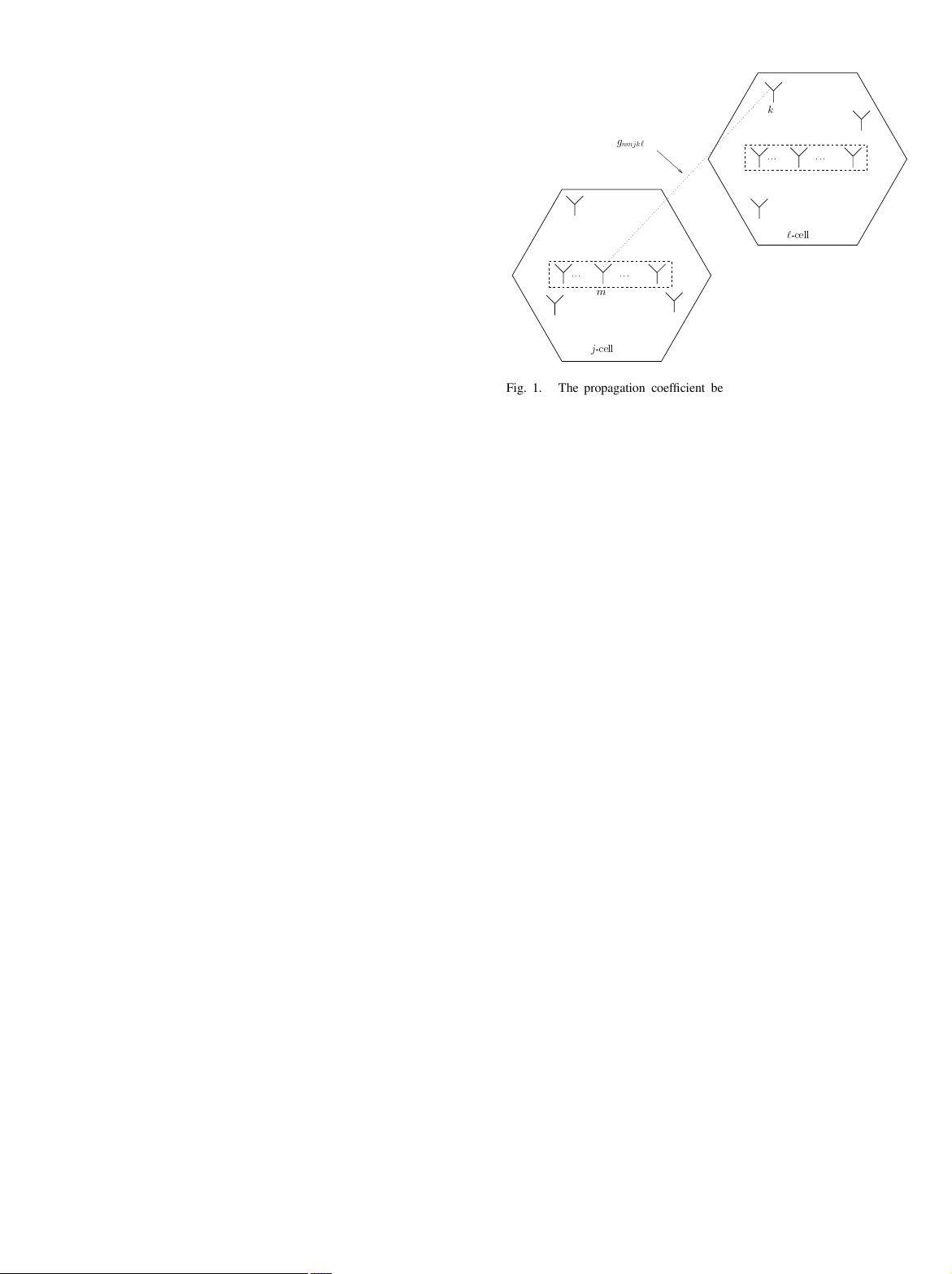
Fig. 1. The propagation coefficient between the 𝑘-th terminal in the ℓ-th
cell, and the 𝑚-th base station antenna of the 𝑗-th cell, in the 𝑛-th subcarrier,
is denoted by 𝑔
𝑛𝑚𝑗𝑘ℓ
.
obtains cumulative distribution functions for the SIRs and
capacities for a particular scenario. Sectio n VII discusses the
ramifications of our results.
II. S
CENARIO
Our scenario entails a hexagonal cellular geometry, base
stations having an unlimited number of antennas, terminals
having single antennas, OFDM, time-division duplex (TDD)
operation, and fast fading upon which is superimposed geo-
metric attenuation and log-normal shadow fading.
A. Hexagonal cells
The cells are hexagonal with a radius (from center to vertex)
of 𝑟
c
. Within each cell, 𝐾 terminals are placed randomly,
uniformly distributed over the cell, with the exclusion of a
central disk of radius 𝑟
h
. At the center of the cell is a base
station array comprising 𝑀 omnidirectional antennas, where
in the subsequent analysis, 𝑀 grows without limit.
B. OFDM
We assume that OFDM is utilized. We d enote the OFDM
symbol interval by 𝑇
s
, the subcarrier spacing by Δ𝑓 ,the
useful symbol duration by 𝑇
u
=1/Δ𝑓, and the guard interval
(duration of the cyclic prefix) by 𝑇
g
= 𝑇
s
− 𝑇
u
. We call the
reciprocal of the guard interval, when measured in subcarrier
spacings, the “frequency smoothness interval”,
𝑁
smooth
=1/(𝑇
g
Δ𝑓). (1)
C. Propagation
For our subsequent analysis we need to describe the prop-
agation coefficient between a single-antenna terminal in one
cell, and a base station antenna in another cell. Because of
TDD operation and reciprocity the propagation is the same
for either a downlink or an uplink transmission .
As shown in Fig. 1, we denote the complex propagation
coefficient between the 𝑚-th base station antenna in the 𝑗-th









