利用Bc→D(*)衰变测定|Vub|：竞争力提取与Lepton flavor universality测试

35 浏览量更新于2024-07-16 收藏 1.34MB PDF 举报

本文主要探讨的是利用即将到来的大型强子对撞机（LHC）升级II的潜力，对Bc介子的半 leptonic 衰变过程Bc→D(*)lν进行测量，其中l代表轻子，ν表示中微子。作者关注的是Bc→D(*)μν衰变，这是一种关键的途径来精确测量 CKM 矩阵中的|Vub|元素，这是由 Cabibbo-Kobayashi-Maskawa (CKM) 纠正因子所决定的，它反映了夸克之间的弱相互作用。通过计算得到的Bc→D(*)形状因数，作者提出了一种方法，即通过比较Bc→D(*)μν与Bc→J/ψμν这两种衰变模式，来降低实验和理论上的不确定因素，从而实现对|Vub|值的竞争性提取。Bc→J/ψμν衰变是相对较为稳定的参考点，其衰变特性已为人熟知，这种对比有助于提高精度。此外，文章还提及了一个理论上的兴趣点，即在零 recoil 限制下，通过比较Bc→D0eνe和Bc→Bseνe这两种衰变率的比值来间接估计|Vub|/|Vcs|的比值。虽然这一方法具有潜在的洞察力，但它目前尚处于理论探讨阶段，实际应用可能受到更复杂的理论计算和实验条件的限制。根据当前平均的|Vub|值，作者预测了Bc→D0μν和Bc→D*μν的分支比分别为(2.4±0.4)×10^-5和一个相关数值，这表明实验结果对|Vub|的测量具有重要的指导意义。这篇论文不仅关注实验技术的进步，还深入讨论了理论模型的应用，对于理解和提升对基本粒子物理参数的理解具有重要意义。

JHEP02(2020)171

(a) (b) (c) (d)

Figure 1. Contributions to correlation function from left to right: (a) perturbative diagram, (b)

nonlocal quark condensate diagram, (c) an example of a quark-gluon condensate diagram, (d) an

example of a gluon condensate diagram.

with the model-dependent function [30]

f(ν) =

a−2

Γ(a − 2)

1−a

−λ/ν

, a − 3 =

4λ

(2.14)

or in the simpler version [28, 29], which we use in this paper

f(ν) = δ



ν −



. (2.15)

The ﬁrst two moments of the model function f(ν) are ﬁxed by the OPE as

∞

dν f(ν) = 1,

∞

dν ν f(ν) =

(2.16)

Here m

is the standard OPE parameter [31–33] connected with the average quark virtu-

ality, and is deﬁned as a ratio of quark and quark-gluon condensates

gh¯q(x)(σ·G)q(0)i ≈ m

h¯q(x)q(0)i. (2.17)

We have checked explicitly that the use of the more sophisticated function f(ν) given

in (2.14) does not change anything in the conclusion. Namely, numerically the non-local

quark condensates Π

(3)

= Π

h¯qqi

and the mixed quark-condensate Π

(5)

= Π

h¯qσ.Gqi

are con-

tributing just up to 1% to the result. The analytic expressions are given in appendix A.2

and A.3, respectively.

The gluon condensate contributions Π

(4)

= Π

hGGi

to B

→ D

(∗)

form factors are

calculated from diagrams in ﬁgure 1d. The procedure is well known and the expressions

are very lengthy and cumbersome and similar to those already published in the literature

for B

→ J/ψ, η

transitions [34] and will not be given explicitly here. Some subtleties of

the calculation are given in appendix A.3. Numerically the gluon condensate contributions

do not exceed O(1%) in determined window of Borel parameters, but somewhat stabilize

the sum rules at smaller values of these parameters.

We deduce that all non-perturbative parts are numerically negligible, and can be safely

neglected. The main contribution to the correlation function (2.11) comes from the per-

turbative parts, eqs. (A.3)–(A.5).

– 7 –

JHEP02(2020)171

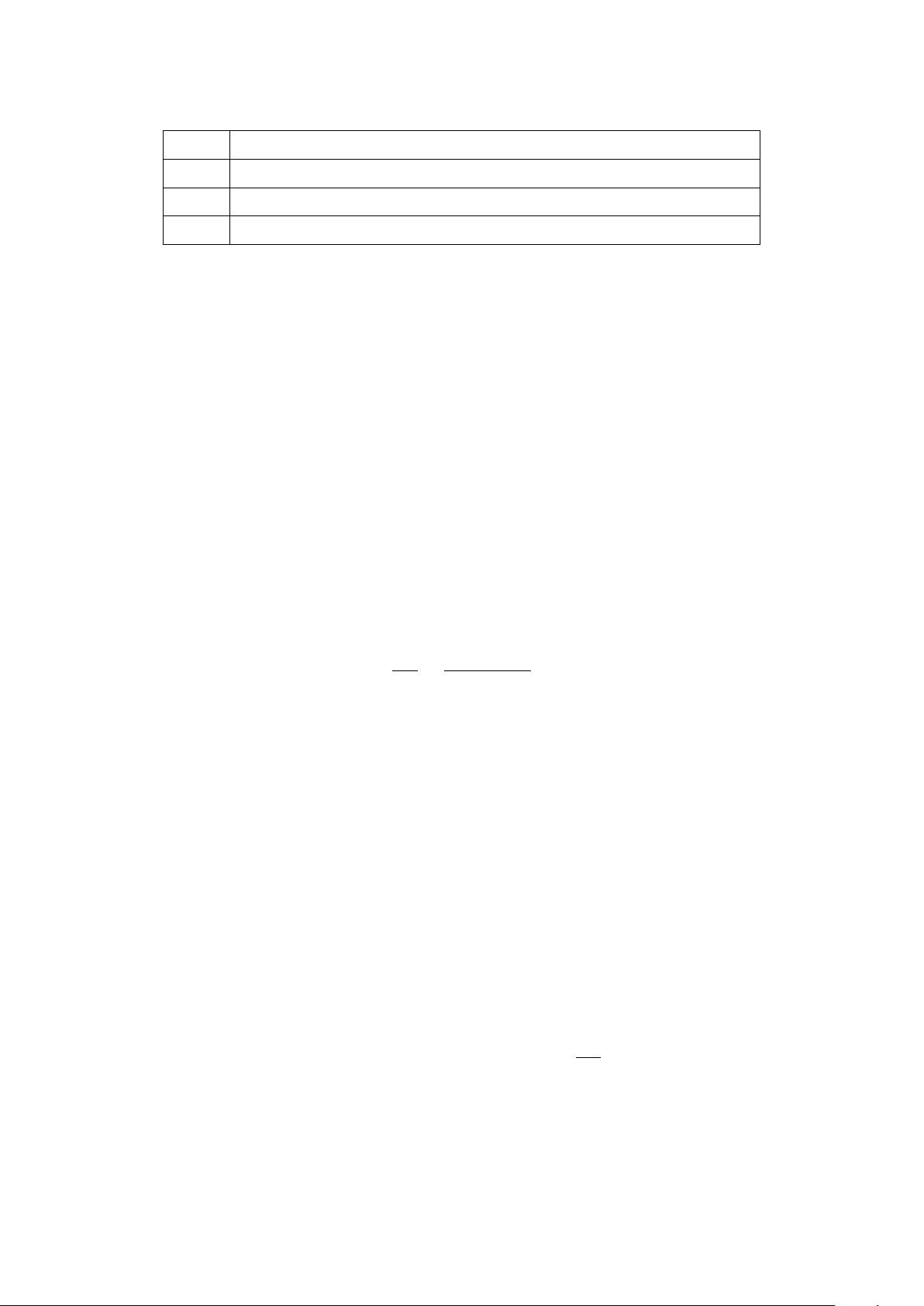
Meson lattice [MeV] our value [MeV] s

eﬀ

[GeV

] M

[GeV

]

427±8 [36], 434±5 [37] 425±25 53–55 30–50

213±2 [38], 207±4 [39] 212±16 7–7.5 4–6

∗

278±23 [40], 224±9 [41] 258±40 6–8 6–8

Table 1. Decay constants of mesons with 3ptSR parameters.

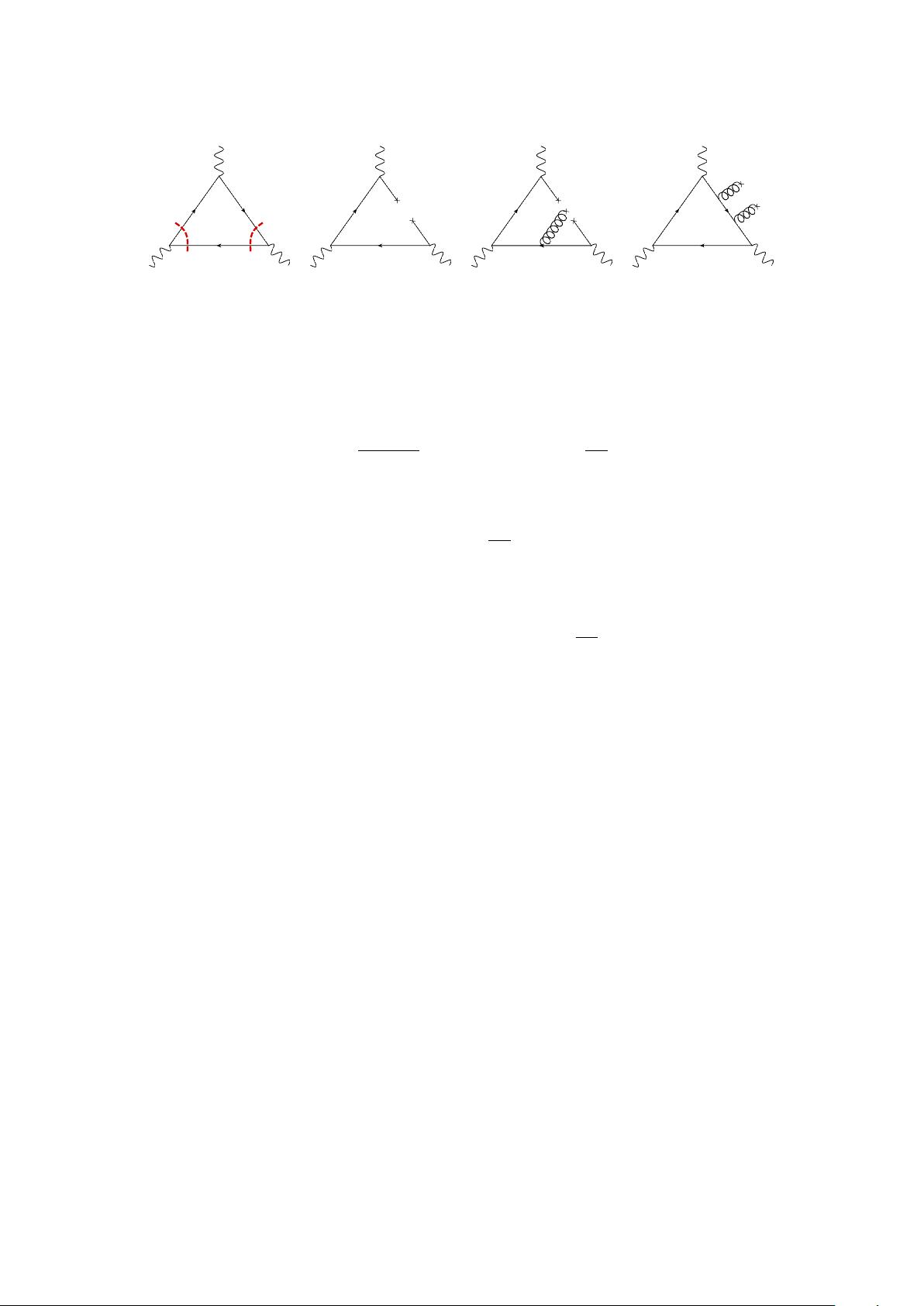
To calculate the form factors in QCD sum rules, in which the correlation function is

written as a sum of perturbative and non-perturbative contributions as in eq. (2.11), the

perturbative part is calculated by the usual expansion in the coupling constant, while the

non-perturbative part is described by the manner of Wilson’s operator product expansion

as a sum of expectation values of operators of increasing dimension. Since it is known that

when using the Borel-transformed sum rules in calculating heavy meson decay constants

higher orders of perturbation series can contribute as much as 30–40%, depending on the

scheme (heavy-light decay constants are known to NNLO [35]), whereas the QCD 3-point

function is only known to LO, here we parametrize the 3-point function with the same

threshold parameters s

eﬀ

that, at LO in QCDSR reproduce the meson decay constants

obtained from the lattice QCD calculations, listed in table 1, whereas the 3ptSR Borel

mass parameters M

are taken in the region where the stability is achieved in the sense of

appearance of the so called Borel plateau. An approximate relation connecting the Borel

mass parameters of diﬀerent meson decay constants noticed by authors in [42]

≈

− m

(2.18)

where m

is mass of the meson, and m

is the mass of its heavier quark, is found to

hold here too, and, as will be shown later, in 3-point calculations as well. Note however,

that the uncertainties of decay constants arising in our calculation are connected with our

speciﬁc method of calculation, since the threshold parameters are actually ﬁxed so that

they reproduce the lattice values, along with their uncertainties. Venturing into the 3-point

calculation, as mentioned above, we use the same Borel thresholds paired with the decay

constants that are reproduced by them. It is important to notice that, when estimating

uncertainties in the parameters of the 3ptSR calculation we do not vary the decay constants

and thresholds independently, but rather we always use decay constants values together

with the corresponding thresholds ﬁxed by the decay constants calculation. The hope is

that all the higher order/higher dimension operator contributions are reproduced through

the threshold modiﬁcation in the 3-point calculation as well. Otherwise, for the b quark

we use the so called “potential subtracted” mass [43], which is coincidentally very close to

both the Υ(1S) scheme mass [44] and the kinetic scheme mass [45, 46], whereas the c-quark

the mass is then given by varying the ratio Z of the two masses given by the QCD lattice

calculation [47, 48], keeping in mind that we do not use the MS masses, and this ratio for

pole masses tends to be lower as higher order corrections are included — which is why we

choose to use a somewhat lower value of Z. The same method described above was already

– 8 –

剩余44页未读，继续阅读

weixin_38550812

粉丝: 3
资源: 894

利用Bc→D(*)衰变测定|Vub|：竞争力提取与Lepton flavor universality测试

Bs→Kℓν和Bs→Dsℓν半瘦子衰变和| Vub / Vcb |的形状因子比率

$$ | V_ {cb} | $$ | Vcb |的确定 和$$ | V_ {ub} | $$ | Vub | 来自重音$$ \ Lambda _b $$Λb衰减

测定 V ub | 来自独家重音B衰变

晶格QCD + QED中的光子轻子衰变速率

B s→Kℓν衰变中前导HQET形状因数的连续极限

Omnès改进的构​​成夸克模型的B→K半瘦子衰变

VUB116-16NO1;中文规格书,Datasheet资料.rar

VUB116-16NO1;中文规格书,Datasheet资料.pdf

一种缺失粒子的衰变研究方法

Vub_ENV:跟踪真实漏洞相关靶场环境搭建

最新资源

$$ | V_ {cb} | $$ | Vcb |的确定和$$ | V_ {ub} | $$ | Vub | 来自重音$$ \ Lambda _b $$Λb衰减

Omnès改进的构成夸克模型的B→K半瘦子衰变