TeV尺度标量鳞夸克的EFT现象学研究

52 浏览量更新于2024-07-16 收藏 1.42MB PDF 举报

"这篇论文探讨了TeV尺度标量鳞片夸克（Leptoquark, LQ）在有效场论（Effective Field Theory, EFT）框架下的现象学。作者构建了一个完整的SU(2)单重态标量LQ与标准模型粒子间的领先有效算子集，最高至维度六。他们证明，尽管可重归一化的LQ-轻子-夸克相互作用拉格朗日能解释B衰变中的超出标准模型现象，如B到D(*)τν'、B到K'±l∓，以及μ子的异常磁矩，但LQ的高维有效算子可能产生与轻子数违反相关的新型效应。这些效应包括单环和双环下亚电子伏特级别的Majorana中微子质量生成，介导中微子双β衰变，以及在对撞机中可能出现的新型LQ信号。在具有近似Z3代对称性的框架下，研究者关注第三代LQ（ϕ3），并指出一类一维五维LQ算子可能导致非对称的等电荷ϕ3ϕ3对产生，从而在大型强子对撞机（LHC）上产生低背景的同符号轻子信号。例如，在特定的LQ质量（Mϕ3约为1 TeV）和新物理尺度（Λ约为5 TeV）下，预计在13 TeV LHC的综合亮度达到300 fb-1时，可通过pp碰撞产生大约5000个τ+τ+和约500个τ-τ-事件，带有特定的喷流特征。" 文章详细讨论了EFT在LQ现象学中的应用，LQ作为标准模型之外的潜在新粒子，它们可能解释B衰变实验中的异常和μ子的异常磁矩。通过对LQ进行有效的操作元分析，作者揭示了LQ如何参与非标准的轻子数违反过程，这可能与中微子质量的产生、双β衰变和对撞机信号相关。特别是，他们关注了Z3代对称性下的第三代LQ，这种LQ的特殊性质可能导致在LHC上观察到的τ+τ+或τ-τ-的对称信号，这对实验探测提供了新的方向。该研究不仅深入探讨了LQ理论模型的构建，还为实验观测提供了具体预测，对于理解超越标准模型的物理现象具有重要意义，特别是在中微子物理学和强子对撞机实验领域。开放获取的标签意味着这篇论文的研究成果可供全球科学界自由访问和使用。

regime, since the corresponding cross section scales as

σðq

q → l

−

Þ ∝ y

, thus providing a complimentary

sensitivity to the LQ dynamics as the LHC [7,37,38,49–

52]; in particular yielding better access to larger LQ masses

where the QCD on shell LQ pair production channel is

suppressed.

IV. EFT BEYOND THE ϕSM FRAMEWORK

In this section we focus on the EFT extension of the

renormalizable Lagrangian in Eqs. (1)–(3), for the down-

type LQ ϕð3; 1; −

Þ. The effects of the NP which underlies

the ϕSM framework in Eqs. (1)–(3) can be parametrized by

a series of effective operators O

, which are constructed

using the ϕSM fields and whose coefficients are suppressed

by inverse powers of the NP scale Λ,

L ¼ L

ϕSM

∞

n¼5

n−4

ðnÞ

; ð4Þ

where n is the mass dimension of O

ðnÞ

and we assume

decoupling and weakly coupled heavy NP, so that n equals

the canonical dimension. The dominating NP effects are

then expected to be generated by contributing operators

with the lowest dimension (n value) that can be generated at

tree-level in the underlying theory.

Before listing the specific form of the higher dimension

operators, O

ðnÞ

, it is useful to denote their generic structure

in the form,

ðnÞ

∈ ϕ

; ð5Þ

where a, b, c, d are integers representing the multiplicity of

the corresponding factors: O

ðnÞ

contains a LQ fields ϕ or



, b Higgs fields H or

H, c fermionic fields ψ and d

covariant derivatives D. Group contractions and which

fields are acted on by the derivatives are not specified.

We find that there are only two possible dimension-five

operators involving the LQ Φð3; 1; −

Þ and the SM fields

—both violating lepton number by two units. To see that,

note that the dimension-five operators with c ¼ 0 in Eq. (5)

are all absent because of gauge invariance. Furthermore,

operators of the form ϕ

must contain the fermion

bilinear

, so that only a single gauge invariant

dimension five operator of this form survives [with two

possible SU(3) color contractions which are not specified],

ð5Þ

; ð6Þ

which violates lepton number by two units.

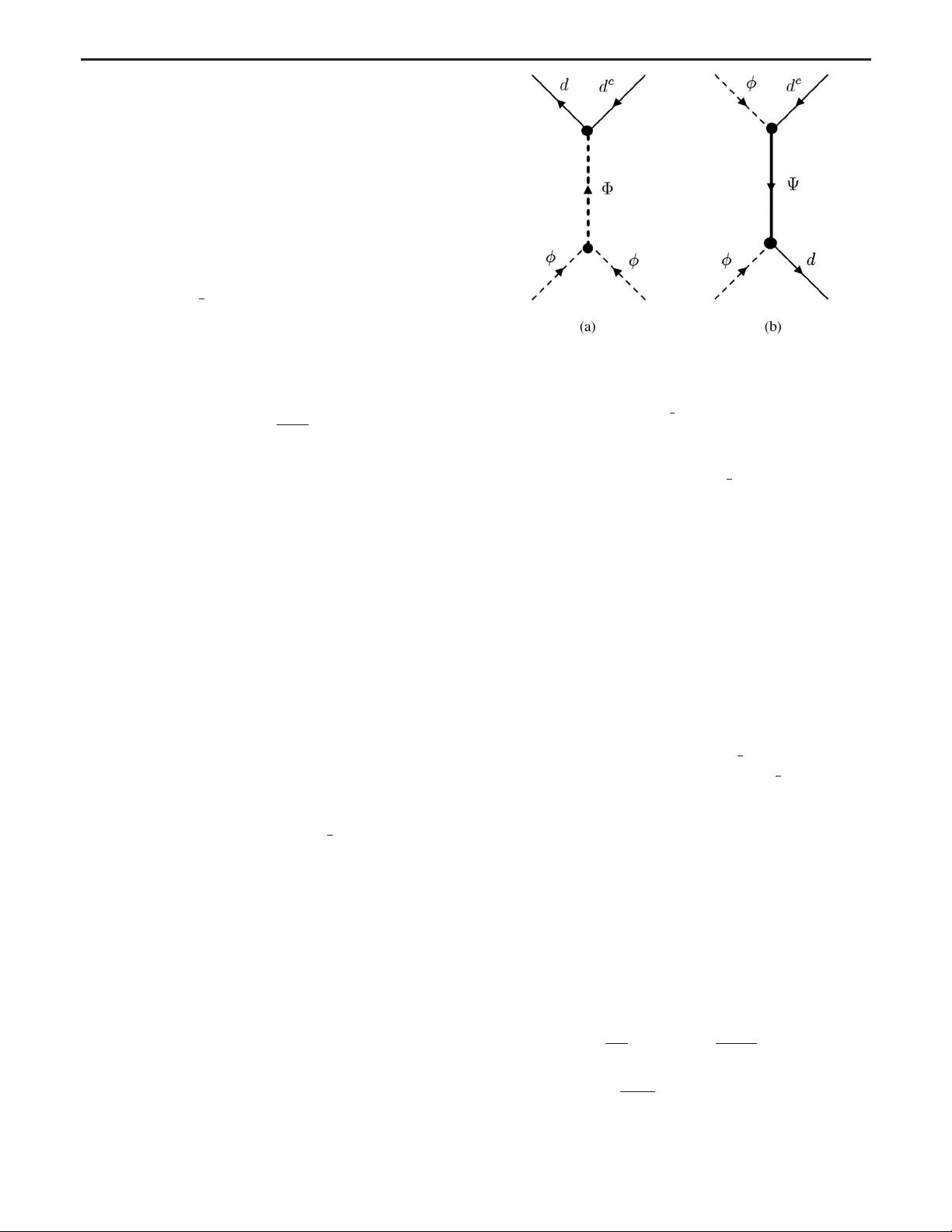
The diagrams that can generate the dimension five

operator

at tree-level in the underlying heavy theory

are depicted in Fig. 1; the corresponding heavy NP must

contain a heavy scalar Φð6; 1; −

Þ and/or the heavy

fermions Ψð1; 1; 0Þ, Ψð8; 1; 0Þ.

Dimension five operators of the class ϕψ

D can be

shown to be equivalent to operators without a derivative

using integration by parts and, therefore, can be ignored.

Thus, the remaining class of dimension five operators is of

the form ϕψ

H and, therefore, must also contain the

fermion bilinear

. The only gauge invariant operator

of this form, which also violates lepton number by two

units is

ð5Þ

ldϕH

Hϕ



: ð7Þ

The heavy physics generating this operator at tree-level

must contain a heavy scalar Φð3; 2;

Þ and/or the heavy

fermions Ψð1; 1; 0Þ, ψð1; 3; 0Þ or Ψð3; 2; −

Þ; see Fig. 2.

We recall that there is also a unique dimension five

operator that can be constructed using the SM fields only;

the so-called Weinberg operator [53],

ð5Þ

⋆

†

l; ð8Þ

that can be generated in the underlying theory at tree-level

by an exchange of a heavy scalar Φð1; 3; 0Þ and/or the

heavy fermions Ψð1; 1; 0Þ, Ψð1; 3; 0Þ.

Therefore, the overall dimension five effective operator

extension of L

ϕSM

ΔL

ð5Þ

ϕSM

⋆

†

l þ

ldϕH

Hϕ



þ H:c:; ð9Þ

FIG. 1. Tree-level graphs in the underlying heavy theory that

generate the dimension five effective operator

. Φ and Ψ

stand for a heavy scalar and heavy fermion, respectively, with

quantum numbers Φð6; 1; −

Þ and Ψð1 ; 1 ; 0Þ or Ψð8; 1; 0 Þ

(see text).

BAR-SHALOM, COHEN, SONI, and WUDKA PHYS. REV. D 100, 055020 (2019)

055020-4

剩余15页未读，继续阅读

weixin_38655987

粉丝: 8
资源: 933

TeV尺度标量鳞夸克的EFT现象学研究

标量产生和衰减到最高夸克，包括采用EFT方法对QCD中NLO的干扰影响

有效的场论和夸克行业的标量扩展

TTGammaEFT：在ttgamma中进行顶夸克耦合测量（EFT）的存储库

EFT中可以容纳多少流量？

标准模型EFT中的QCD校正适合WZ和WW生产

为QCD中的多区域处理在EFT中针对两个尺度的有效顶点计算单环校正

通货膨胀和兴奋的初始状态的一般扩展EFT中的非单位演化

flavio:标准模型及其他模型中用于风味物理学现象学的Python软件包

EFT2.16.0中文正式版V4.zip

无核EFT方法中核结合的开始

最新资源