消除单片三轴环形谐振器匹配误差方法

39 浏览量更新于2024-08-27 收藏 227KB PDF 举报

"消除单片三轴环形谐振器失配误差的方法" 在光学领域，尤其是激光技术和精密光学仪器的设计中，单片三轴环形谐振器（Monolithic Triaxial Ring Resonator，MTRR）因其独特的性能而受到广泛关注。【标题】中的“Method for eliminating mismatching error in monolithic triaxial ring resonators”着重讨论了如何解决MTRR中常见的失配误差问题。【描述】提到，通过运用增强的5×5光线矩阵方法，同时考虑镜面的轴向位移和三个平面正方形环形谐振器的不准确性，研究者得以揭示光轴扰动的规律，并成功消除了MTRR的失配误差C。失配误差C是MTRR在实际操作中普遍存在的一个问题，它可能导致谐振器性能下降，影响其作为光学传感器或光学腔体时的精度和稳定性。【标签】中的“空间三轴”和“失谐误差”强调了这个问题是在三维空间中对谐振器性能的影响。光学轴的扰动是导致失配误差的关键因素，可能源于制造过程中的微小偏差、安装误差或是环境因素引起的机械振动。文章中提到的增强的5×5光线矩阵方法是一种用于分析光路传播的工具，它能够更全面地考虑光学系统中各个组件的位置和角度偏差。通过这种方法，研究者可以系统地分析和校正MTRR内部各部分的不匹配，从而提高整体系统的性能。这种方法的应用对于优化腔体设计至关重要，同时也有助于MTRR的改进和对准过程。【标签】中的“140.4780,140.3410,140.3370,140.3560”是Optical Society of America（OSA）的Optical and Quantum Electronics (OQE)专题下的学科代码，这些代码可能代表了这篇研究涉及的具体光学子领域，例如激光技术、光学共振器或者光学传感等。这些代码为读者提供了更精确的分类信息，便于相关领域的研究人员快速定位和理解文章内容。该研究提供了一种有效消除单片三轴环形谐振器中失配误差的方法，这对提高光学谐振器的性能和可靠性具有重要意义，特别是在高精度测量和光学传感应用中。通过精细的光学分析和模型建立，研究者能够克服由于制造公差和环境因素导致的光学轴扰动，从而实现更高效、更稳定的光学系统设计。

Decemb er 10, 2010 / Vol. 8, No. 12 / CHINESE OPTICS LETTERS 1135

Method for eliminating mismatching error in monolithic

triaxial ring resonators

Xingwu Long (龙龙龙兴兴兴武武武) and Jie Yuan (袁袁袁杰杰杰)

∗

Department of Opto electronic Engineering, College of Optoelectronic Science and Engineering,

National University of Defense Technology, Changsha 410073, China

∗

E-mail: jieyuan@nudt.edu.cn

Received April 22, 2010

Several results on optical-axis perturbation and elimination of the mismatching error C of a monolithic

triaxial ring resonator (MTRR) are rep orted. Based on the augmented 5×5 ray matrix method, by

simultaneously considering axial displacement of a mirror and the misalignments in three planar square

ring resonators of a MTRR, the rules of optical-axis perturbation are obtained. The mismatching error

C of the MTRR is eliminated. The results obtained are important for cavity design, as well as in the

improvement and alignment of MTRR.

OCIS codes: 140.4780, 140.3410, 140.3370, 140.3560.

doi: 10.3788/COL20100812.1135.

This letter is a continuation of Ref. [1]. Ring laser has

been used as a rotate sensor. The planar or non-planar

resonator, as well as monoaxial or monolithic triaxial

ring resonator (MTRR), have been widely used for laser

gyroscopes

[2−9]

. Continuous research on optical-axis per-

turbation in ring resonators have been conducted

[10−15]

However, the perturbation sources in all the previous ar-

ticles have been on radial displacements or angular mis-

alignments of the optical components of the ring res-

onator. The perturbation source of the axial displace-

ment of a mirror has not been previously discussed. The

augmented 5×5 ray matrix method is used to handle

these perturbation sources.

The ray matrix of a general optical component with

angular misalignment and radial displacements has the

form:



















0 0 E

0 0 F

0 0 A

0 0 C

0 0 0 0 1



















, (1)

where r

, r

, and r

are the input ray and out-

put ray heights from the reference axis along the x and

y axes; this is referred to as optical-axis decentration.

Then, r

, r

, and r

are the angles that the in-

put and output rays construct with the reference axis in

the x and y planes, resp ectively; this is referred to as

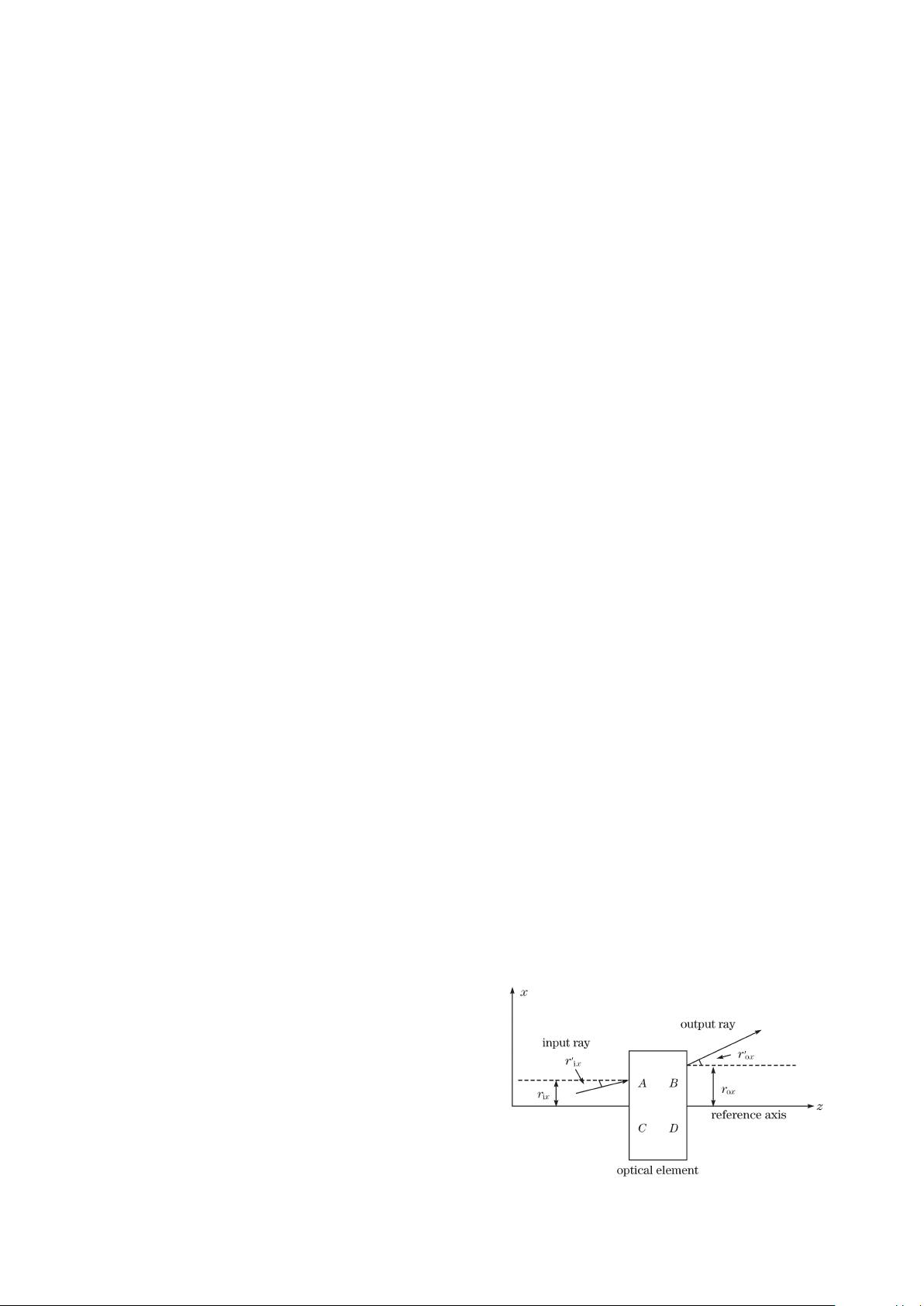
optical-axis tilts. In Fig. 1, x axis has been chosen as an

example to show the parameters. A

, B

, C

, and D

are the standard ray-matrix elements in the tangential

plane; A

, B

, C

, and D

are the standard ray-matrix

elements in the sagittal plane; and E

and E

are the de-

centration terms representing radial displacements along

x and y axes. F

and F

are the tilt terms represent-

ing the angular misalignments. In the case of a mirror,

= 2 tan(θ

), F

= 2 tan(θ

), and θ

and θ

are the

misalignment angles in its local tangential and sagittal

planes, respectively.

In Fig. 2

[1]

, a spherical mirror has been chosen as an

example to show the perturbation sources. M and M

represent the mirrors before and after axial displacement

δ along the normal z direction. Axes x and y are perpen-

dicular to z. Generally, the mirror has six movements,

which are the displacements along the x, y, and z axes,

and the rotations around the x, y, and z axes. The trans-

lation of the mirror along the x and y axes manifests as

radial displacements represented by E

and E

in the

augmented 5×5 matrix.

The rotary movements around the axes of x and y are

the angular misalignments of the mirror, and they can

be represented by the terms F

and F

in the augmented

5×5 matrix. The rotation of the mirror around the axis z

can be ignored because it has spherical symmetry. In this

letter, the displacement of the mirror along the z axis is

called axial displacement. It cannot be represented by

any term in the augmented 5×5 matrix in the current

framework of the study. Thus far, this axial displacement

has not been discussed in detail.

In this letter, the axial displacement of the mirror is

treated as the source of p erturbation along with the mis-

alignments. The augmented 5×5 ray matrix is modified

to include this perturbation. To the best of our knowl-

edge, this is the first time that such axial displacement

has been considered as a perturbation source. The square

ring resonator and MTRR are chosen as examples to

show its application. Optical-axis perturbation induced

by the axial displacement of the spherical mirror and the

corresponding misalignments can be obtained by utilizing

the modified 5×5 ray matrix. The method for reducing,

Fig. 1. Schematic diagram of the input and output rays.

1671-7694/2010/121135-04

° 2010 Chinese Optics Letters

下载后可阅读完整内容，剩余3页未读，立即下载

weixin_38688906

粉丝: 12
资源: 904

消除单片三轴环形谐振器匹配误差方法

A Method Framework for Automatic Airspace Reconfiguration-Monte Carlo Method for Eliminating Irregular Sector Shapes Generated by Region Growth Method

Eliminating noisy information in Web pages for data mining

Numerical method based on transfer function for eliminating water vapor noise from terahertz spectra

A Passive EMI Filter for Eliminating

Eliminating Memory Leaks in Symbian OS C++ Projects

A hybrid expansion method for FRFs of non-proportionally damped systems

ASTM E2011 - 21 Standard Test Method for Evaluation of Hygienic

ASTM E2613 - 14 ：2014 Standard Test Method for Determining Fungu

ASTM E2011 - 13：2013 Standard Test Method for Evaluation of Hygi

ASTM E2755 - 15：2015 Standard Test Method for Determining the Ba

最新资源