"解约束满足问题的形式化：CSP与算法介绍"

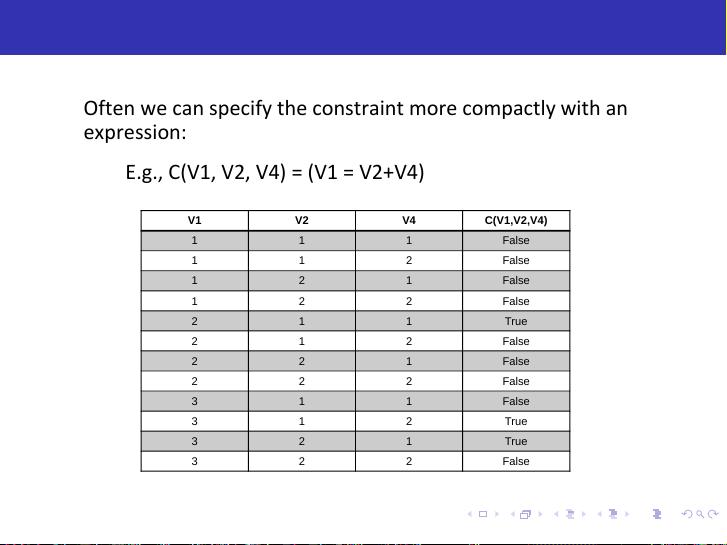
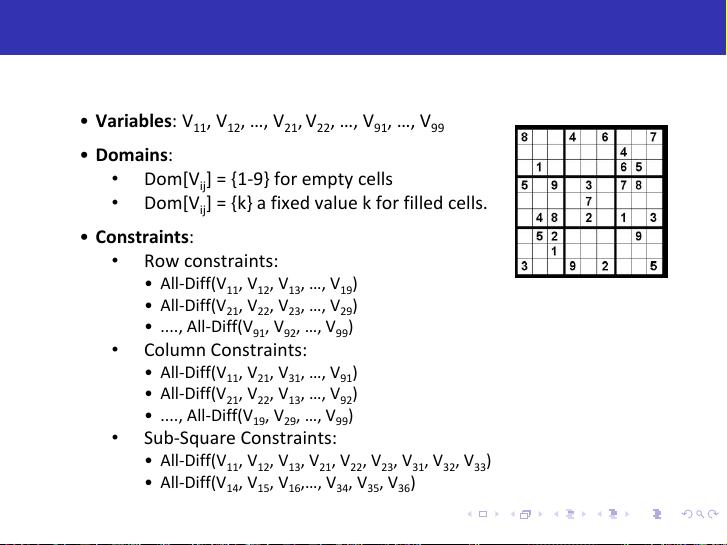
In Lecture 9, we delved into the complex world of Constraint Satisfaction Problems (CSP). A CSP involves finding a consistent assignment of values to variables that satisfies a set of constraints. This formalization of a CSP allows us to utilize various search algorithms to efficiently navigate through the possible solutions. One popular algorithm for solving CSPs is the Backtracking algorithm. This algorithm systematically explores potential solutions, backtracking when it reaches a dead end. By making informed decisions about variable assignments and leveraging constraints to prune the search space, the Backtracking algorithm can efficiently find a valid solution. Another algorithm discussed in the lecture is the Forward Checking algorithm. This algorithm utilizes constraint propagation to immediately eliminate values that violate constraints, reducing the search space and improving efficiency. By continuously updating the domains of variables based on previous assignments, Forward Checking can quickly converge on a valid solution. Additionally, the lecture introduced the Generalized Arc Consistency (GAC) algorithm, which enforces consistency by ensuring that every value in every domain is consistent with all constraints. By iteratively examining arcs and enforcing consistency, the GAC algorithm can efficiently prune the search space and find a valid solution. Overall, the formalization of CSPs and the introduction of specialized search algorithms such as Backtracking, Forward Checking, and GAC provide a powerful framework for tackling complex constraint satisfaction problems. By leveraging constraints and smart search strategies, we can efficiently navigate through the vast solution space and find optimal solutions to challenging CSPs.