异质性星核模型：线性与二次状态方程的超密恒星研究
138 浏览量
更新于2024-09-03
收藏 1.03MB PDF 举报
本文探讨的是具有不同状态方程的超高密度星核包络模型，特别关注的是超稠密恒星的结构特性。核心部分采用线性状态方程，这一设计与夸克物质的理论相一致，反映出核心内部可能存在的量子色动力学（QCD）相变现象。另一方面，包络部分则采用了二次状态方程，这种区分反映了核心和外部环境在物质性质上的差异。
作者们遵循了Takisa等人在《Pramana J Phys》(92:40, 2019)中的研究哲学，对模型进行了细致的构建。他们主要目标是确保模型的物理参数在核心区域是现实可行的，而在过渡到包络时保持连续性，以满足实际天体物理学的约束。通过严格的数学分析和理论计算，研究者展示了模型的稳定性和合理性。
模型的可行性得到了两个著名的奇异星——SAX J1808.4-3658和4U1608-52的观测数据支持。这两个天体被认为是极端环境下的典型例子，它们的物理特性与模型预测相吻合，进一步验证了模型的有效性。
随着恒星质量的增加，研究发现核心密度也随之提升，这与重力对于高质量天体的作用规律相符合，即质量越大，引力压缩越强。这表明模型不仅能够描述单个天体的行为，还能揭示天体演化和引力效应的普遍规律。
这篇文章不仅提供了一个理论框架，用于理解超稠密星的内部结构，还为探索宇宙中的极端物理条件提供了有价值的研究工具。其研究成果对于理解恒星内部物理过程，特别是核心与包络的交互作用，以及可能存在的新型物质状态，有着重要的科学价值。同时，它也推动了未来对高密度星体和可能存在的新型物质状态的进一步观测和实验研究。
Eur. Phys. J. C (2019) 79 :602 Page 3 of 11 602
3 Conditions for a physically realistic core-envelope
model
In order to make the model physically viable, one needs to
verify the following conditions in core, envelope and exte-
rior regions (conditions developed by [47] are further aug-
mented):
(i) Geometrical regularity: The metric potentials and mat-
ter variables should be defined at the center and should
be well behaved throughout within the star [47].
(ii) Density and pressures trends: The matter density ρ,
radial pressure p
r
and transverse pressure p
t
the core
and envelope of the star should be continuous at the
junction, positive and monotonically decreasing out-
ward. Further, the pressure-density ratios should be
positive and less than 1 throughout within the star (Zel-
dovich’s condition [51]) and continuous at the junc-
tion.
(iii) Mass-radius relation, Red-shift and Compactifica-
tion factor: The mass function m(r), compactification
parameter u(r) and gravitational red shift z(r) for the
core and the envelope of the star should be continuous
at the interface and increasing and decreasing respec-
tively with the radial coordinate r.
(iv) Anisotropic constant: The radial pressure should coin-
cides with the tangential pressure at the core of the star
and should be continuous at the interface besides being
asymptotic and increasing outward.
(v) Causal nature of radial sound speed: The radial sound
speed of a compact star model should satisfy the
causality condition at the center and should be mono-
tonically decreasing outward besides being continuous
at the interface.
(vi) Adiabatic index: The adiabatic index should be con-
tinuous at the interface and should satisfy the Bondi
condition.
(vii) Energy conditions: The core and the envelope for the
star should satisfy the energy conditions besides being
continuous at the interface.
(viii) TOV equation: The TOV condition should be satisfied
within the star and all the three forces should be con-
tinuous at the interface resulting the system to be in
static equilibrium.
(ix) At the stellar boundary p
r
(R
E
) = 0[47].
(x) The metric potentials of the core region should match
smoothly with the gravitational potentials of the enve-
lope region [47].
(xi) The gravitational potentials of the envelope layer
should connected smoothly over the boundary with
the Schwarzschild exterior metric [47].
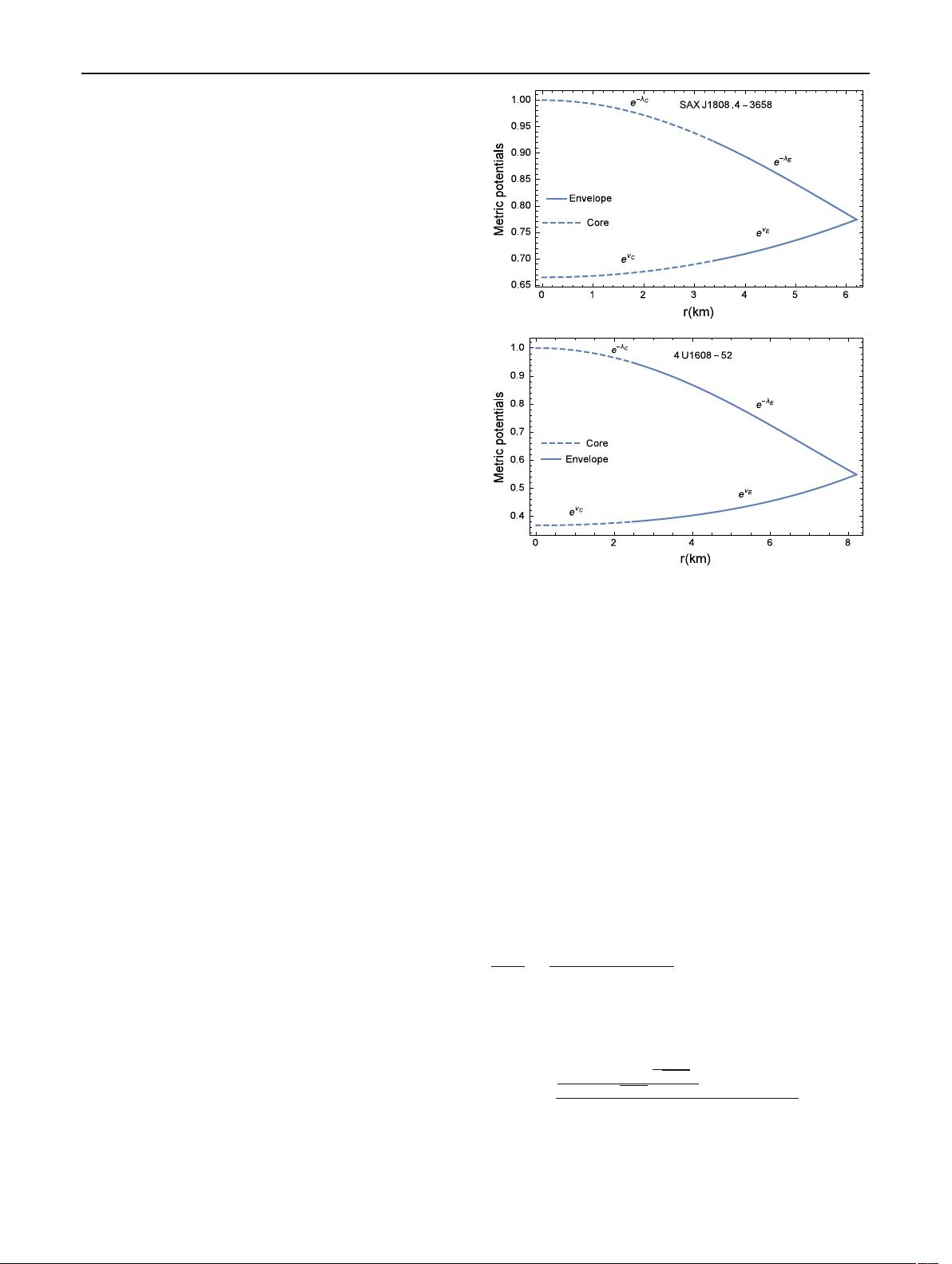
Fig. 1 Variation of metric potentials with radial coordinate r for (i)
SAX J1808.4-3658 (above) with mass M = 0.7M
and radius R
E
=
6.2 km for the values of b = 0.000035/km
2
, B = 0.00025/km
2
, A =
90/km
2
, α = 0.1409/km
2
, R
C
= 3.393 km; (ii) 4U1608-52 (below)
with mass M = 1.85M
and radius R
E
= 8.2 km for the values of b =
0.00003/km
2
, B = 0.00005/km
2
, A = 155/km
2
, α = 0.312/km
2
,
R
C
= 2.459 km
4 The core-envelope model
For core region (0 ≤r ≤ R
C
), we choose Tolman VII type of
g
11
for the gravitational potential e
λ
satisfying a linear EOS
z = e
−λ
C
= 1 − ax + bx
2
, (15)
p
r
C
= αρ − β, (16)
where a, b ,α, and β are constants. Substituting z value from
Eq. (15)inEq.(11) and using Eqs. 8, 9, 15 and 16 , we obtain
the following differential equation:
y
(x)
y(x)
=
P
1
+ P
2
x
2
−ax + bx
2
+ 1
. (17)
On integrating Eq. (17), we get
y = e
ν
C
= P
3
e
⎛
⎜
⎜
⎜
⎜
⎜
⎝
2
(
aP
2
+2bP
1
)
tan
−1
2bx −a
√
4b−a
2
√
4b−a
2
+P
2
log
(
−ax+bx
2
+1
)
4b
⎞
⎟
⎟
⎟
⎟
⎟
⎠
, (18)
123
剩余10页未读，继续阅读
2020-03-26 上传
137 浏览量
2017-10-21 上传
2021-05-12 上传
2022-01-27 上传
2021-10-12 上传
weixin_38748207
- 粉丝: 7
- 资源: 917
我的内容管理
展开
