WANG et al.: 5.35-mW 10-MHz SINGLE-OPAMP THIRD-ORDER CT MODULATOR 2785
Fig. 4. Block diagram of the proposed 3rd-order ADC.
Besides SAB, the passive filter can also enhance the LF
order with a small power overhead [18], [19]. As Fig. 3(b)
shows, higher order can be obtained with passive components,
including resistors and a capacitor; however, such passive inte-
grator exhibits a drawback because its TF is non-ideal which
affects the inband quantization noise attenuation. We will
analyze its design impact in Section III-C.
B. Proposed Modulator
The SAB jointly with the passive integrator replace
the power-hungry 1st–3rd integrators from the architecture
in Fig. 2. We implement the first and second integrators,
as well as the first feedforward path, with an improved
version of the SAB integrator. Further, the passive integrator
substitutes the last integrator and the second feedforward path.
Such combination enables a 3rd-order LF implementation with
one opamp, thus significantly reducing the power consumed
by the integrators. The passive integrator also allows ELD
compensation without any active adder. Fig. 4 shows the
circuit schematic of the proposed modulator with the other
parts in the block format. We use non-return-to zero (NRZ)
current-steering DACs (DAC1/DAC2) to alleviate the impact
of the clock jitter and improve the power supply rejection ratio
(PSRR). The value of the input resistor (R1) is determined by
the noise requirement of the modulator, which simultaneously
decides the conducted current of the DAC1 and capacitor
loads (C1), implying that the value of R1 induces a tradeoff
between the noise budget and power of the DAC and opamp.
In the modulator, we choose R1 = 1k to balance such
tradeoff with the target SNR specifications for low power.
In [16], a feedback resistor is introduced to improve the SQNR
by optimizing the zero in the NTF, but its induced non-
shapeable thermal noise indeed yields a diminishing return on
the overall SNR. The proposed SAB integrator removes the
feedback resistor in the RC biquad which alleviates a non-
shapeable noise source thus improving the overall SNDR of
the modulator.
DAC1 and DAC2 are the main feedback and the ELD com-
pensation DAC, respectively. We adopt an SAR architecture
in the 4-bit QTZ due to its outstanding energy efficiency and
no offset issue with only one comparator, which is critical in
our design as the passive integrator attenuates the input swing
of the QTZ to about 0.5 V
pp
. The ELD is introduced by the
Fig. 5. STF and NTF magnitude response for 3rd-order LF, and 2nd-order
LF + passive integrator.
QTZ and feedback path, which is caused by the sampling of
the SAR QTZ and the latching operation of the main DAC.
The proposed modulator also shows a strong stability even
with a high out-of-band gain which benefits from the SAB
together with the passive integrator, as it is detailed next.
C. Passive Integrator and ELD Compensation
Even though the passive integrator can supply extra noise
shaping with little power overhead, it imposes that the TF of
the LF is non-ideal as shown by the follow TF of the proposed
modulator
H(s) =
⎛
⎝
1
R
1
C
1
s +
1
R
1
R
2
C
2
1
s
2
+ k
DAC2
⎞
⎠
×
R
4
R
3
+R
4
s + k
3
s + k
3
,
k
3
=
1
C
2
(R
3
+ R
4
)
(1)
where k
DAC2
is the feedback coefficient of DAC2. Based
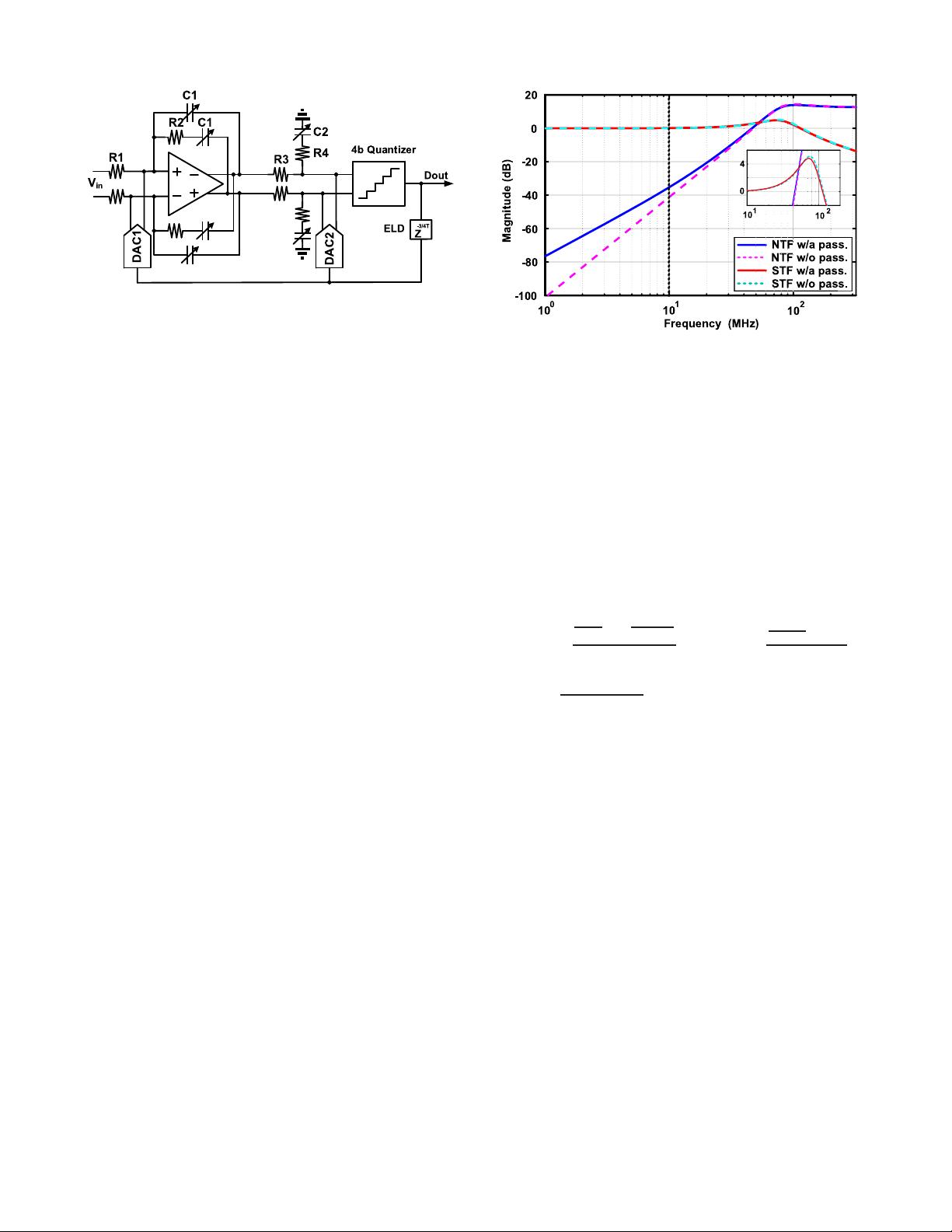
on (1), Fig. 5 shows the STF and NTF of the modulator
with and without the passive integrator. The STF peak results
from the CIFF-FB architecture, which has the feedforward
path in the LF. When compared with the STF of the ideal
3rd-order modulator, the STF peak drops from 5.1 to 4.8 dB
because the non-ideal effect of the passive integrator. A zero
of the NTF is pushed away from DC to the left plane
on the S-domain with the location of the zero determined
by k3. It worsens the inband quantization noise attenuation
of the modulator. But the influence is not significant when the
bandwidth of the modulator is small. Fig. 6 shows the peak
SQNR of the 2nd-order LF, 3rd-order LF, and 2nd-order LF +
passive integrator modulator under various out-of-band gains.
If compared with the ideal 3rd-order LF, the SQNR of the
passive integrator drops from 107 to 101 dB. Such influence
is negligible since our target is around 82 dB. On the other
hand, the passive integrator combined with the 2nd-order LF
enables an improvement of ∼10 dB in the SQNR with a small
power overhead.
In the proposed modulator the passive integrator is also used
as a passive adder to achieve ELD compensation. The feedback
current of DAC2 is added at the output of the LF where its
swing is small because of the passive integrator’s attenuation.









