134 CHINESE OPTICS LETTERS / Vol. 7, No. 2 / February 10, 2009
Evanescent waves of an annular left-handed material lens
Changchun Yan (
AAA
SSS
)
1,2
, Yiping Cui (
www
²²²
)
1∗
, Qiong Wang (
)
1
, and Changgui L¨u (
½½½
BBB
)
1
1
Advanced Photonics Center, School of Electronic Science and Engineering, Southeast University, Nanjing 210096
2
School of Physics and Electronic Engineering, Xuzhou Normal University, Xuzhou 221116
∗
E-mail: cyp@seu.edu.cn
Received September 16, 2008
The imaging system formed by an annular left-handed material (LHM) lens as well as the evanescent
waves in the lens are simulated numerically with a finite-difference time-domain (FDTD) method. For
b − a > λ (a and b are respectively the inner and outer radii of the annular lens, and λ is the wavelength),
when a point source is placed at an internal grid point, we demonstrate that the evanescent waves are
produced around the internal interface, and cannot propagate outwards. As for b − a < λ, the evanescent
waves appear around both the internal and the external interfaces, which remarkably implies the coupling
between the two interfaces. Hence it can be inferred that the evanescent waves around the external interface
participating in the super-resolution imaging result from the coupling of the evanescent waves around the
interface. Moreover, the partly uncomprehended properties of the evanescent waves in the LHM slab are
also disclosed. It is conducive to understanding the evanescent waves in the LHMs further.
OCIS codes: 160.4760, 260.5740, 100.6640.
doi: 10.3788/COL20090702.0134.
It has been near ly 40 years since Veselago proposed that
the combination of negative permittivity (ε < 0) and neg-
ative permeability (µ < 0) leads to a negative refractive
index
[1]
. However, the first demonstration of left-handed
materials (LHMs) was in 2000
[2]
, which causes increas-
ing interests of researchers. At present, great progress
has been made in producing the LHMs
[3−11]
. The ex-
treme attention to the LHMs results from their unique
properties and potential applications, such as reversal
of both the Doppler shift and Cherenkov radiation
[1,12]
,
negative refra c tion
[13]
, imaging of sup e rlens
[14−17]
, en-
hanced nonlinearity
[18]
, specific field distribution of
guided modes
[19]
, etc. Undoubtedly, one of the im-
portant properties of the LHMs is the amplification of
evanescent waves
[20,21]
. This amplification leads to the
supe r-resolution imaging in the near fields
[16,17,22,23]
. In
the past, the researchers more examined the eva nescent
waves of a LHM planar lens
[14,22,24−26]
. Although Pendry
et al. ever discussed about the annular LHM lens, they
paid attention to its perfect imaging but less studied its
evanescent waves
[27,28]
.
In this letter, the full-wave imaging of an annular LHM
lens is s imulated with a finite-difference time-domain
(FDTD) method. The evanescent fields around the in-
terfaces of the lens are investigated. The simulations
and analyses show some characteristics of the evanes-
cent waves in the annular lens. It is conducive to un-
derstanding the evanescent waves in the LHMs further.
Meanwhile, from these characteristics, we can infer that
the coupling of the evanescent waves results in the super-
resolution imaging.
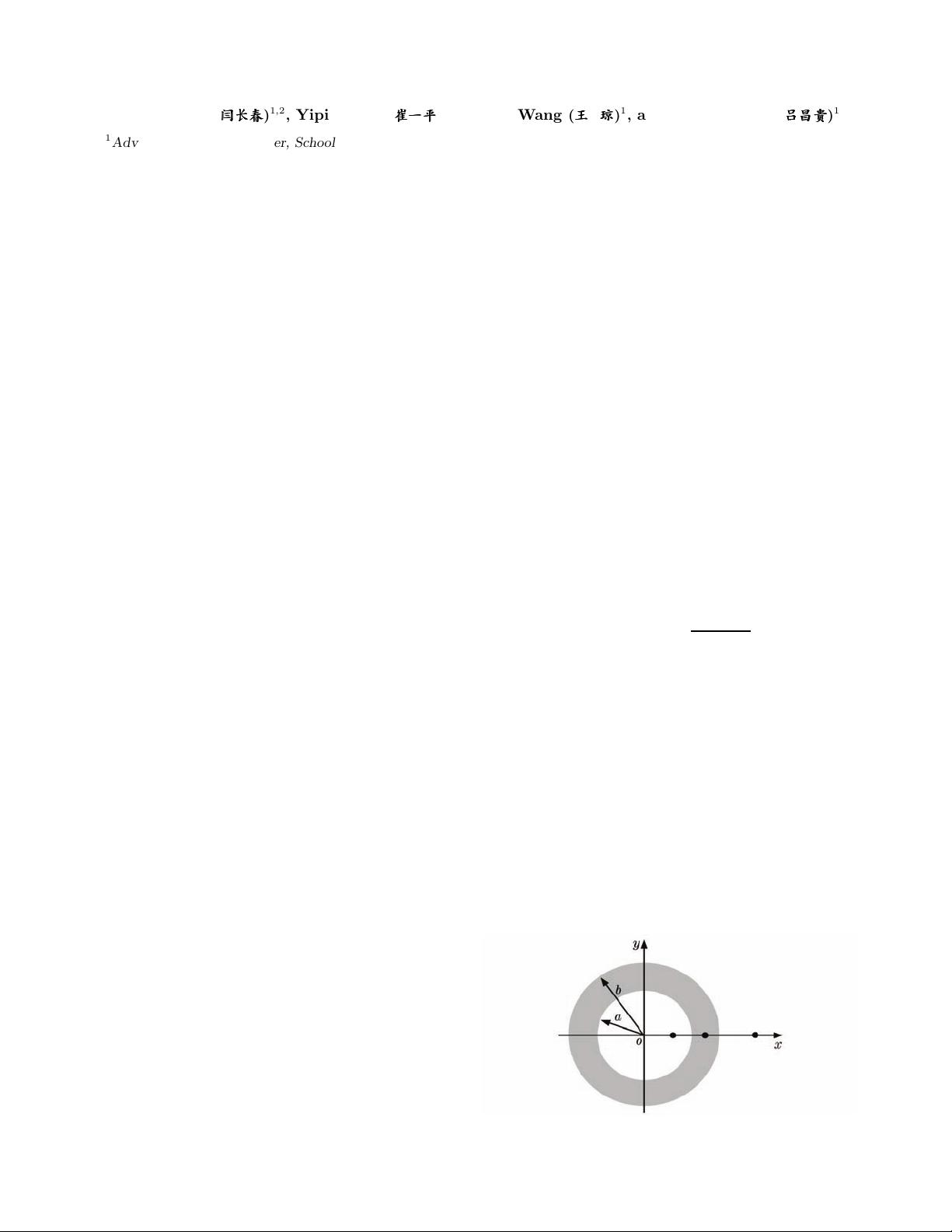
The two-dimensional (2D) imaging sys tem we consider
here contains an annular LHM lens, which is surrounded
by va c uum, with an internal radius a and an external ra-
dius b (see Fig. 1). Following the description in Ref. [28],
the annular lens can produce a magnified image of an
internal object, and a demagnified image of an external
object. Namely, the object closer than r = b
2
/a can form
a demagnified image inside the annulus. Conversely, the
objects within the annulus and farther than r = a
2
/b to
the center will produce a magnified image outside the an-
nulus. This statement will be verified below. We assume
2D transverse electric fields in a cylindrical coordinate
system (E
z
, H
θ
, H
r
6= 0). The lens is supposed to be
isotropic and characterized by relative permittivity and
permeability of identical plasmonic form as
[29,30]
ε
r
(ω) = µ
r
(ω) = 1 −
ω
2
p
ω
2
− iωτ
, (1)
where ω
p
is the plasma frequency and τ is the colli-
sion freq uency. Emphatically, although the isotropic
medium is assumed in our s imulations, the simulation
results below are similar to those for the anis otropic
medium in Ref. [28]. In addition, a point source is ex-
pressed as E
z
(r, θ, t) = E
z
(r, 0, t) = sin(ωt). Choos-
ing ω = 1.885 × 10
11
rad/s, ω
p
= 2.6658 × 10
11
rad/s, and τ = 1.885 × 10
8
rad/s, we can obtain
ε
r
(ω) = µ
r
(ω) = −1 − iγ = −1.0 − 0.002i (refractive
index n = −1.0 − 0.002i). Obviously, the annular lens
is a matched and loss material. We employ the FDTD
method and a perfectly matched layer (PML) bound-
ary condition in the cylindrical coordinate system
[31]
to
simulate the electr omagnetic fields. A spatial step of
∆r = λ/30, an angle step o f ∆θ = 2π/300, a time step of
Fig. 1. Schematic of an annular HLM lens with marks.
1671-7694/2009/020134-04
c
2009 Chinese Optics Letters









