光子与量子点自旋量子比特间的高效信息转移

105 浏览量更新于2024-08-31 收藏 358KB PDF 举报

"量子信息在光子与量子点自旋量子比特之间的转移" 这篇科研文章提出了无需辅助粒子的高效量子信息传输方案，涉及光子与量子点（QD）自旋量子比特之间的交互。在光学微腔环境中，这种信息转移不仅能够实现单个光子与量子点自旋量子比特之间的转换，而且可以扩展到两个光子或两个量子点自旋量子比特之间的通信。这些提议的关键优点在于，即使在高泄露率条件下，也能保持高保真度的传输效率。信息传输过程中，每个步骤都被视为一种受控非门（CNOT）操作。CNOT门是量子计算中的基本逻辑门，能够实现两个量子比特间的相互作用，这对于量子信息处理和量子计算至关重要。文中指出，这种信息转移等效于执行一个CNOT门操作，这为构建更高效的量子信息网络和量子计算机提供了可能性。光学微腔作为量子信息处理的平台，其作用在于增强光与物质的相互作用，使得量子比特与光子之间的耦合更加有效。在实际应用中，这样的技术对于实现长距离量子通信、量子中继以及量子互联网等有着重大意义。此外，由于该方案在高泄漏率下仍能维持高保真度，因此它对实际系统中的噪声和损耗具有一定的鲁棒性。文章中提到的OCIS代码（Optical and Quantum Communication, Information Science, and Systems）包括270.0270, 270.5565, 和270.5585，这可能分别对应量子通信、量子信息科学和量子系统等相关研究领域。文章的发表日期为2015年11月18日，接受日期为2016年3月11日，并于2016年5月3日在线发布，由南华师范大学纳米光电功能材料与器件实验室的赵瑞通和梁瑞生共同撰写，其中梁瑞生为通讯作者。文章的doi编号为10.3788/COL201614.06，可用于查找原始文献。这些研究成果为量子信息技术的发展开辟了新的途径，有望推动量子计算和通信的进一步发展。

Quantum information transfer between photonic and

quantum-dot spin qubits

Ruitong Zhao (赵瑞通) and Ruisheng Liang (梁瑞生)*

Laboratory of Nanophotonic Functional Materials and Devices, School for Information and Optoelectronic Science and

Engineering, South China Normal University, Guangzhou 510006, China

*Corresponding author: liangrs@scnu.edu.cn

Received November 18, 2015; accepted March 11, 2016; posted online May 3, 2016

We propose schemes for the efficient information transfer between a propagating photon and a quantum-dot

(QD) spin qubit in an optical microcavity that have no auxiliary particles required. With these methods, the

information transfer between two photons or two QD spins can also be achieved. All of our proposals can work

with high fidelity, even with a high leakage rate. What is more, each information transfer process above can also

be seen as a controlled-NOT (CNOT) operation. It is found that the information transfer can be equivalent to a

CNOT gate. These proposals will promote more efficient quantum information networks and quantum

computation.

OCIS codes: 270.0270, 270.5565, 270.5585.

doi: 10.3788/COL201614.062701.

The faithful transfer of quantum information and logical

operation between a propagating photon and a stationary

qubit plays a significant role in quantum information

science such as quantum networks

[1]

, quantum repeaters

[2]

and optics quantum computing

[3,4]

, since photons are the

perfect candidates for fast and reliable long-distance

communication, while stationary qubits are suitable for

processor and local storage. Some schemes for the interac-

tion between photonic and stationary qubits, for example

atomic qubits

[5,6]

, have been reported. In recent years,

semiconductor quantum dots (QDs) have attracted exten-

sive attention. As romising solid-state qubits, single spin

confined in QD has a long coherence time

[7,8]

and a

potential for integration on a chip

[9,10]

. Moreover, QD

manipulation has had significant progress

[11–13]

. Many

quantum communication and quantum computation

schemes have been proposed based on QD spins combined

with optical microcavities such as entanglement measure-

ments

[14,15]

, quantum logic gates

[16–19]

, entanglement gener-

ators

[20]

, and quantum repeaters

[21]

. In 2013, Gao et al.

experimentally demonstrated the transfer of quantum in-

formation carried by a photonic qubit to a QD spin qubit

using quantum teleportation

[22]

. They generated an en-

tangled spin-photon state in a QD and interfered the pho -

ton with a single photon in a superposition state of two

colors, i.e., a photonic qubit in a Hong–Ou–Mandel

setup. A coincidence detection at the output of the inter-

ferometer heralds a successful teleportation. The demon-

stration of successful quantum teleportation of photonic

and QD spin qubits could promote the realization of

on-chip quantum networks based on semiconductor

nanostructures.

The interaction between a circularly polarized beam of

light and a QD-cavity system is introduced first. Then, we

propose information transfer between a photon and a QD

spin in an optical microcavity, with no auxiliary particle

required. The information transfer between two photons

or two QD spins is proposed with a QD spin or a photon

as auxiliary photon. All of the information transfer proc-

esses above can be achieved deterministically and can be

seen as controlled-NOT (CNOT) gates for quantum

computation.

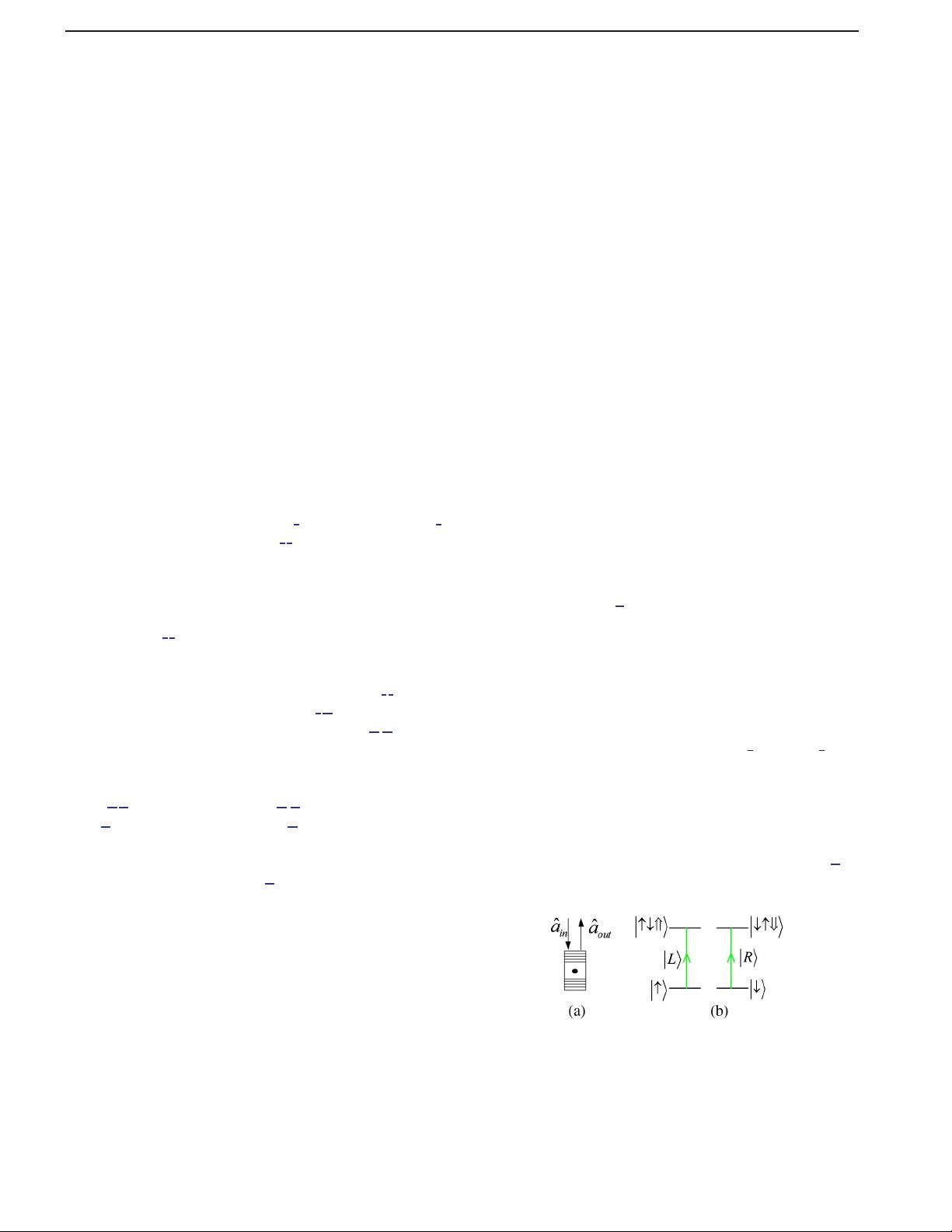
Considering a singly charged QD in an optical micro-

cavity shown in Fig.

1, if the injected excess electron spin

is in the state j↑i, the QD-cavity system resonantly ab-

sorbs a left-handed circularly polarized beam of light

jLi and creates a negatively charged exciton in the state

j↑↓⇑i. If the injected excess electron spin is in the state j↓i,

the QD-cavity system resonantly absorbs a right-handed

circularly polarized beam of light jRi and creates a nega-

tively charged exciton in the state j↓↑⇓i. Here, j⇑i and j⇓i

represent the heavy-hole spin states jþ

i and j −

i, re-

spectively. Due to this spin selection rule, the L- and

R-light encounter different phase shifts after reflection

from the cavity syste m.

The Heisenberg equations for the cavity field operator

and the QD dipole (X

−

) operator ˆσ

−

in the interaction

picture, and the input-output equation are given by

[23]

Fig. 1. Spin-dependent transitions for negatively charged exci-

ton X

−

. (a) A charged QD inside a micropillar microcavity with

circular cross section. (b) The spin selection rule for optical

transitions of negatively charged exciton X

−

due to the Pauli’s

exclusion principle. L and R represent the left- and the right-

hand circularly polarized lights, respectively.

COL 14(6), 062701(2016) CHINESE OPTICS LETTERS June 10, 2016

下载后可阅读完整内容，剩余4页未读，立即下载

weixin_38647039

粉丝: 7
资源: 943

光子与量子点自旋量子比特间的高效信息转移

Quantum Computation and Quantum Information

Optical cross-talk reduction in a quantum-dot-based full-color micro-light-emitting-diode display by a lithographic-fabricated photoresist mold

Slow and fast light in quantum-well and quantum-dot semiconductor optical amplifiers

Antiresonance in multi-quantum-dot system and its application as a spin filter

Information-and-Computation-Classical-and-Quantum-Aspects

MATLAB模拟粒子散射代码-Quantum-Open-Dynamics-and-Gaussian-Information-Linear-Op

A scheme for quantum state transfer between distant atoms

Entanglement Sudden Death and Manipulation in Double-Quantum-Dot Driven by Cavity Fields with Phonon Baths

Swap of Quantum Information between Two-End States in an Open Ferromagnetic Spin Chain

Hybrid entanglement concentration using quantum-dot and microcavity coupled system

最新资源