没有合适的资源?快使用搜索试试~ 我知道了~
首页光学设计实战:Zemax计算机辅助应用
"《实用计算机辅助镜头设计》是关于Zemax光学设计的英文经典书籍,由Willard S. Bell公司出版,作者通过本书分享了他四十多年的光学研究经验,涵盖了从基础理论到实际应用的广泛知识。"
在光学设计领域,Zemax是一款广泛使用的软件工具,它能够帮助设计师进行复杂的光学系统建模、优化和分析。本书"Practical Computer-Aided Lens Design"深入浅出地介绍了如何利用Zemax进行计算机辅助光学设计。作者将自己在光学科学领域的深厚背景与实践经验相结合,为读者提供了详尽的指导。
全书内容可能包括以下几个主要知识点:
1. **光学基础知识**:涵盖光的基本性质,如光的传播、反射、折射和干涉等,这些是理解光学设计的基础。
2. **Zemax软件介绍**:讲解Zemax的界面、工作流程,以及如何创建和编辑光学元件模型,包括透镜、反射镜、光阑等。
3. **光学系统建模**:介绍如何使用Zemax构建实际光学系统的几何模型,包括系统配置、光线追迹和像差分析。
4. **光学设计优化**:讨论如何通过Zemax的优化工具调整系统参数,以达到最佳性能,例如最小化像差、提高分辨率或改善光束质量。
5. **实际应用案例**:可能包含多个实际光学设计案例,如相机镜头、望远镜、激光器等,展示如何应用所学知识解决实际问题。
6. **光束传播和成像理论**:深入探讨光束在不同介质中的传播,以及如何通过光学系统形成清晰的图像。
7. **非理想条件下的光学设计**:包括考虑像差校正、色散、热效应等因素对设计的影响,以及如何处理这些复杂情况。
8. **实验与实践**:可能提供一些实验设计和数据分析的建议,帮助读者将理论知识转化为实际操作能力。
9. **光学材料和制造**:介绍不同光学材料的特性,以及如何选择和处理这些材料以满足设计需求。
10. **光学设计的工程和生产考虑**:讨论光学设计与实际制造过程的接口,包括公差分析和成本估算。
这本著作不仅是对光学设计理论的全面阐述,也是对Zemax软件应用的实用指南,适合光学工程专业学生、研究人员和从业人员学习参考。作者通过个人经历和对光学的热爱,将理论与实践完美融合,为读者呈现了一部宝贵的光学设计资源。
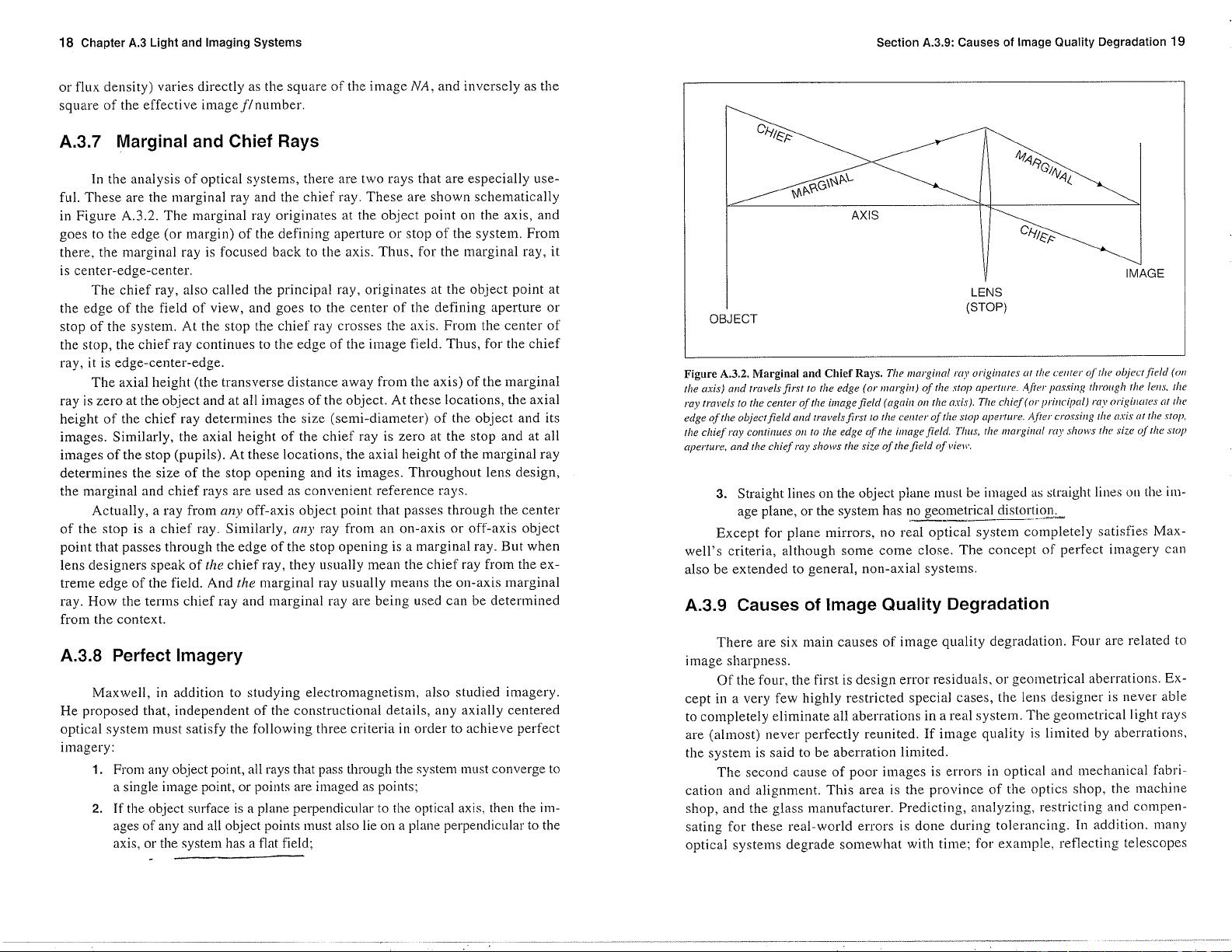
18 Chapter 4.3
L¡ght and lmaging Systems
or flux
density)
varies directly as the square of the
image NA. and iuversely as the
sqr-rale of
the eflective
inragefTnurnber.
A.3.7
Marginal and Chief Rays
In the analysis of
optical
systems,
thele are two
rays
that are especially use-
ful. These are
the marginal ray and
the chief ray. These ale shown schernatically
in Figure A.3.2. The
rnalginal ray originates
at the object
point
on the axis,
and
goes
to the edge
(or
niargin) of the defining
aperture ol' stop of the system. Fron]
thele, the rnarginal ray is focused
back to the axis. Thus,
for the rnarginal ray, it
is center-edge-center.
The chief ray, also callecl
the
principal
ray, originates
ât the object
point
at
the edge of the field of
view,
ancl
goes
to the
center of the defining aperture or
stop of the systern. At
the stop the chief l'ay
crosses the axis. Fror¡ the
center of
the stop, the chief
ray continues to the
edge
of
the iruage field. Tlius,
for the chief
ray, it is edge-center'-edge.
The axial height
(the
transverse distance
away frorn the axis) of the rnarginal
ray is zero
at the
object
and at all irnages of
tlie object. At these locations,
the axial
height of
the chief ray cletermines
the
size
(semi-diameter)
of
the
object
and its
images. Similarly,
the axial height of the
chief ray is zero at the stop and
at all
irnages
of the stop
(pupils).
Atthese locations, the axial
height of the marginal ray
detennines the size of
the
stop
opening and its images.
Throughout lens design,
the marginal and chief rays
âre usecl as convenient reference rn¡'s.
Actually,
a
lay
frorn any off-axi" object
point that
passes
through the
center'
of
the stop is a chief lay. Simiiarly,
cuÐ'ray ftom au on-axis
ol off-axis object
point that passes through the edge of the stop opening
is zr rnarginal ray. But
when
lens
designers s¡reak of ¡åe chief
ray, they usually meau the
chief ray
fi'orn
the ex-
trenre edge of the fieìd. And the
rrarginal ray usually means the on-axis
rnarginal
ray. How the tel'lns chief ray
and marginal ray ate being
used car-r be detenniued
from
the context.
A.3.8
Perfect lmagery
Maxwell, in acldition to studying
electromagnetisrn. also studied
imagery.
He
ploposecl tlrat, independent of the colìstructiou¿ìl
details, any axially centered
optical
system
must
satisfy the following
three cliteria in older to
achieve
^erre¡r
imagery:
1. Flonr any ob.ject
point, atl rays that
pass
thlor-rgh
the system lnust converge to
a
single
irnage
point.
or
points
are iniaged
âs poirìts;
2. If the ob-ject surfàce is a
plane perpendicular to the optical axis. then
the irl-
ages of
any and all
object
points
must also lie on a
¡rlane
perpencliculal to tlre
axis,
or the system has
a flat fietd;
Section
4.3.9: Causes
of lmage Quality
Degradation 19
OBJECT
Figure
4.3,2, Marginal
ancl Chief Ra¡'s.
The marginal
ray
origittatas
ot tlrc centur oJ tlrc object
fie
ld
(on
the
axis) and rra,eLs
first
to the edge
(or
mdrgin) of
the
,stop
apciltrre. A.lter
pttssin¡¡
through
the lens, the
t ¿r\i
tt'ot)els lo the centcr of
lhe innge
field
(again
on î\rc a¡ìs).
Tlrc chic.f
(or
prittt i¡tal) rav oritirtttlt's
ot tlu'
edge
of the
object
.field
and tntvels
JirsÍ
to tllc certcr of llrc sto¡t
operturc. Alter
crossirtg tltc axis
ttt the sto¡t,
the chief
ra,t,
t:onliuucs
on to Ilrc edge
of rhe imaga.field.
TltLts, thc
ntargittul
trt-v.r/tott,s
(h?
si¿a o.Í
lha
sto!)
apeilure,
and tlrc chief ra¡' sltows
the size of the.fiald
rtJ viev.
3. Stlaight
lines on the object
plane must be
intagetl as stlaight
lines
on the iru-
age
plane,
01'the
system has
1:
S:g-melrical
distorti,on.
Except for
plane mirrors, no
t'eal optical systenl
cornpletely
satisfies
Max-
well's
criteria, although
some come
close. The
concellt of
perfect itnagely
can
also be
extended to
general,
non-axial
systetns.
4.3.9
Causes
of lmage
Quality
Degradation
There
ale six main
causes of
irnage
quality degraclation.
Fout'
are related
to
image
sharpness.
Of
the four, the first is
design error
residuals, or
geonletrical alrellatious.
Ex-
cept in
a very few highly
restricted special
cases,
the lens designer
is
uever
able
to cornpletely
elirninate ail
aberlations in
a
real
system.
The
geornetlical
light fays
are
(almost)
never
perfectly
leunited.
If image
quality is limited by
aberrations,
the systeln is said
to be aberration
limited.
The second
cause of
pool
images
is ellors
in optical
and urechanical
fabl'i-
cation and
alignruent. Tliis
area is the
plovince of
the optics sliop,
the
machine
sho¡t, ar-rd the
glass lnanufacturer'.
Predicting,
artal5,7i¡1o,
r'estlictiug
and
cotnpen-
sating for
these l'eal-world
erlors is done
duriug tolelancing.
In addition.
rttarty
optical
systenls
degrade somervlrat
with time:
for examl:le. reflectitlg
telescopes
ì-\
Ø44c>\
à"è---
LENS
(sroP)

20 Chapter
4.3
Light and lmaging Systems
nlust occâsionally
be recollirnated.
Itl sotre lalge
and cotnplex systelns.
active op-
tics
can be
used to rnaintain alignrnent.
The
thircl cause of irnperfect
irnages is
difflaction of
ligltt t'ry apertut'e
and
obscuration
edges in the systeni.
Diffraction
is a cotrsequence
of the
wave
nattlre
of liglit
and
is thus impossible
to elirninate.
Difflaction spleads
out the light in
the
irnages of
point
objects and t'ules out
the
possibility of a
pelfect
point
image of
a
point object. In other
rvords, difffaction
creates the
fundametttal
limit to image
quality
beyond
which it is inrpossible
to
go.
In soltle
systetns,
the effects of dif-
fraction
may be smail
relative
to other sources
of inrage
degladation. But
in highly
collected
systerrs,
diffraction
is the r¡ain soulce
of irnage degladation,
and
these
systerns
ale
said
to be diff|action
lilnited.
The
foulth
cause of unsharp
images is atrnospheric
tut'bttlence,
or
what as-
tronomers
call
attnosphelic seeing.
An extreme
case of seeing
is tlie sliinimering
tliat
is apltalent
rvhen
looking
at a distânt object
in the
desert iti
the
sutnlner
heat.
Seeing
is
always
an
importattt
effect for
ground-based telescopes
that attempt
to
achieve
high angular
resolution
while viewing thlough
the dynantic
and inlrorno-
geneous air. Note
that seeing is
lelatecl to bLrt
not the saure
as the scintillation
or
twinkling of
stals. In
very
special
systems,
adaptive optics
can
paltially
relrove
the efTects
of seeing
in real time. Optical
systems
whose itnage
quality
is limited
by
atnrosphel'ic
turbulence are said
to be seeing
limited.
4.3.10
The
Point Spread
Function
Becanse it
is impossible
to image a
point
object
as a mathernatically
perfect
point
image,
the concept of
point
spt'eacl
function, or PSF,
has been
introduced.
The
PSF
gives the
physically cotrect light clistribution
in the ilnage
of a
point ob-
.ject
due to the
efïects of abelrations
and difftaction.
Neither fabrication
and alignrnent
errors
nor atrnospheric seeing
are includ-
ed in a stanclard
PSF
analysis. These erl'ols
are handled separately
because
they
ale of a st¿rtistical
rather
than a detelministic
r-r¿rture. Althougli
these sources
of
ellor are often
important,
the PSF due only
to aben'ations
and difiraction
is very
useful for the
lens designel because
it
provides a rneasttre of
the
best
image
qual-
ity a
nourinal design is
capable of achieving.
Most letts
clesign coutputel
progralns
can
calcul¿rte the
PSF and clisltlay
tlre results either nuurerically
ol
grapliically.
For
a two-dimensional extended
object,
the light distribution
in the
irnage is
derived
nìathelnatically
by a tivo-dirnensional
convolution
of the olrject
(ol
its
pert'ect
irnage)
with the PSF. Inrage
processiug and
Foulier
optics
ale based on
this lelationship,
and they are
career specializations
in their orvn
right.
4.3.11
lmage Motion
Tlie
fif th cause of inrage
quality
degr:adation
is image r.notion.
Iura-qe
utotiott
cau
be
produced
by
a lineal translatic¡n ol
an angulal rotation
ofan optical
systeltl.
Section 4.3.12: Stray Light 21
The
classic example is camera vibration,
althor.rgli thele ale rnany othel sources.
In
addition,
sometirnes
the
object
itself is in motion.
In eithel
case. unless precaLr-
tions
are taken, the
result
is image
snrealing. AlthoLrgh
irnage
motion
is
often
irl-
portant
in
the
design and use of optical systenrs, it is only r:alely a
factol
in lens
design.
A.3.12 Stray
Light
Stlay
light, tlie sixtli cause
of
irnage
quality
degladation, is caused b),
r-'n-
wanted
light scattered and/or ref'lected by optical ancl rrech¿rnical sulfaces
u,ithin
an optical systeln. Stlay light can take two folrns. The f ilst folur
is a loughly uni-
form
veiling glare
across the irnage that reduces contrast. The second
form is
gl-rost
images. Ghost irrrages can also take two forms. The
filst is a complete du-
plicate
irnage, often invelted
ancl
in failly
goocl
focus. The seconcl consists of dis-
crete flare
spots, often highly aberrated, corresponding
to
bright
ligttt
sources
within or close to the fielci of view.
Excessive stlay light
can
disqualify
a potential optical design as readily
as
excessive
aberrations.
Stlay
light
is
a
particularly
difficult
plobleur
for
complex
systems
that either have
rnany lens
surfaces ol that have lalge rlecharnical
distanc-
es between surfaces
(including
the inrage sulface).
It is im¡roltant for the optical
designer to be aware of stlay-light
considerations
fi'om
the beginning of the
cle-
sign
process.
Waiting
until
a design is nearly finished and
then trying to correct
stta¡,-lioþ1
problems is
usually
doomecl to failure.
Since
about 1
940,
there have been two advances
that have revolLrtionized
the
control of stray light. The first
aclvance
is
thin-film anti-r'eflection
lens coatings.
Urrcoated
glass
leflects about 4Vo of the incident light
per
sulface,
with higher'-in-
clex
glasses
reflecting somewhat lnol'e than lower-index
glasses.
Fot a urulti-ele-
ment lens
with r.r.rany air-to-glass
surfaces,
the total of
this
reflected
light cau be
substantial. The big
problem is not
the
loss of ir.r.rage light; the big
problern is the
increase in stray light. This leflected liglit must
go
somewhele,
irncl rnuch of it
in-
variably
encls up on the irnage surface
as
stl'ay
light. A coated
Ieus
surface,
how-
ever, typically reflects less than 1Vo, and often rnuch
less. Thus, stlay
light is
reduced. Coated lenses nrake
plactical rnany corrplex systenrs
that otherwise
would
be
r¡seless.
Tlre second advance is the development of
collrprìtel'
prograllìs that analyze
stray
light,
especially light
reflected by
inteliof mechanical surl'aces
such as baf-
fles.
The results
frorn this capability liave
pointed
the
way towarcl
gt'eat
itnpt'ove-
nrents in high-pelforrrance optical systenrs such
as
olbiting
telescopes.
At the
present time, the nrajol
stray-light
cornputel
prograrns
ale APART,
ASAP,
ancl
GUERAP. Note tlìat these
prograrns
anerlyze
stray light, but they
clo noI clesign
baffles. Baffles must still be designed by hand. Thin-fil¡
coatings
and stray-light
supplession are each caleer specializations in
their
own
ri-ght.
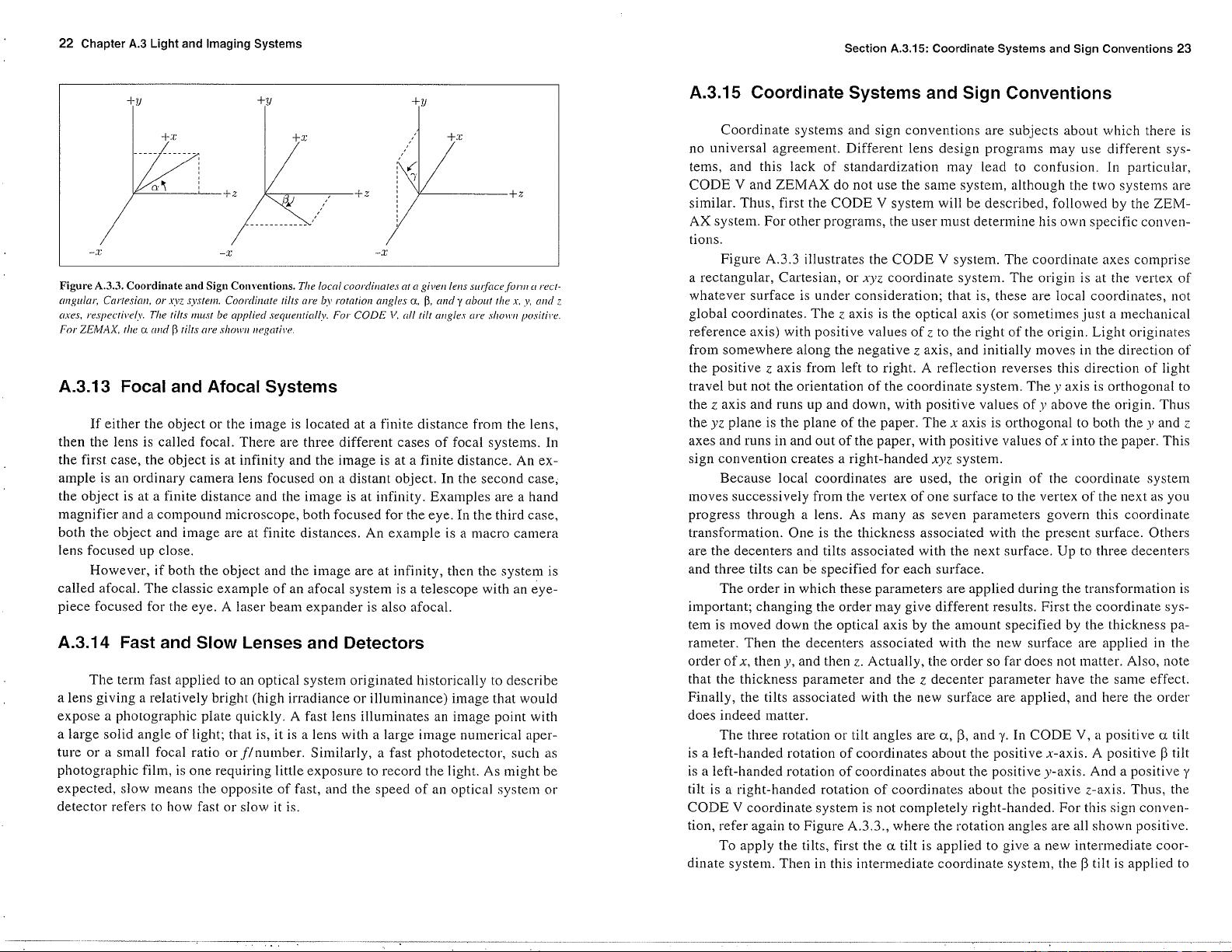
22
Chapter
4.3 Light and lmaging
Systems
Figure 4.3.3. Coordinate and Sign
Convcntio¡rs. The
loctl
coorditLaÍe.\ ot a
given
lens surface.fonn u rect-
trttgular, Carlesicut, ot'.rvt .s¡,.slem.
Coctrditttút
l¡lts are bt' rota¡ion
ttrtl¡las a,
þ,
curcl
'¡
ubotl tltc x,
t',
ttntl
z.
axas,
res¡tectit'elt,. Tlrc tilt.ç
nu.rt be ap¡tlied sequetúiall\J. Far
CODE
\t, all til¡ angla.s are
slto¡'n
ltt¡sitit
c.
For ZEÌvIAX,
¡|rc
a untl
þ
tilts are shovtt n(.ß¿ttit'¿.
4.3.13
Focal and Afocal
Systems
If
either the
object 01'the image is located
at a finite distance from the lens,
then the lens is called
focal. Thele are three cliffelent cases
of
focal
systerns. In
the filst case, the
object is at infinity and the irnage
is
at a
finite distance. An ex-
ample
is
alì ordinary camera lens
focused on a distant object. In the
second
case,
the object is at a
finite distance and the
image is at infinity. Exarnples are a hand
magnifier and a cornpound
micloscope, both focused for the
eye. In the third case,
both the object and irnage ale
at finite dist¿rnces. An exan-rple
is a ntacro car.ìlera
lens focusecl up close.
However, if
both
the
object and the irnage
are at infinity, then the system is
called afocal.
The classic example
of an afocal system is a telescope with an eye-
piece
focused for the
eye. A laser
bear.n
expander
is also afocal.
4.3.14 Fast
and Slow Lenses
and
Detectors
The tenn fast applied
to an optical
systern oliginated historically to clescribe
a
lens
giving
a relatively bright
(high
in'adiance or illuminance)
ilnage that
woulcl
expose a
photographic
piate quickly.
A fast lens
illulninates an iurage
point
with
a large solid angle
of
liglit;
tliat is, it is a lens with a large
image numerical aper'-
tule or a
srnall
focal
ratio or.f/nunrber.
Sirnilarly, a fàst
photodetector,
such
as
plrotoglaphic
film, is
one requiling little exposule to record
tlie light. As rniglit be
expectecl.
slow rneans the
opposite of fast, and the
speed of atl optical systenl or
detecto| r'efèr's to how
fast or slow it is.
Section 4.3.15: Coordinate Systems
and Sign Conventions 23
4.3.15
Coordinate
Systems and
Sign Conventions
Coordinate systems and sign conventions
ale subjects about which
there is
no
universal
agreelnent. Different lens design progranls
may use different sys-
tems,
and ttris
lack of standardization rnay lead
to confusion.
ln
palticular-.
CODE
V and ZEMAX
do not use the salne
systerÌ1,
although the
two
systelÌls
are
sirnilar.
Thns, first the
CODE V system
will
be described,
followecl by the ZEI\4-
AX system. For other
progl'ams,
the Llsel'
must deterrnine his orvn
specif.ic couveu-
tions.
Figure
A.3.3
illustrates the
CODE
V
systern. The
coorclinate
axes cornplise
a rectangular, Cartesian, or x¡,¿ coordinate systeln.
The oligin is at the
vertex
of
whatever surface is under consideration; tliat is,
these al'e local coordinates, l'tot
global cooldinates. The
z
axis is the optical axis
(or
sometimes
just
a urechanical
leference axis) with
positive values
of
¿
to
the right of the origin. Light oliginates
frorn somewhere aiong the negative
z
axis, and initialty
moves ir.r
the
direction of
the
positive
z
axis fi'om left to
right. A reflection reverses this direction of light
travel but not tlìe orientation of the coordir-rate
system.
The
1,¿¡¡i.
is orthogonal to
tlie
¿
axis and runs
up
and
down, with
positive
valnes of
.1,
above the origin. Thus
the
¡r¿
plane
is the
plane
of tl.re paper.
The ¡
axis
is
orthogonal
to botli the
i,
and
-
axes
and
tuns in and out of the
paper,
with
positive
values
of
,r into the
paper.
This
sign
convention
creates a right-handed
x},¿ system.
Because local coordinates
are used, tlie origin of the coordinate systeln
lnoves successively fi'om the vertex
of one surface
to the vertex
o1'the
next as
yor,r
progress
through a lens. As many as
seven
parametels govern
this cooldinate
transfbrmation.
One is the thickness associated
with
the
present
sulface. Others
al'e the decenters and tilts associated rvith the
next
surface.
UD
to three decenters
and thlee tilts can be specified for each surface.
The order in which these parameters are applied duling the transfonnation is
impoltant;
changing
the order rnay
give
different results. First the coordinate sys-
tern is
rnoved
down
the optical axis by the arnount specified by
the thickness
pa-
rameter. Then the decenters associated with tlie new surface are applied in
the
order of x, then
y,
and then
z.
Actually, the
order so
far does not rì1atter. Also, note
that the
thickness
parameter
and the
z
decenter
paraÍneter
have the sarre
effect.
Finally,
the tilts associated with the
new surface
are appliecl, and lrele the order
does indeed
matter.
The three rotation
or
tilt angles are
cx.,
B,
and
y.
In
CODE
V, a
positive
ct tilt
is a
left-handed rotation ofcooldinates about the
positive
¡-axis. A
positive
B
tilt
is a left-handed
rotation of coordinates about the
positive
),-axis.
Ancl
a
positive
1
tilt
is a light-handed
rotatiorì of
coordinates âbout the
positive
¿-axis.
ThLrs, the
CODE V
coordinate
system
is not cornpletely right-l.randed. For this sign conven-
tion,
retèr
again to Figule 4.3.3., whele the rotation angles are all shown
positive.
To apply the tilts,
first the ct tilt is applied
to
give
a new intennediate coor'-
dinate
systeur. Then in this intelnrediate coordiuate systerÌ1,
the
B
tilt is applied to

24 Chapler 4.3
Light and lmaging Systems
give
a second
new intel'ìnecliate
cooldinate systellì.
Lastly, in the second intenne-
diate coordinate system,
the y tilt is applied to
give the final coordinate systenl.
Of
course,
sorne
of
these
seven
pal'anìeters can
be
zero. In
particular,
to
avoicl
confusion, specifying only one nolìz-el'o
tilt angle at
a time is recotnurended
(use
rnultiple single-angle tlanstbnnations
as needed). In
addition, note carefully
that
if
yoLr
wish to re\/erse ol
unclo
a coorclinate
transformatior'ì.
yotì
cannot melely
l'e\/erse the
signs of the
palarnetefs: you
must
also corlpletely
re\/erse the older'
that the
paralneters
are applied. CODE
V lias a command
that allo'"vs this to be
done easily.
In ZEMAX,
coolclinates, clecenters, and
tilts are handled the sarne
r'vay
rvith
three exceptions.
First, in ZEMAX
there is no
¿
decenter;
this function nrust be
hanclled by
the tl.rickness
pararreter.
Secor.td,
a
positive
clt.
tilt is a
rlglrt-lianded
t'o-
tation
about the
positive.r-axis. Ancl third, a
positive
B
tilt is a llglrt-hanclecl
rota-
tion about the
positive
l'-axis.
Thus, the
ZEMA'X system is
cornpletely
right-handed,
and the
cr
and
B
angles shown
in Figure 4.3.3.
now
become
nega-
tive.
There are rnore
sign conventions. If the
lens sut'face is curved,
a positive cur-
vature has the center of
curvature
to
the right
of the surface,
atrd a negative cttr-
vature
has
the center of curvatule
to the left of the surface.
For a lens as a rvhole,
a
positive
lens causes
rays to increase convelgence,
while a negative
leus causes
rays
to decrease convergence
(or
inclease divergence). Convex
lettses are
positive
and concave
lenses ale uegative, bnt
concave mirrors ale
positive.
Still
rnore sign
conventions will be introduced
later'.
Finally,
r'ecall that for lotationally syrlrnetlic,
axially
centered systetns, any
plane
containing the optical
axis is a rneridional
plane, ar.rd all rneridional
planes
are equivalent.
Thus,
rvith
no loss of
generality,
the¡'z rneridional
plane is usttalll,
selected.
and
objects
and images at'e located
along the
),-axis.
4.3.16 Optical
Prescriptions
In
oldel
to be able to enter
a
lens
into a col'r'rputer for optimization
or evalu-
ation, the optical
prescription is lequired. An optical
prescription contaius
all the
inforrration
needed to specify the nominal
lens configuration. Tolelances
are ustì-
ally not included
ancl are hanclled separately.
Because
most lenses consist of collections
of surfaces through
which the
light
¡rasses
seqLrentially, the
nrain palt of a conventional
plescliption is a table
listing the snrf'ace
paramete[s.
The sulfaces
are numbered
and tabulatecl in orclel
stalting with the object sulface
(sulface
0)
ancl ending
with
the irnage surface. A
rninirrurn o1'three sulfaces is required:
the
ob.ject sulface,
the stop sut'face.
atrd
the image sul'face. The sto¡r cannot be on the object ol
irnage surfaces. The
details
of how an optical
prescription is plesented depend very tnuch on
your
particular
lens desi
gn pl'ograril.
Section 4.3.16: Optical Prescript¡ons 25
For eacl-t
sulfãce, the
parameters
incÌude: sulface nnrnber'. raclius
of
culva-
tule,
thickness
to the uext
surface,
glass
type in the next r-neclinln.
any
asplielic
data,
any apertul'es and
obscuratious, arly tilt and clecenter
data, ¿rtrd auy solves.
One
sulf¿ice is designated tlìe
stop surface.
Illstead
of ladius of cuLvatule,
the leciplocal of'r¿rclir.rs
of cnrvature. knolvrr
sirnply
as tl'ìe curvature.
is often substituted.
CnrvatuLe has the acli'antages that a
greatel'absolute value denotes
a
gl'eater
effect and that a flat
surface does not need
to be descl'ibed
with
an infinite
quantity.
However, cLrrvature cloes requile
the
in-
convenient
use
of tiny nuurbel's
(of
course,
(he
coulplrter
cloes uot nrind)
Thickness is vertex
(not
edge) sepal'¿rtior'ì along the optical axis l'rorn
one sul'-
face
to the
next. Glass type can
be
any
optical
glass
as
well
as a crystal,
wittel',
aif,
vacuull1,
and so forth.
A special
glass
clesignation
is
n-rillol-, whel'e
tlre Iight is le-
flected
back into the
plevious
rnedium.
Solves
are specizrl
opelations that acl.just
lens
palarretefs
to satisfy
conditions; solves aLe cliscussecl in
Cliapter
4.13.
A
multiple
configulation
lens
is ¿r special
situation. Hele thele are two or
rnore
discrete lens configulatiol'rs,
with one or tìlore lens
¡talametels
chaneing
be-
tween configurations.
One
of the
most cornulou nrultiple configuration lens types
is a zoom lens. In a zoorn lens, the lens elelnents
lnove longitudinally relative to
each other
to vary the focal
length. But rnultiple configuration lenses are not lirn-
ited to zoorn lenses. Otherexarnples ir-rclude
lenses
witli
scanning rniuors,
rnulti-
ple wavelength
passbands,
beam splittels, and so forth. In
Chapter
8.4 the lens is
given
multiple entrance
pupil
diar.neters.
ln
a
lens
prescription,
the
palameters
that change
betrveen
configurations
are listed separately along with their
values
in each configr-rration. Altelnatively,
more than one lens tallle is
given.
Anothel special
situation is unusual characteristics, sucl'r as sulfaces
u,itlr
difflactive
properties
or
gradierrt
index rnedia. Again, these palametels are often
listed separately.
Three other
pal'alneters
o[
groups
of
parameters
ale also
part
of the optical
prescription.
The first is the
set of wavelengths used. The set nray consist of only
one waveleugth fol'a rnonochromatic or an all-r'eflecting system.
For
a color'-cor'-
Iected refracting
systern, two or rnore wavelengths are necessary. The extferne
wavelengths
define the
wavelength
passband, and usually a centlal rvavelength is
clesignated as the reference, plincipal,
ol
prirnary
w¿rvelength for calculating
first-order
properties.
The second
pararneter gloup
specifies
tlle
positions
of the object
points
in the
field of
view.
Either angulal position
as seen
frorn
the entrallce
pupil
ol
lineal po-
sition on the object surface can I¡e
used. Three ol
foLrr
object
points ale commonly
specified.
One object
point is
usLrally on the optical axis, and one is
usually at the
edge
of the field.
Half-field
angles or distances relative to the optical axis are
giv-
en;
a
full-field
of
40"
corresponds to a half-fielcl of
*20".
For asymnretric or'
off-axis
systems, many object
points
allayed in two climensions across the obiect
surface
rnay be needed.

26 Chapter
4.3 Light and
lmaging Systems
The
third
paratneter
contfols
the diameter
of the on-axis
beam
transrnitted
by
the system.
Mgs.t_9o-B!lolly,
entrauce
pup{9tqT_.tg(ElÐfgspecified.
Alterna-
tively, the
dtg]]]glgl-9|l¡€--ep9llrlre-rrn
the slgplulgg
rarl-Þ9_s!9c-ifieçl,
Another
alternative
is to specify
tbe-Éûlumbg,L.or
nttmerical
aperture of
the irnage.
For fi-
nite
conjugate lenses,
the nutnerical
apertut'e
of the object
is often
used. Other'
variations are also
possible, but the idea
is the sarne.
Finally,
an optical
prescription includes
a title,
any apodization
(nonuniforn
partial transmission
across
the etitrance
pupil), any vignetting
factol's
(a
way of
specifying
the sizes
of off-axis
beams),
any special
controls
for the
program
(such
as ray airning),
and the
units used. Millimeters
are becorning
the
world
standard
for optics;
use inches
if
you rnust; avoid
centimeters.
For
convenience,
when listing
a
prescription,
your
softwale
tlay
append sev-
eral derivecl
quantities.
These
rnay include:
focal length,
focal
ratio, back
focal
length,
overall length,
maxirnnul
irnage
lieight, entlance
and
exit
pu¡til diarnetels
and locations,
clear
apertures,
and so forth.
Be sut'e
to differe¡tiate
between spec-
ified
and delived
quantities.
The
derivecl
quantities are technically
not
part
of the
prescription.
A.3.17
Aspheric
Surfaces
Optical
surfaces
are most often
spherically
shaped, but
general conics,
poly-
nomials,
and
other shapes
can also be used.
For the more
comrnon rotationally
symmetric
surfaces
(including
spheres),
the
departure of
a surface frorn
a
plane'
also
called the sag of
a surface
parallel to the optical
axis, is
given
by:
whele:
lt2
--
x2
+
),2
is the axial height
on the surface
(transvelse
distance
froni
the axis),
c
is the curvatule
of the surface
at its
vettex,
k is
the conic constant of
the sulface,
and
A,
B,
C,
D, ate the
4th-,6th-, 8th-, and
l0ttr-order
polynomial surface
deforma-
tion
coefficients, respectively.
For
the conic constarìt,
I
| 1 a t)
I
+
| I
-
(l
+ klc'h'l-
LJ
Section
4.3.18: Thin Lenses 27
k
=
-1
gives
a
paraboloid,
k
<
-1
gives
a liyperboloid,
and
k
>
0
gives
an oblate ellipsoid
(like
a dool knob or the top o{'a spinning
planet.
such
as the Earth
ol
especially Jupiter).
Note
that
by
definition, arz_r' surface that
is not a
pute
sphere
is arr asphet'e.
As
pleviously mentioned, a
plane
surface
is considered a sphere
rvith
an infinite
ladius of
curvature. Spheles are
distinguished from all othel sulface shapes
be-
cause
spheres
(and
flats) are the only surfaces
that can be tnade relatively easily
in the optical shop.
Any asphere is hard
to make.
4.3.18
Thin Lenses
Fol a real singlet
lens with a nouzero
thickness, the
palaxial
fbcal
length is
.
(.
,.ì
|
, ,,1
I I
lt1
-tltl
j=rtt-,,[[-;-,\\)
where/is
the focal length,
rr
is the
lefractive index of
the
glass,
r, atrd rr al'e
respec-
tively
the front
and
lear ladii
of curvature, and ¡
is the axial thickness.
If the thickness
of the lens
is
small
relative to its other
dimensions, then
this equation reduces
to the
lensrnaker's equation :
,)
ch-
+ Aha +
Bh6
+
ChB
+ Dltto
(4.3.2)
where
¿ is the conic
eccentlicity ft'orl] analytical
geouretry, ancl:
k=0givesasphere,
-l
<
k
<
0
gives
a
prolate ellipsoid
(like
the
pointecl end of
a football),
,2
The
concept of a thin lens is
encounteled again
ancl agaiti in lens
design.
A
thin lens has snch snrall
culvatures and centel
and edge thicknesses
that
all of its
refractive
power
is effectively concentrated
in a
plane.
Of
course, truly
thin lenses
do
not exist in the real
wor-ld, and the concept
is an approxirnaticln.
Nevertheless,
for rnany
applications, the errors
are srnall and
can
be
neglected,
at least initially.
Note that at the
center
of
a thin lens,
the two lens surfaces
ar-e
parallel to each
othel and are effectively
in contact. Thus,
a ray
passing through the centel
o1'a
thin
lens is
uncleviated.
Many useful
optical formulas
are thin-lens fo¡:uiulas.
Perhaps
tlìe most use-
ful is the thin-lens
eouation:
\=
r,-r)lr-11
./ \/'r
12)
rvhere/is the focal length. s is the object
distauce,
anci
sign conventions
ale as
given
above.
Note that the focal
length of
a
tliin
lens is equal
to the iniage
when viewing a distant object.
Itl
-
=
--+-
.f
.r .r'
(A.3.3)
,ç' is the
irnage distancc.
The
to the distance
frorn the
lelis
剩余135页未读,继续阅读
点击了解资源详情
点击了解资源详情
158 浏览量
2008-11-14 上传
2018-02-26 上传
2009-05-23 上传
2018-07-30 上传
shengdacheng
- 粉丝: 3
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

最新资源
- 小型宽带微带天线设计与进展
- QTP 8.0 中文教程:自动化测试与脚本操作详解
- OPC UA基础解析 - 概述与概念RC中文版
- Proteus入门教程:无需实验板的51单片机仿真指南
- Java面试必备:核心知识点详解
- 万方视景科技:虚拟现实内容与项目专家
- Dialogic CTI技术入门到精通:系统工程师指南
- OBJ文件详解:格式、特点与基本结构
- ntop简易安装教程:快速部署流量监控
- Oracle初始化参数深度解析
- WebSphere MQ for z/OS 消息与代码手册
- JFreeChart 1.0.9 开发指南:免费资源与付费版本对比
- 使用Java与WebSphereMQ v6.0交互
- Win32下MinGW与MSYS安装指南
- Linux软件安装指南:从新手到高手
- ADO技术详解:高效数据访问接口
安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功