7012 IEEE TRANSACTIONS ON VEHICULAR TECHNOLOGY, VOL. 65, NO. 9, SEPTEMBER 2016
time required by the RN for broadcasting I
3
[k] to both User
1 and 2 v ia the DL of the BD-DF-FDN is regarded as the DL
period. Finally, the time required for completing a pair of UL
and DL periods is regarded as a complete BD-DF-FDN period.
Naturally, the BD-DF-FDN period is equal to max [UL period,
DL period].
The p ath-loss reduction gain (PLRG) achieved by the re-
duced transmission distance experienced in cooperative sys-
tems is introduced next. As detailed in [28], the average PLRGs
of the User-1-to-RN link and of the User-2-to-RN link are
given by G
1
=(D/D
1
)
α
and G
2
=(D/D
2
)
α
, respectively,
where D, D
1
,D
2
are the distances from User 1 to User 2, from
User 1 to the RN, and from User 2 to the RN, respectively.
Throughout this paper, the p ath-loss exponent is fixed to α = 4,
for representing a typical urban area. In practice, the d irect
link b etween User 1 and User 2 of our system may become
weak, while simultaneously being interfered by the strong
contaminating signal o f the RN. Hence, similar to [21] and [22],
it may be reasonable to ignore the signal received via this
direct link in Fig. 1. Then, all the possible propagation paths
in our BD-DF-FDN are assumed to be the flat block-fading
Rayleigh channels, where the fading coefficient of a channel
remains constant over a block period but fluctuates in a flat
independent Rayleigh fading manner among different blocks. It
is also assumed that they are reciprocal channels, which means
that the channel from User 1 to the RN is identical to that
from the RN to User 1 during the same period. Furthermore,
we assumed that a BD-DF-FDN period happens to overlap a
block period of the associated channels. Finally, we do not
consider any sophisticated power allocation scheme in this
paper. We equitably share the entire power among User 1,
User 2, and the RN, i.e., we have P
1
= P
2
= P
3
= P ,where
P
1
,P
2
,P
3
is the transmit power of User 1, User 2, and the RN,
respectively.
Based on these assumptions, the signal received at the RN
within the transmission of a specific information frame is given
by y
3
= h
1
√
G
1
S
1
+ h
2
√
G
2
S
2
+ h
3
S
3
+ n
3
,whereh
1
and
h
2
are the fading coefficients of the User-1-to-RN link and of
the User-2-to-RN link, respectively, while S
1
,S
2
,S
3
represent
the symbols transmitted by User 1, User 2, and the RN, respec-
tively. Finally, n
3
is th e additive white Gaussian noise (AWGN)
imposed on the RN, which obeys n
3
∼CN(0,σ
2
). Specifi-
cally, the signal component h
3
S
3
captures the SI imposed on
the RN, as shown in Fig. 1, where h
3
may be regarded as the
attenuation of the SI channel. After implementing the SI can-
celation, the residual SI becomes
˜
h
3
S
3
, owing to a potentially
imperfect cancelation process. Let us define the SI suppression
factor as G
SI
= 1/|
˜
h
3
|
2
, which is inversely proportional to the
power of the residual SI. Consequently, after SI cancelation, the
received signal y
3
may be modified to
y
3
= h
1
G
1
S
1
+ h
2
G
2
S
2
+
˜
h
3
S
3
+ n
3
. (2)
III. C
ONVEX REGION OF (R
1
+ R
2
)
Based on th e system model built in Section II, particularly on
the physical concepts introduced in Section II, we now define
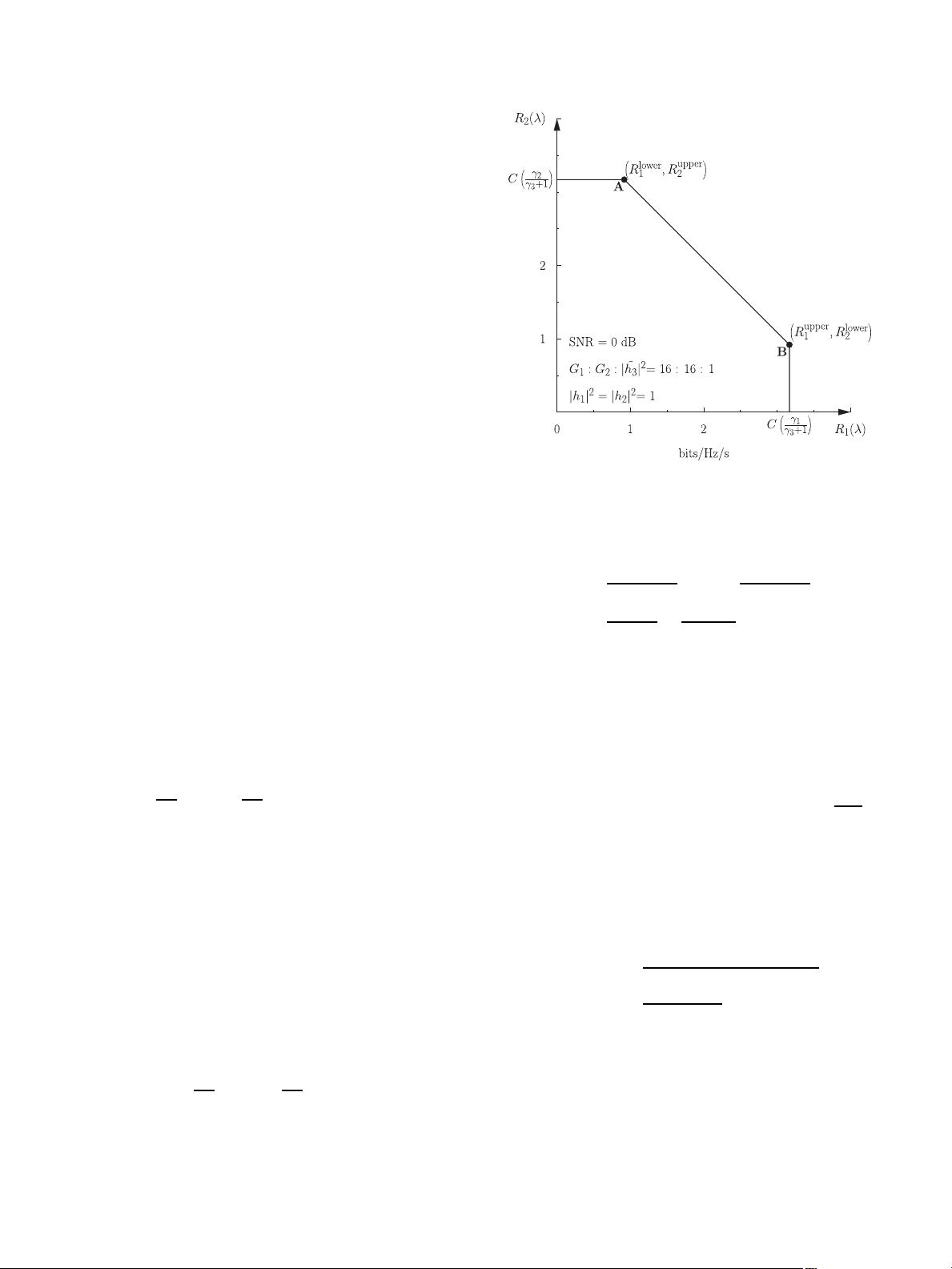
Fig. 2. Convex region of the rate pair (R
1
+ R
2
), where a scenario having
“SNR = 0dB;G
1
: G
2
: |
˜
h
3
|
2
= 16 : 16 : 1; |h
1
|
2
= |h
2
|
2
= 1” is consid-
ered as an example.
the relevant SNRs as f ollows:
γ
1
=
|h
1
|
2
G
1
P
1
σ
2
,γ
2
=
|h
2
|
2
G
2
P
2
σ
2
γ
3
=
|
˜
h
3
|
2
P
3
σ
2
=
P
3
σ
2
· G
SI
. (3)
Without loss of generality, we may assume that
3
γ
2
≥ γ
1
.
Since the RN in Fig. 1 relies on the DF protocol, we have
to carefully avoid the error p ropagation problem. Hence, the
transmission rates R
1
and R
2
have to be specifically chosen
to ensure that the information frames I
1
[k] and I
2
[k] can be
perfectly d ecoded at the RN. According to the multiple-access
channel capacity theorem in [29], these rate pairs (R
1
,R
2
) have
to lie within th e convex region shown in Fig. 2. Furthermore,
the rate pairs (R
1
,R
2
) distributed along the segment AB will
result in the maximum sum rate of (R
1
+ R
2
).
In more d etail, considering the UL in Fig. 1, if the RN
first decodes the information frame I
1
[k], it may regard the
information frame I
2
[k] as a contamination. Hence, according
to (2), the overall signal-to-inference-plus-noise power ratio
(SINR) of the User-1-to-RN link is given by
SINR
1→3
=
|h
1
|
2
G
1
P
1
|h
2
|
2
G
2
P
2
+ |
˜
h
3
|
2
P
3
+ σ
2
=
γ
1
γ
2
+ γ
3
+ 1
. (4)
In this case, the associated capacity of the User-1-to-RN link
may be formulated as
4
C(γ
1
/(γ
2
+ γ
3
+ 1)), which is also the
lower bound of R
1
, n amely, R
lower
1
, when simultaneously satis-
fying th e flawless decodability of information frames received
at the RN, while sim ultaneously attaining the maximu m sum
rate of (R
1
+ R
2
).
3
This implies that the higher one between γ
1
and γ
2
is always represented
by the label “γ
2
.”
4
It is exploited herein that C(x)=log
2
(1 + x).









