TL431/TL432
ADJUSTABLE PRECISION SHUNT REGULATOR
TL431/432
Document number: DS35050 Rev. 2 - 2
3 of 14
www.diodes.com
September 2010
© Diodes Incorporated
NEW PRODUCT
Electrical Characteristics (T
A
= +25°C, unless otherwise noted)
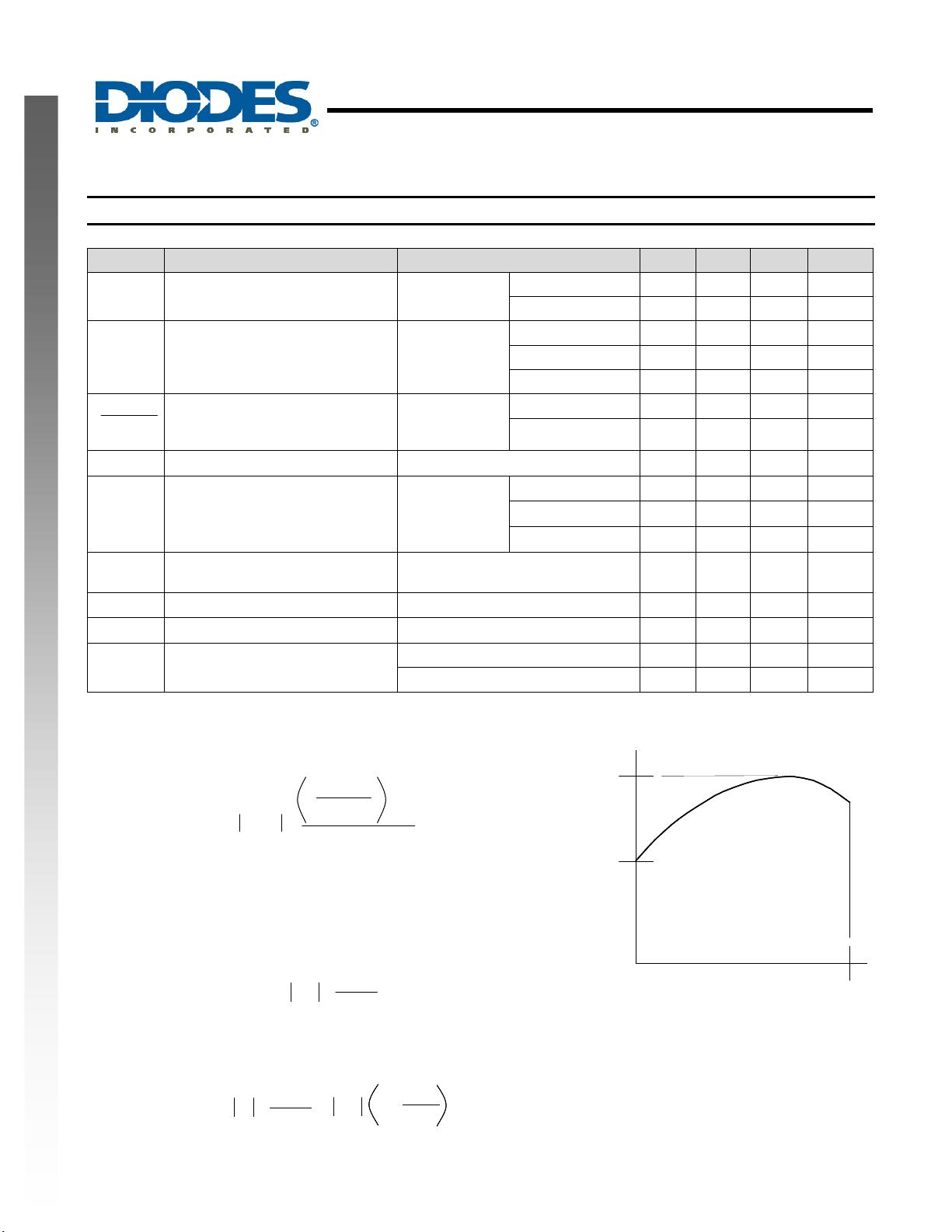
Symbol Parameter Test Conditions Min Typ. Max Unit
V
REF
Reference voltage
V
KA
= V
REF
,
I
KA
= 10mA
TL431A 2.470 2.495 2.520 V
TL431B 2.482 2.495 2.507 V
V
DEV
Deviation of reference voltage over
full temperature range (Note 5)
V
KA
= V
REF
,
I
KA
= 10mA
T
A
= 0 to 70
o
C 6 16 mV
T
A
= -40 to 85
o
C 14 34 mV
T
A
= -40 to 125
o
C 14 34 mV
ΔV
REF
ΔV
KA
Ratio of the change in reference
voltage to the change in cathode
voltage
I
KA
= 10mA
V
KA
= 10V to V
REF
-1.4 -2.7 mV/V
V
KA
= 36V to 10V -1 -2 mV/V
I
REF
Reference input current I
KA
= 10mA, R1 = 10KΩ, R2 = ∞ 1 4
μA
ΔI
REF
I
REF
deviation over full temperature
range (Note 5)
I
KA
= 10mA, R1 =
10KΩ, R2 = ∞
T
A
= 0 to 70
o
C 0.8 1.2
μA
T
A
= -40 to 85
o
C 0.8 2.5
μA
T
A
= -40 to 125
o
C 0.8 2.5
μA
I
KA(MIN)
Minimum cathode current for
regulation
V
KA
= V
REF
0.4 0.7 mA
I
KA(OFF)
Off-state current V
KA
= 36V, V
REF
= 0V 0.05 0.5
μA
|Z
KA
|
Dynamic output impedance (Note 6) V
KA
= V
REF,
f = 0Hz 0.2 0.5 Ω
θ
JA
Thermal Resistance Junction to
Ambient
SOT23 380
o
C/W
SOT25 250
o
C/W
Notes: 5. Deviation of V
DEV
, and ΔI
REF
are defined as the maximum variation of the values over the full temperature range.
The average temperature coefficient of the reference input voltage αV
REF
is defined as:
Where:
T2 – T1 = full temperature change.
αV
REF can be positive or negative depending on whether the slope is
positive or negative.
Notes: 6. The dynamic output impedance, R
Z, is defined as:
When the device is programmed with two external resistors R1 and R2, the dynamic output
impedance of the overall circuit, is defined as:
=
T2 – T1
αV
REF
V
DEV
V
REF
@ 25ºC
X 10
6
ppm/ºC
=
T2 – T1
αV
REF
V
DEV
V
REF
@ 25ºC
X 10
6
ppm/ºC
Vmax
Vmin
T1 T2
V
DEV
= Vmax - Vmin
Temperature
Vmax
Vmin
T1 T2
V
DEV
= Vmax - Vmin
Temperature
=
Z
KA
ΔV
KA
ΔI
KA
=
Z
KA
ΔV
KA
ΔI
KA
=
Z’
ΔV
ΔI
Z
KA
1 +
R1
R2
≈
=
Z’
ΔV
ΔI
=
Z’
ΔV
ΔI
Z
KA
1 +
R1
R2
Z
KA
1 +
R1
R2
≈









