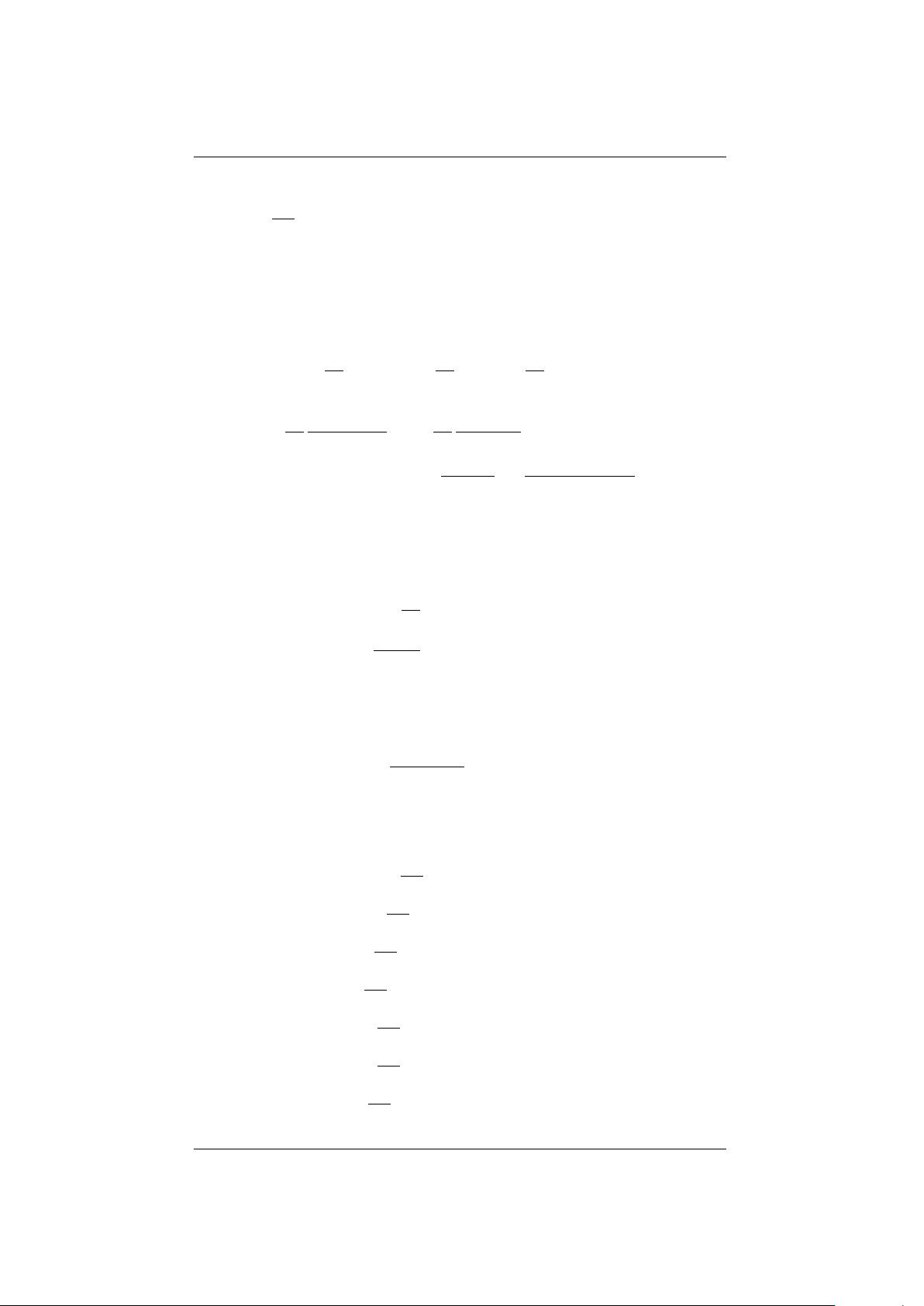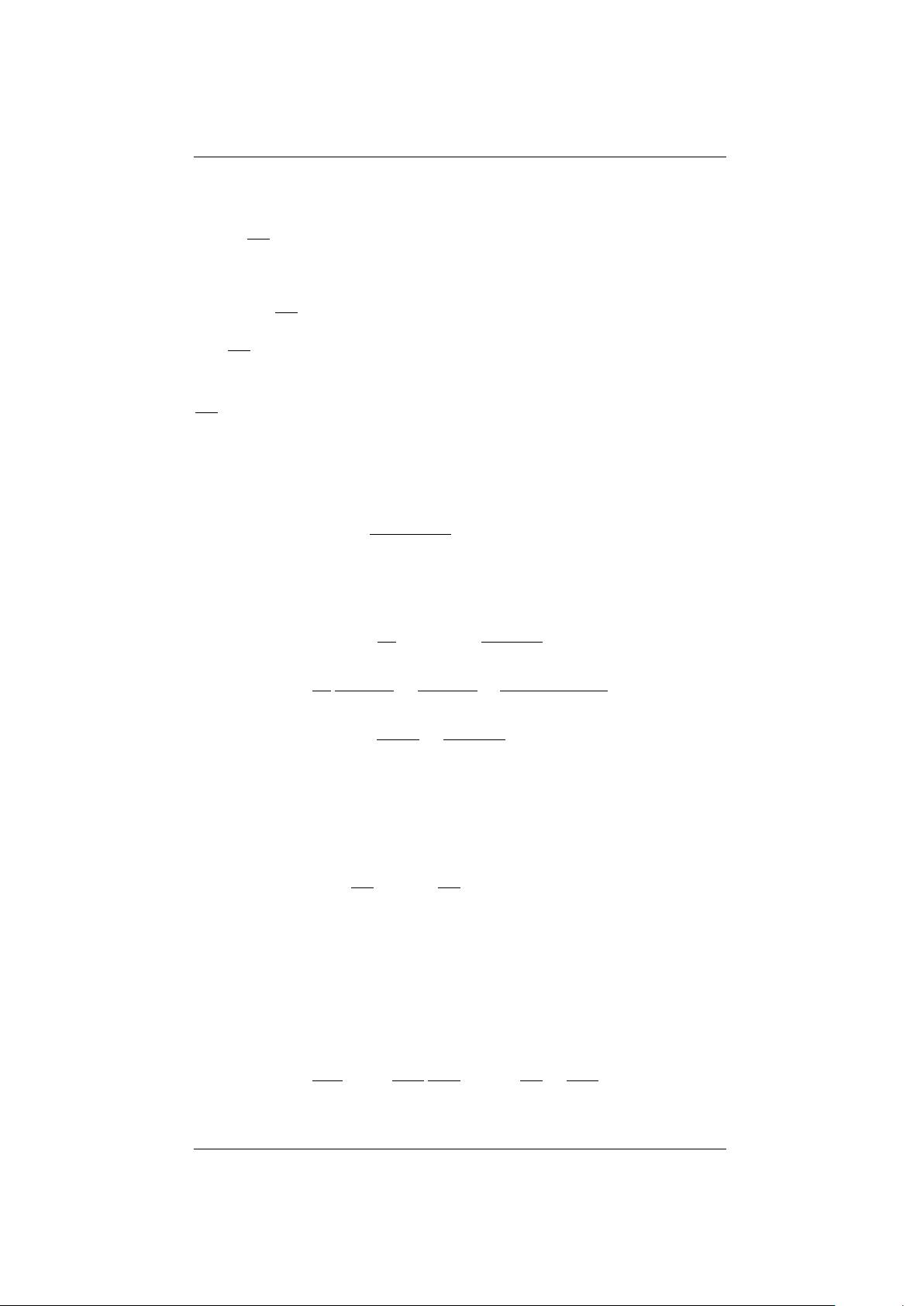矩阵宝典：快速参考的矩阵计算与技巧

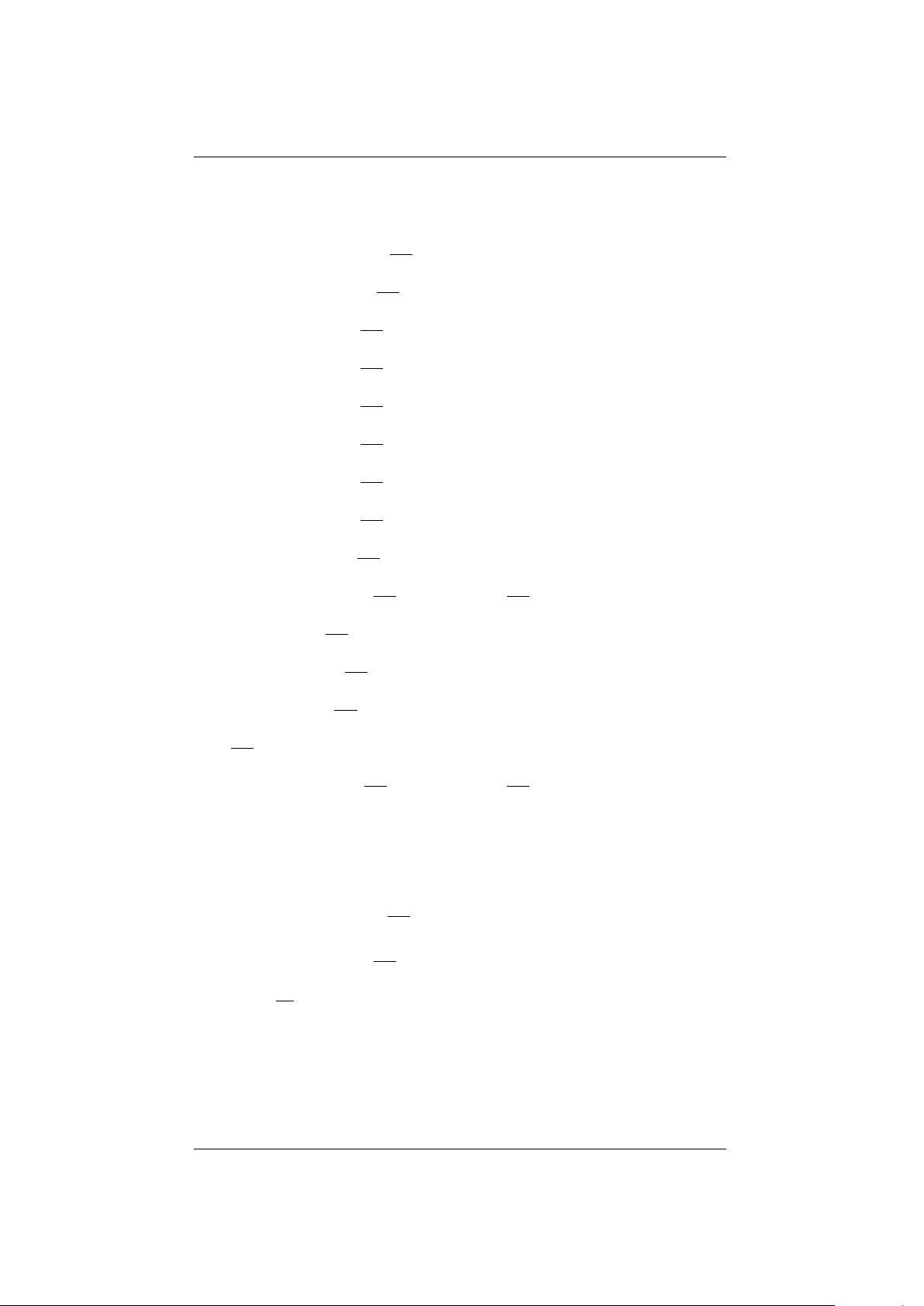
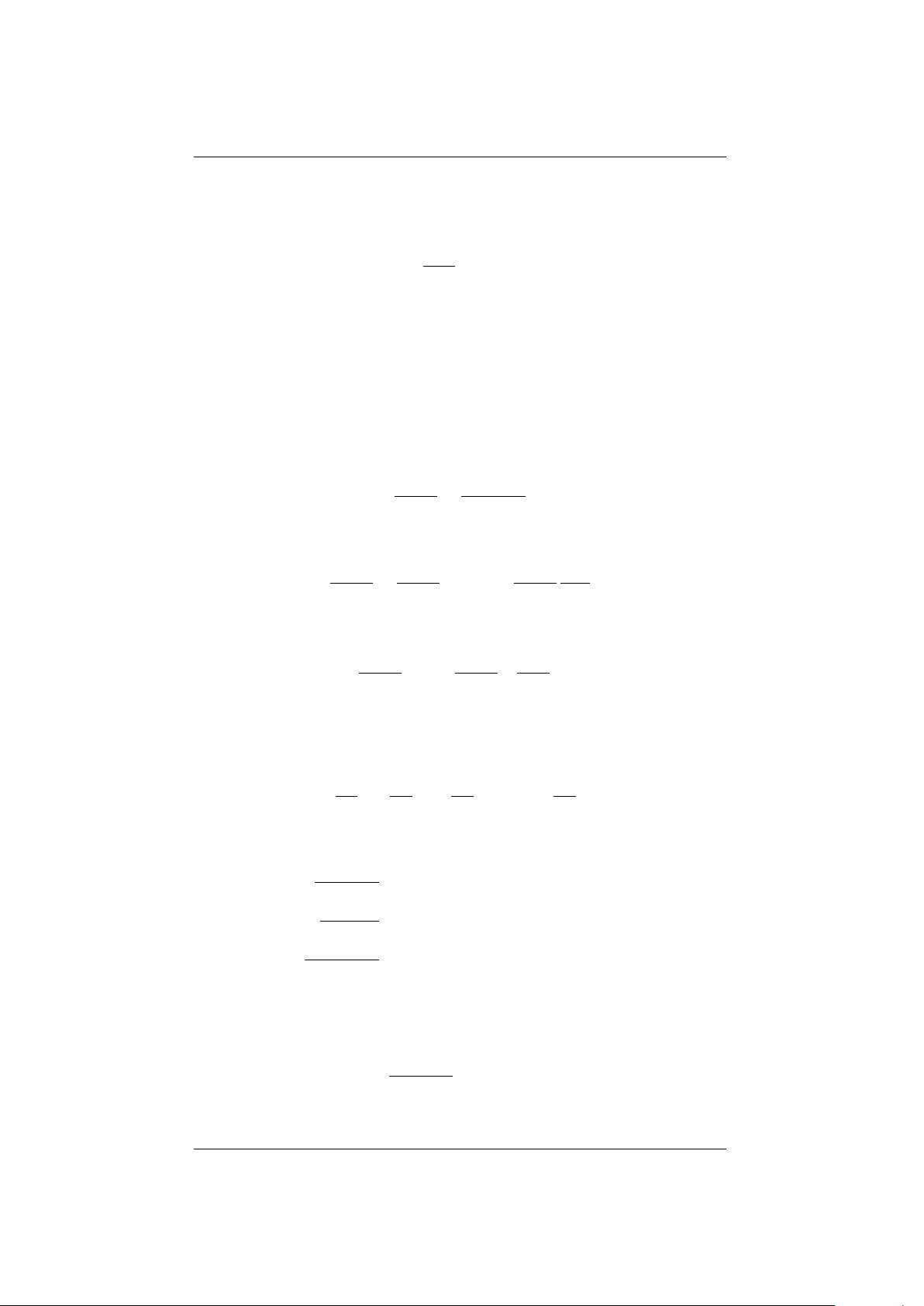
"The Matrix Cookbook" 是一本专门针对矩阵数学领域的参考书籍，由 Kaare Brandt Petersen 和 Michael Syskind Pedersen 编撰，于 2012 年 11 月更新。该书的目的是为需要快速查阅矩阵相关知识的读者提供一个方便的桌面工具。书中包含了各种关于矩阵的定理、近似公式、不等式以及它们之间的关系，这些内容并非原创，而是从众多来源汇总而来，包括互联网上的笔记和书籍附录，作者对于可能存在的错误和遗漏表示歉意，并鼓励读者通过 cookbook@2302.dk 提供纠正和建议。 这本书涵盖了广泛的矩阵知识，例如矩阵的导数计算，这在许多科学和工程领域中是不可或缺的工具，尤其是在机器学习、深度学习、线性代数以及控制系统中。它可能包括对矩阵乘法的性质（如分配律、结合律）、矩阵的特征值和特征向量、行列式的性质、矩阵求逆、迹（Trace）和迹展开、矩阵的谱分解、以及与矩阵运算相关的特殊矩阵（如对角矩阵、单位矩阵、奇异值分解等）的处理方法。 此外，书中可能还涉及矩阵的迹和迹的运算、矩阵积分、矩阵的迹展开技巧、矩阵的指数和对数形式、矩阵的傅里叶变换、以及矩阵的近似算法（如QR分解、SVD等）。由于矩阵在很多高级数学和工程问题中扮演核心角色，这本书对于研究者、教师和工程师来说是一本极具价值的参考资料。 尽管版本不断更新，但作者也提醒读者，书中可能存在尚未发现的错误和不完整之处，所以持续关注最新版本是非常重要的。如果你在阅读过程中遇到新发现或想要深入探讨的主题，可以通过邮件向作者提出建议，共同丰富和完善这个矩阵知识宝库。 "The Matrix Cookbook" 是一个实用且全面的矩阵数学参考资源，适合任何希望深入了解和运用矩阵理论的人群，无论是进行深入研究还是日常工作中需要快速查询，都是一种非常有价值的辅助工具。