M
gain
10
0 q
2
gain
; (4)
where q
gain
2n∕n
2
1 is the transmission coefficient of
the TE-polarized electric field at Brewster’s angle for the gain
medium, and n is the refractive index (e.g., for Cr:ZnSe, refrac-
tive index around 2.5 μm is 2.44, Brewster’s angle is 67.7°, and
q
znse
0.71). For the Brewster’s angle inserted BRF, the Jones
matrix can be written as [2,5]
M
BRF
Cos
2
θSin
2
θExpjΔϕ q
brf
CosθSinθExpjΔϕ − 1
q
brf
CosθSin θExpjΔϕ − 1 q
2
brf
Cos
2
θSin
2
θExpjΔϕ
!
: (5)
In (5), q
brf
is the transmission coefficient of the TE-
polarized electric field at Brewster’s angle (for magnesium fluo-
ride, the refractive index is 1.37, Brewster’s angle is 53.9°,
q
brf
0.916), and Δϕ is the phase retardation of the plate
and can be calculated using
Δϕ
2π
λ
t
Cosβ
n
e
− n
o
Sin
2
γ
2π
λ
tΔn
Cosβ
Sin
2
γ: (6)
In Eq. ( 6), n
o
and n
e
are the ordinary and extraordinary in-
dices of refraction for the BRF material, and for magnesium
fluoride, a Sell Meier type dispersion equation given in
Table 1 of [16] has been used for calculation of the wavelength
dependence of birefringence Δn n
e
−n
o
. The angles θ and γ
appearing in Eqs. (5) and (6) can be calculated in terms of the
other more accessible angles using
CosγCosβCosσSinβSinσCosρ; (7)
Cosθ−
SinσSinρ
Sinγ
: (8)
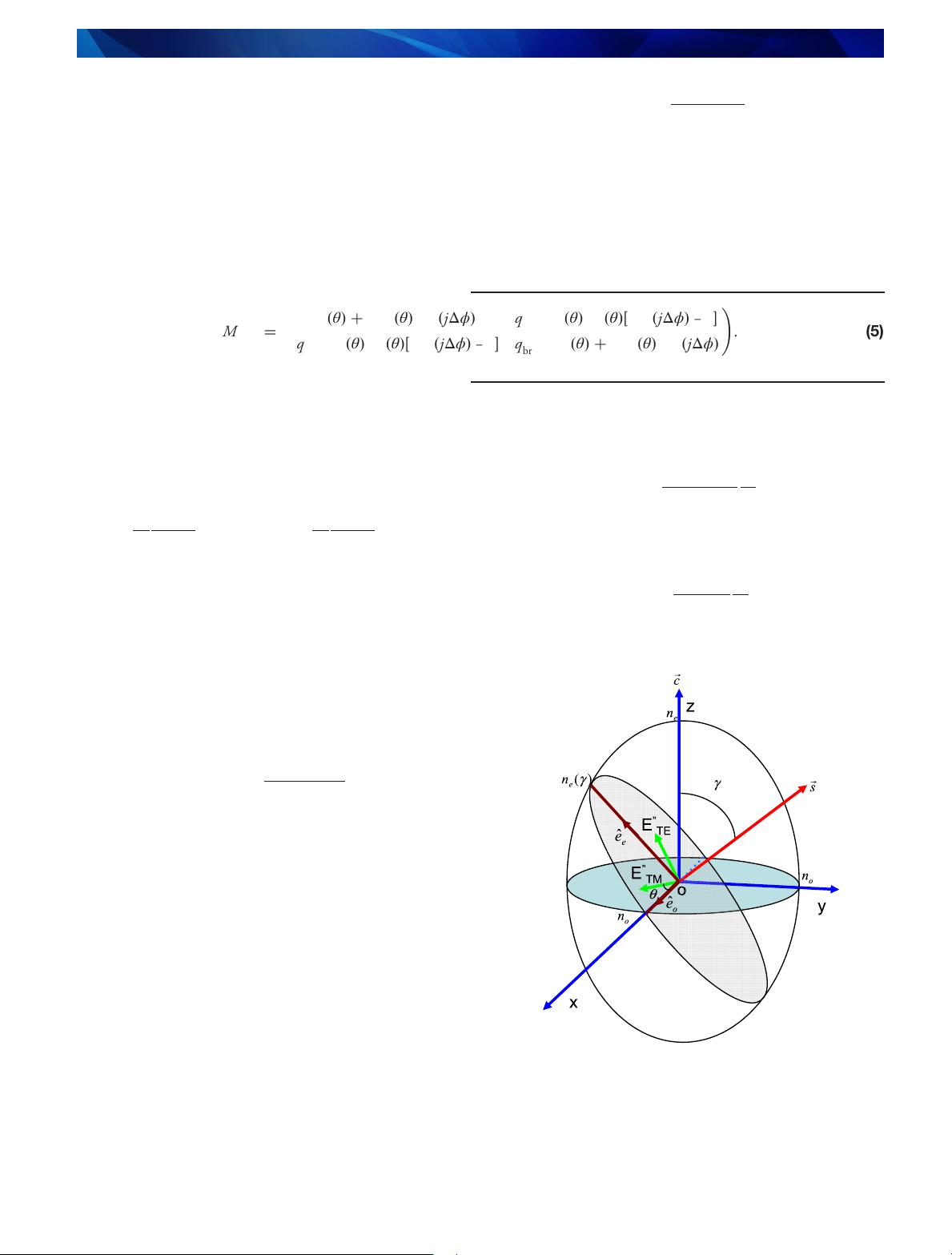
Figure 2 depicts the situation using an index ellipsoid for
better visibility. Note that γ is the angle between the optic axis
of the birefringent cr ystal and the beam propagation direction
[angle between ~c and ~s, as shown in Fig. 1(b) also], and θ is the
angle between the TM-polarized part of the electric field (E
00
TM
)
of the incident light beam and the ordinary refractive axis of the
birefringent plate (ˆe
o
). We note here that there is a mistake in
the stated equation for the calculation of θ in [2], and the cor-
rect form is given in Eq. (8).
While using the BRF inside the laser resonator, the laser
wavelengths that satisfy
Δϕ m2π; (9)
relation will not be effected by the BRF (will see it as a full-wave
plate). For our specific cavity described then, the solution of
Eq. (1) shows that at these wavelengths the filter transmission
is unity, and the polarization eigenmode has only the TM com-
ponent. The wavelengths at which Eq. (9) holds can be
calculated using [5]
λ
m
tΔn Sin
2
γ
m Cosβ
: (10)
When the wavelength is λ
m
(m
th
resonance wavelength), the
polarization is TM at all the interfaces, and the beam will not
observe any loss. On the other hand, other wavelengths on both
sides of λ
m
will have elliptic polarization and will observe loss
due to their TE component of the electric field.
Tuning of the laser wavelength is facilitated by rotation of
the plate about an axis normal to the surface (corresponds to
changing ρ, which will change γ and hence the peak transmis-
sion wavelength λ
m
). Hence, the TR could be expressed as
(dλ
m
∕dρ)
TR
tΔn Sin2γ
m Cosβ
∂γ
∂ρ
; (11)
and using Eq. (10) can be re-written as
TR
λ Sin2γ
Sin
2
2γ
∂γ
∂ρ
: (12)
Fig. 2. Index ellipsoid of the magnesium fluoride crystal that poss-
eses a positive uniaxial birefringence [2]. Projection of the incident
electric field onto the crystallographic axis of the filter is also
shown. n
o
: ordinary refractive index, n
e
: extraordinary refractive index,
n
e
γ: refractive index observed by the extraordinary wave in kDB
formalism, ~c: optic axis, ~s: direction of beam propagation, γ: angle
between ~c and ~s.
Research Article
Vol. 56, No. 28 / October 1 2017 / Applied Optics 7817









