Z. Falomir et al. / Expert Systems With Applications 97 (2018) 83–94 85
Fig. 1. Diagram for describing QCD: discretization of the HSL color space.
In order to determine the interval of values associated to the
Qualitative Color Reference System (QCRS), a test was carried out
on 534 participants (students and teachers) at Universitat Jaume
I and Universidad de Sevilla in Spain. A computer application
was implemented ( Falomir et al., 2015a; Sanz et al., 2015 ) which
showed 10 different colors selected randomly and uniformly using
their HSL coordinates. For each color selected, participants were
asked if they considered the color to be in the grey or rainbow
scale. For those colors classified in the grey scale, participants were
asked if the color was white, light_grey, grey, dark_grey or black ,
that is, card (QC
NAME
1
) = 5 . For those colors classified in the rain-
bow scale, participants were asked if the color was red, orange, yel-
low, green, turquoise, blue, purple or pink , that is, card(QC
NAME
2 .. 5
) =
8 , and if it was light, pale or dark . Thus, a total of 37 color names
were considered.
Let us justify the parameters selected: (i) card(QC
NAME
1
) = 5 be-
cause the less saturated and extreme colors in luminance are white
and black and, according to the M sets defined, there are two more
gradations in lightness light- and dark- and one more in saturation
pale- , which correspond to light-grey, dark-grey , and grey , respec-
tively; and (ii) card(QC
NAME
2 .. 5
) = 8 since the rainbow/spectral col-
ors are 7 and the majority of the participants of the test suggested
to add also pink
2
.
The QCRS was calibrated according to the results obtained from
those studies ( Falomir et al., 2015a; Sanz et al., 2015 ). The selected
QC
INT
are given in Table 1 showing the HSL values assigned to each
color name.
It is possible to obtain tailored parameterizations of the QCD
theory, with different thresholds and/or sets of adjectives. An ex-
ample of another experimentally validated parameterization for a
specific user community (including less color experts) is provided
by Sanz et al. (2015) . The main differences with respect to the pre-
vious QCD parameterization are (i) slightly different hue intervals
(ii) a smaller threshold between the gray scale and the chromatic
scale and (iii) the pale- and light- modifiers are merged into a sin-
gle palelight adjective. This alternative parameterization is specified
in Table 2 .
2
Note that the selected values for the cardinalities of the sets depend on the
current use case and that different values of those parameters could have produced
different outcomes in the survey.
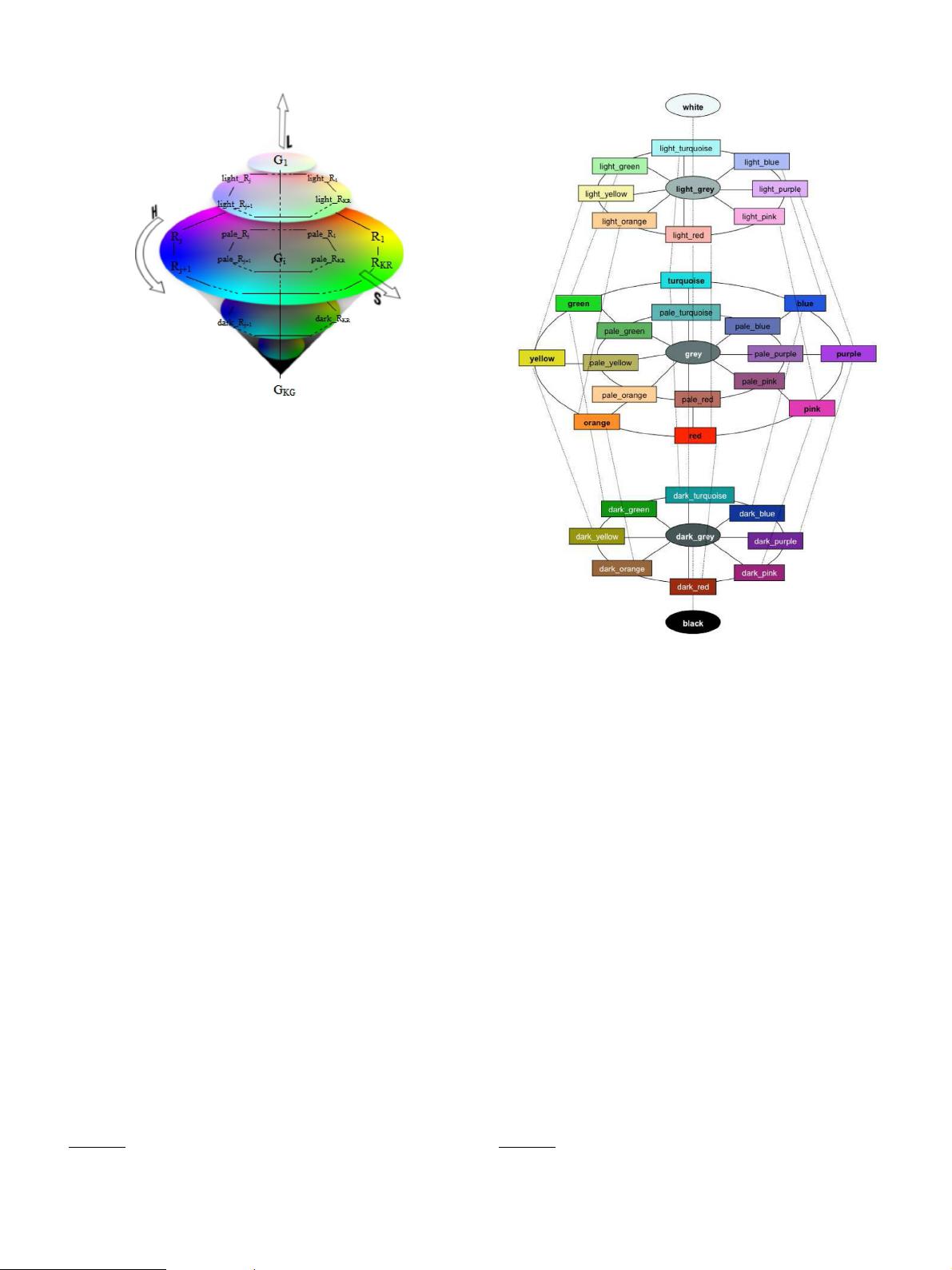
Fig. 2. Diagram for describing QCD: CND corresponding to QCD, w
i
correspond to
the weights used to calculate the similarity between colors.
3.
A similarity measure between paintings using QCD
The QCD model has a relational structure since it is build on
a 3-dimensional color space where the luminosity, saturation and
hue of the colors change continuously. The colors defined by the
QCD model can be organized in a Conceptual Neighborhood Di-
agram (CND) ( Freksa, 2013 ) according to how one can be trans-
formed into another by changing its luminosity, saturation or hue.
For example, the colors red and orange are conceptual neighbors
since a continuous change in hue causes a direct transition from
red to orange . However, the colors blue and red are not conceptual
neighbors since a continuous transformation of hue from blue to
red finds other colors in between. A CND for the computational
QCD model has been built for the original parameterization (37
colors) and it is shown in Fig. 2 . A similar CND can be built for
the adapted parameterization. The nodes of this CND correspond
to the color names, where the path connecting neighboring colors
are drawn as lines. In order to calculate the similarity between the
color names in the QCD, an interval distance as defined below has
been used, since it can be calculated for any of the used parame-
terizations ( Falomir et al., 2013 ).
Given two intervals
3
, I
1
= (a
1
,b
1
) = B
r1
(c
1
) and I
2
= (a
2
,b
2
)
= B
r2
(c
2
) , a family of distances between them was defined in
Gonzalez-Abril, Velasco, Ortega, and Cuberos (2009) , which de-
3
An interval is written indistinctly in the classic notation, I =
(a, b) or in the
Borelian notation, B
r
( c ) (open ball), that is, I =
(a, b) = (c − r, c + r) , where c = (a +
b
) / 2 and r = (b − a ) / 2 . If c ∈ R
2
, then B
r
( c ) represent an open ball in R
2
.









