COL 11(11), 113301(2013) CHINESE OPTICS LETTERS November 10, 2013
Robust estimation of spectral reflectance by a
projector-camera sys t em
Yuqi Li (
ooo
üüü
;;;
)
1∗
, Dongming Lu (
°°°
ÀÀÀ
²²²
)
1
, and Lei Zhao (
ëëë
[[[
)
1
College of Computer Science and Technology, Zhejiang University, Hangzhou 310027, China
∗
Corresponding author: fenghuoqilin@gmail.com
Received July 11, 2013; accepted S eptember 16, 2013; posted online November 4, 2013
A Projector-camera (Procam) system is an inexpensive, household, controllable system that can be used
to eliminate inter-reflection existing in the measurement. We propose an estimation method for spectral
reflectance that uses the Procam system. The method recovers reflectance from the training set constructed
by a known reflectance and the corresponding 9D color-mixing matrix. Experiment results show that our
method performs well with 9D response, and the local weighted training set b ased on Mahalanobis metric
can enhance the accuracy of result efficiently.
OCIS codes: 330.1690, 330.7310.
doi: 10.3788/COL201311.113301.
Estimating the spectral reflectance of objects or sce nes
in visible wavelengths is useful in numerous vision
tasks such as material recognition, relighting, multispec-
tral projection display, etc. Although using a spec-
trophotometer and the illumination sequences of spe-
cific wavelengths is a precise way to recover spectral
reflectance, it is not practical since the laboratory in-
struments are expensive and require professional train-
ing. Hence, methods that use multi-spectral imaging
systems have been proposed as a substitute to using
sp e ctrophotometers
[1−4]
. These methods can es timate
the spectr al reflectance from the training samples with-
out known a illumination spectrum and spectral sensi-
tivity. The training samples are constructed w ith abun-
dant spectral reflectance a nd the corresponding camera
response. However, these multi-spectral imaging tech-
niques cannot deal with concave objects in the presence
of inter-reflection beca use inter-reflection that exists in
the measurement may cause inac curate results.
Benefit fr om encoded patterns techniques
[5]
of the
Projector-camera (Procam) system, we can separate di-
rect and indirect light rapidly which cannot be realized
with other controllable light sources. The system that
combines controllable projectors with cameras is popular
in a wide range of applications, such as three-dimensiona l
(3D) scanning, flexible display walls
[6]
, light field acqui-
sition, a nd interaction. Han et al .
[7]
used a digital light
processing (DLP) projector and a camera to re c over re-
flectance, but the method requires the spectral sensitivity
of camer a to be known. As mentioned above, the method
is inaccurate and the process may cause accumulated
errors without laboratory measurements. Furthermore,
previous prior-free methods
[1−4]
are also impractical for
estimation reflectance using the Procam system since the
adjacent channels of the Procam s ystem are highly cor-
related. These methods are not applicable to high corre-
lation datasets. In this letter, a robust prior-free method
is proposed. We will show the lo cal weighted samples
based on Mahalanobis metric are more appropria te for
estimating spectral reflectance by using the Proca m sys-
tem.
The method is largely inspired by the Procam color-
mixing (PCCM) matrix which was introduced to radio-
metric compensation previously
[8]
. The 3 × 3 matrix is
defined to describe the behavior of pr ojection surface in
the feedback o f a Procam system. Spectral reflectance
is an intrinsic characteristic independent of the spec tral
distribution of illumination and sensitivity of camera sen-
sors, thus we estimate it from the PCCM matr ix. By us-
ing a known spectral reflectance and the cor responding
PCCM matrix o f the training samples, the goal spectral
reflectance can be recovered. To recover the absolute re-
flectance ratio each time, a certain distance between the
projector and the reflection surface is necessary. Obvi-
ously, we can so lve the extrinsic parameters (pose and
position) of a Procam system by using geometric calibra-
tion techniques
[9]
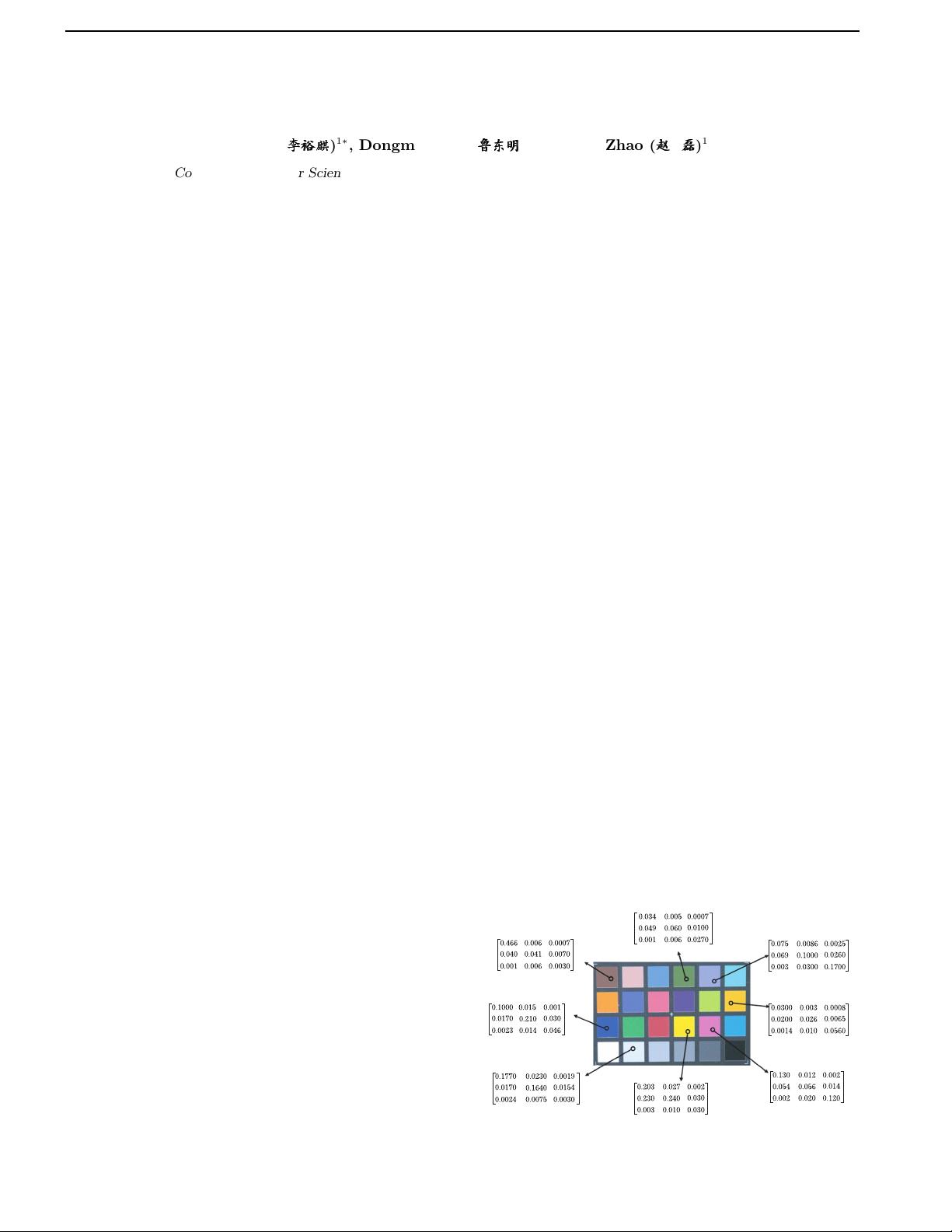
. In practice, we use a 2 4 co lor-Macb eth
chart to create the training set. The PCCM matrix of
several color chips on the Macbeth chart is shown in Fig.
1.
In general, the response model based on the Procam
system can be expressed as
C
mn
=
Z
(P
m
l
m
(λ) + e(λ))s
n
(λ)r(λ)dλ, (1)
where C
mn
is the response of n th channel under the m th
illumination, e(λ) is the spe c tral distribution of noise
light, l
m
(λ) is the spectral distribution of the m th pro-
jector channel, r(λ) is the spectral reflectance of a surface
Fig. 1. PCCM matrix of the color chips on the Macbeth chart.
1671-7694/2013/113301(4) 113301-1
c
2013 Chinese Optics Letters









