Physics Letters B 746 (2015) 347–350
Contents lists available at ScienceDirect
Physics Letters B
www.elsevier.com/locate/physletb
The diamond rule for multi-loop Feynman diagrams
B. Ruijl
a,b,∗
, T. Ueda
a
, J.A.M. Vermaseren
a
a
Nikhef Theory Group, Science Park 105, 1098 XG Amsterdam, The Netherlands
b
Leiden University, Niels Bohrweg 1, 2333 CA Leiden, The Netherlands
a r t i c l e i n f o a b s t r a c t
Article history:
Received
7 May 2015
Accepted
7 May 2015
Available
online 11 May 2015
Editor:
J. Hisano
Keywords:
Feynman
integrals
Integration
by parts identities
An important aspect of improving perturbative predictions in high energy physics is efficiently reducing
dimensionally regularised Feynman integrals through integration by parts (IBP) relations. The well-known
triangle rule has been used to achieve simple reduction schemes. In this work we introduce an extensible,
multi-loop version of the triangle rule, which we refer to as the diamond rule. Such a structure appears
frequently in higher-loop calculations. We derive an explicit solution for the recursion, which prevents
spurious poles in intermediate steps of the computations. Applications for massless propagator type
diagrams at three, four, and five loops are discussed.
© 2015 The Authors. Published by Elsevier B.V. This is an open access article under the CC BY license
(http://creativecommons.org/licenses/by/4.0/). Funded by SCOAP
3
.
1. Introduction
Reducing complexities of Feynman integrals through integration
by parts (IBP) relations [1,2] is an important component of modern
multi-loop calculations. Finding more efficient reduction methods
allows the computation of higher order terms in perturbative ex-
pansions
which in turn aids in providing a better quantitative un-
derstanding
of ongoing experiments. Since the 1980s, the so-called
triangle rule [1,2] has been used for removing a propagator line
from diagrams corresponding to a certain class of integrals. Any
topology that has the following substructure can be simplified us-
ing
the triangle rule:
F (a
1
, a
2
, b, c
1
, c
2
)
=
d
D
k
k
μ
1
...k
μ
N
(
k + p
1
)
2
+ m
2
1
a
1
(
k + p
2
)
2
+ m
2
2
a
2
(k
2
)
b
×
1
(p
2
1
+ m
2
1
)
c
1
(p
2
2
+ m
2
2
)
c
2
(1)
where D is the dimension which is set to 4 − 2 [3,4], and b, c
1
,
c
2
are positive integers. The diagram corresponding to this integral
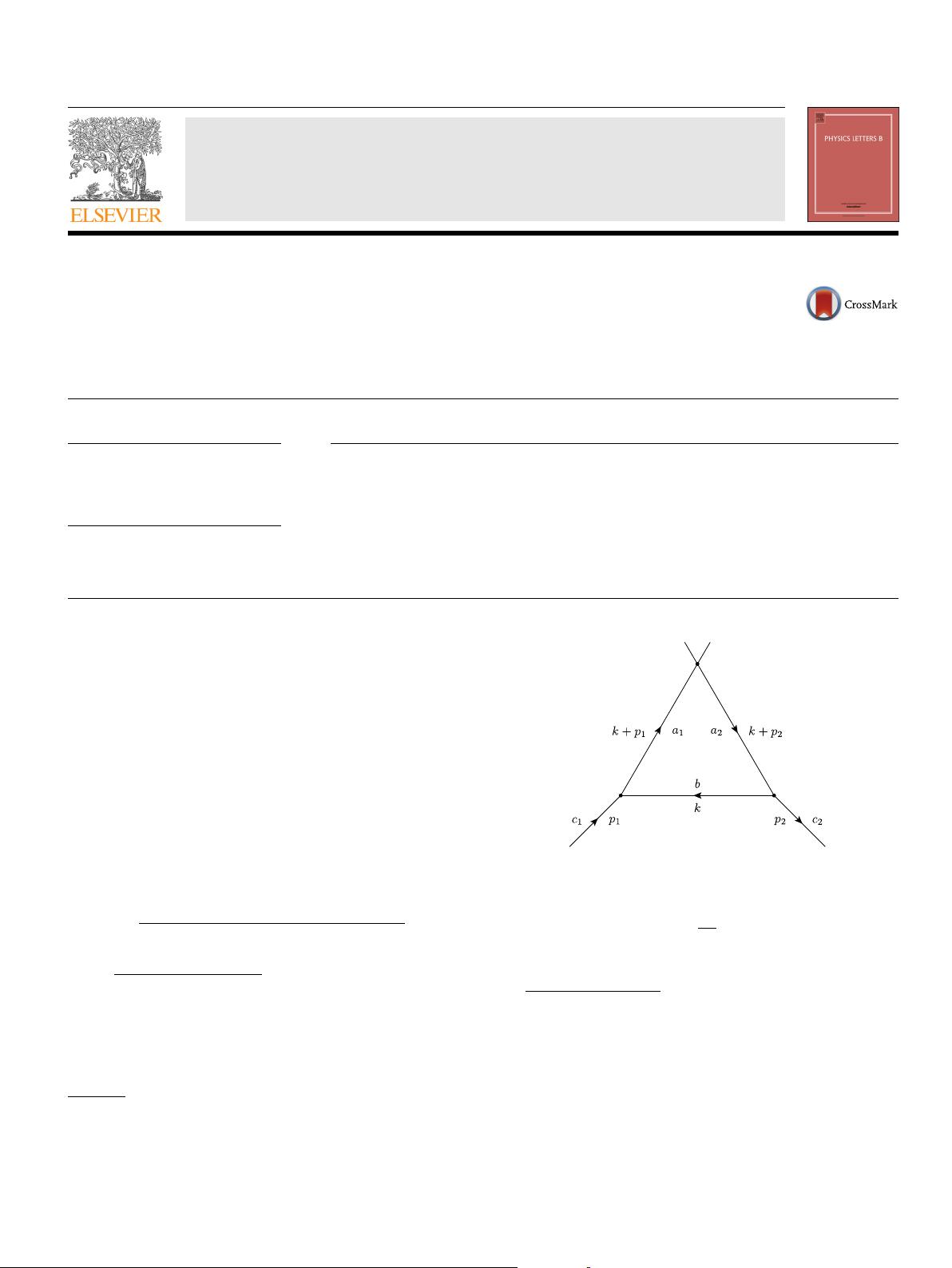
is shown in Fig. 1.
*
Corresponding author.
E-mail
addresses: benrl@nikhef.nl (B. Ruijl), tueda@nikhef.nl (T. Ueda),
t68@nikhef.nl (J.A.M. Vermaseren).
Fig. 1. A triangle subtopology where the loop momentum k is assigned to the central
line. p
1
and p
2
are external momenta. a
1
, a
2
, b, c
1
, and c
2
represent the powers of
their associated propagators.
We write out the IBP relation
∂
∂k
μ
k
μ
F = 0 (where the derivative
must be performed before the integration) to obtain
1 =
1
D + N − a
1
− a
2
− 2b
×
a
1
A
+
1
(B
−
− C
−
1
) + a
2
A
+
2
(B
−
− C
−
2
)
, (2)
where A
+
i
, B
−
, and C
−
i
are operators acting on an integral that
increase the power a
i
by one, decrease the power b by one, and
decrease the power c
i
by one, respectively. Numerators that are
expressed in dot products of k and an external line contribute as a
constant N to the rule. The rule of the triangle can be recursively
http://dx.doi.org/10.1016/j.physletb.2015.05.015
0370-2693/
© 2015 The Authors. Published by Elsevier B.V. This is an open access article under the CC BY license (http://creativecommons.org/licenses/by/4.0/). Funded by
SCOAP
3
.









