LHC下p-Pb碰撞中ϒ(1S)与ϒ(2S)的产率研究与核修正

174 浏览量更新于2024-09-02 收藏 917KB PDF 举报

在大型强子对撞机（LHC）的p-Pb碰撞实验中，研究团队利用ALICE探测器在sNN=5.02 TeV的能量下对clusive（1S）和ϒ（2S）的产生进行了深入分析。这些测量是在两个特定的 rapidity（y）区间内进行的：向下(-4.46 < ycms < -2.96) 和向前(2.03 < ycms < 3.53)，并在零横向动量范围内进行。研究的主要焦点是探讨这些重子onium态在核物质中的行为，即它们的生产率和核修改因子。实验结果显示，ϒ（1S）的产生在正向rapidity区域显示了与pp碰撞相比的抑制现象，这是通过将p-Pb碰撞中的产量与二进制核子-核子碰撞数量的比例进行比较得出的。这可能是由于在高能核-核碰撞中，可能存在核阴影效应，即核子在通过核环境时的部分能量损失导致的ϒ（1S）产生减少。另一方面，在负向rapidity区，这种抑制效应并未观察到，可能暗示着在不同方向上不同的核物理效应。 ϒ（2S）的生产和核修改因子也被测量，并与理论模型进行了对比。这些模型通常考虑了诸如量子色动力学(QCD)中的冷核效应、核密度依赖的修正以及多重散射等因素。通过对实验数据的细致解析，研究人员能够检验这些理论的预测，并可能进一步深化对强相互作用在极端条件下的理解。这项研究不仅提供了关于强子对撞机环境下ϒ介子行为的新数据，也为核物理和QCD在极高能环境下的基本过程提供了宝贵的实验依据。未来的研究可能会继续探索其他更复杂的重子态以及其在核-核碰撞中的行为，以进一步加深我们对强子物理学的理解。

ALICE Collaboration / Physics Letters B 740 (2015) 105–117 107

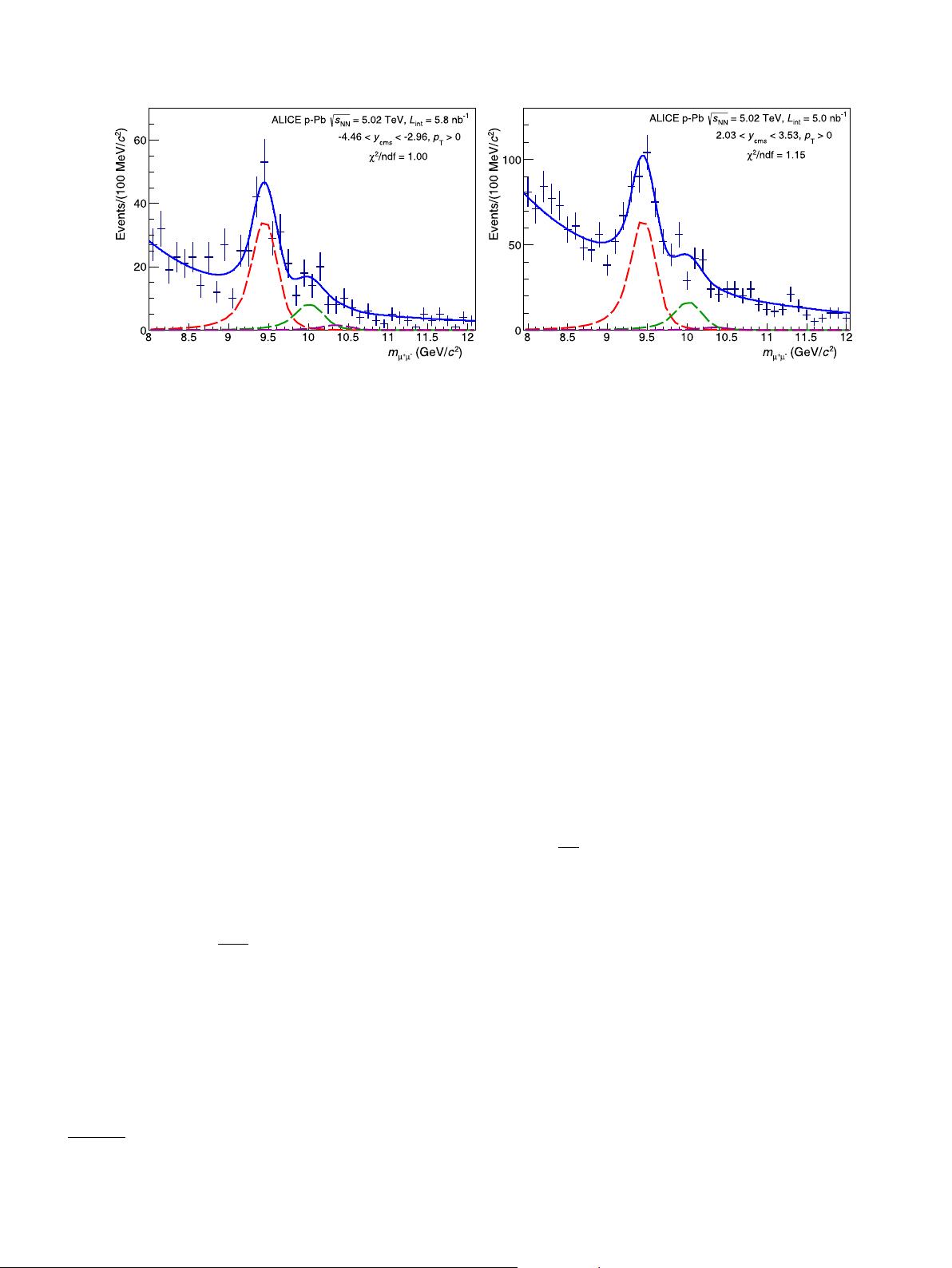
Fig. 1 . Invariant mass distribution of opposite-sign dimuons in the rapidity regions −4.46 < y

cms

< −2.96 (left) and 2.03 < y

cms

< 3.53 (right) in p–Pb collisions. In each

case, the full curve shows the total ﬁt function and the dashed curves the signal component for the three Υ states (see text for details).

3. Data analysis

Muon track candidates are reconstructed in the muon spec-

trometer

using the standard tracking algorithm [44]. The tracks

are required to exit the front absorber at a radial distance from

the beam axis, R

abs

, in the range 17.6 < R

abs

< 89.5cmto reject

tracks crossing the region of the absorber with the highest density

material. In this region, multiple scattering and energy loss effects

are large and can affect the mass resolution. The contribution from

fake and beam-gas interaction induced tracks is reduced by select-

ing

tracks pointing to the interaction vertex. In addition, tracks in

the tracking system are requested to match a track segment in the

trigger system (trigger tracklet).

The Υ signal is obtained from the invariant mass distributions

of opposite-sign dimuons with a laboratory pair-rapidity in the

range 2.5 < |y

lab

| < 4down to zero transverse momentum. The

raw number of Υ is obtained by ﬁtting the invariant mass dis-

tributions.

A sum of two exponential functions is used to param-

eterize

the background continuum, and each Υ resonance shape

is described by an extended Crystal Ball (CB) function [45]. The

CB function is made of a Gaussian core and a power-law tail on

each side and is found to reproduce the shape of the Υ peak

obtained in Monte Carlo (MC) simulations. Since the CB tails are

poorly constrained by the data, they are ﬁxed from the results of

the MC simulations. It is also necessary to ﬁx the mass differ-

ence

between states by using the PDG values [46] and to force

the width of the Υ (2S) and Υ (3S) to scale proportionally with

the Υ (1S) width according to the ratio of the resonance masses.

MC simulations validated these assumptions. The Υ (1S) signal to

background ratio (S/B)

is between 0.8 to 1.8, allowing the posi-

tion

and width of the Υ (1S) peak to be free parameters in the

ﬁt. The signiﬁcance (S/

√

S +B) for Υ (1S) is between 6.3 and 11.6

for

the rapidity bins considered in the analysis. The signiﬁcance

of the Υ (2S) in the rapidity ranges −4.46 < y

cms

< −2.96 and

2.03 < y

cms

< 3.53 is larger than 3, which allows a reliable mea-

surement.

However, due to the limited statistics, the signiﬁcance

of the Υ (3S) state is too low to separate the signal from the un-

derlying

background. Fig. 1 illustrates the ﬁtting method for the

rapidity intervals −4.46 < y

cms

< −2.96 (left panel) and 2.03 <

cms

< 3.53 (right panel). The measured Υ (1S) peak position is in

agreement with the resonance mass value from PDG [46] and the

The signal to background ratio and signiﬁcance numbers are always evaluated

determining the number of signal and background counts in an invariant mass

range centred on the Υ mass and corresponding to ±3times the width of the

peak.

measured width (155 ± 25 MeV/c

in −4.46 < y

cms

< −2.96 and

160 ± 22 MeV/c

in 2.03 < y

cms

< 3.53) agrees with the results

from MC simulations. A similar agreement was observed for all ra-

pidity

bins considered in this Letter.

investigate the systematic uncertainties on the signal extrac-

tion

procedure, different ﬁts were performed parameterizing the

background continuum with the sum of two power-law functions

and using alternative invariant mass ﬁtting ranges. Since some pa-

rameters

are ﬁxed in the ﬁtting procedure, the related systematic

uncertainties were also studied. The CB tail parameters were var-

ied

according to their spread obtained by several ﬁts of the MC

distributions in different mass ranges. The width of the Υ (2S) and

Υ (3S) were varied according to the size of the uncertainties of

the Υ (1S) width obtained from the ﬁt. The latter method was

similarly used to estimate the systematic uncertainty related to

the ﬁxing of the Υ (2S) and Υ (3S) peak position. The raw num-

ber

of Υ (1S) and Υ (2S) in the rapidity range −4.46 < y

cms

−

2.96 are 161 ± 21(stat) ± 9(syst) and 42 ± 14(stat) ± 5(syst),

respectively. In the 2.03 < y

cms

< 3.53 rapidity range, they are

305 ± 34(stat) ± 13(syst) for Υ (1S) and 83 ± 23(stat) ± 10(syst)

for Υ (2S).

The acceptance-times-eﬃciency of the muon spectrometer for

the measurement of Υ , A × ε, is calculated with MC simulations.

The p

and y distributions of the generated Υ (1S) were extrapo-

lated,

with a procedure equivalent to the one adopted for the J/ψ

[47], to

√

= 5.02 TeV from existing pp measurements [48–50].

Nuclear shadowing calculations [51] were used to include the ex-

pected

CNM effects. The systematic uncertainty was estimated by

varying the p

and y input distributions by an amount suﬃ-

ciently

large (based on theoretical estimations) to include the a

priori unknown impact of CNM effects. Since the available data

favour a zero or small polarization of Υ (1S) [52–54], an unpolar-

ized

production was assumed. Particle transport is performed us-

ing

GEANT3 [55] and a realistic detector response is applied to the

simulated hits in order to reproduce the performance of the appa-

ratus

during data taking. The time dependence of the tracking and

trigger eﬃciencies is taken into account by incorporating in the

MC simulations the dead channel maps obtained from the online

detector information and the trigger chamber eﬃciencies obtained

from a real data analysis. In addition, a realistic description of the

residual misalignment of the tracking chambers is included in the

simulations. The tracking eﬃciency is evaluated with data by an-

alyzing the

cluster distribution of the reconstructed tracks in the

detection chambers with the algorithm described in [44]. The same

algorithm can be used to estimate the tracking eﬃciency from MC

data. The systematic uncertainties on this value are obtained by

剩余12页未读，继续阅读

weixin_38651468

粉丝: 5
资源: 896

LHC下p-Pb碰撞中ϒ(1S)与ϒ(2S)的产率研究与核修正

多奇异的重子产生在sNN = 5.02 TeV的p Pb碰撞中

在sNN = 5.02 TeV的p–Pb碰撞中产生Σ（1385）±和Ξ（1530）0

在sNN = 5.02 TeV的p–Pb碰撞中从重味强子衰变产生的介子

在sNN = 5.02TeV的Pb–Pb碰撞中快速测量Z 0玻色子产生

在sNN = 5.02TeV的p–Pb碰撞中测量来自重味强子衰变的电子

在sNN = 5.02TeV时p–Pb碰撞中包容性初级带电粒子产生的横向动量依赖性

Ï•-前向速度的介子生产在sNN = 5.02 TeV处的p–Pb碰撞中和在s = 2.76 TeV处的pp碰撞中

在sNN = 5.02TeV时p–Pb碰撞中J /ψ的产生与带电粒子假快速密度的函数

J / Ïˆ抑制sNN = 5.02 TeV下Pb–Pb碰撞中的前向速度

$$ \ sqrt {s_ {NN}} = 5.02 $$ sNN = 5.02 TeV的p–Pb碰撞的热模型描述

最新资源