ATLAS探测器测量13TeV质子-质子碰撞中H→WW→eνμν的胶子-胶子融合与矢量-玻色子融合希格斯玻色子产生截面
125 浏览量
更新于2024-07-16
收藏 1.8MB PDF 举报
"这篇论文是ATLAS合作组在《Physics Letters B》期刊上发表的研究成果，详细报告了在质子-质子碰撞中通过胶子-胶子融合和矢量-玻色子融合机制产生希格斯玻色子的截面测量情况。实验基于2015年和2016年在大型强子对撞机(Large Hadron Collider, LHC)上以13 TeV质心能量进行的碰撞数据，总集成光度为36.1fb^-1。研究中分析了H→WW^*→eνμν的衰变通道，测量结果显示，该衰变分支的乘积与胶子-胶子融合和矢量-玻色子融合截面之积分别为11.4 pb和0.50 pb，统计和系统误差在内，这些结果与标准模型的预测相符。"
这篇研究的核心知识点包括：
1. **希格斯玻色子**：希格斯玻色子是粒子物理学标准模型中的基本粒子，它负责通过希格斯机制赋予其他基本粒子质量。2012年，LHC的ATLAS和CMS实验宣布发现了希格斯玻色子。
2. **胶子-胶子融合**：这是在高能粒子碰撞中产生希格斯玻色子的主要机制之一。胶子是强相互作用的媒介粒子，存在于质子和中子内部的夸克之间。两个胶子可以融合产生一个希格斯玻色子。
3. **矢量-玻色子融合**：另一种产生希格斯玻色子的方式，涉及两个弱力媒介的矢量玻色子（W或Z玻色子）的融合。这个过程在高能质子-质子碰撞中相对较少见，但仍然是探测希格斯玻色子的重要途径。
4. **H→WW^*→eνμν衰变通道**：这是希格斯玻色子衰变到一对虚拟W玻色子，进而转化为一个电子、一个中微子和一个μ子、另一个中微子的过程。这种衰变模式有助于在实验中识别希格斯玻色子信号。
5. **质子-质子碰撞数据**：研究的数据来源于LHC的质子-质子碰撞，其中质心能量为13 TeV，这是当前最高能量的粒子对撞实验。
6. **集成光度**：36.1fb^-1表示实验收集的数据量，fb^-1是“逆 femtobarn”单位，用于衡量高能物理实验中粒子碰撞的密度。
7. **测量误差**：统计误差和系统误差是实验测量的不确定度来源。在这项研究中，它们分别给出了对结果的上下限。
8. **标准模型预测**：实验结果与标准模型的预测相符合，这验证了标准模型的准确性，同时为可能的新物理现象提供了限制。
9. **《Physics Letters B》**：这是一个著名的物理学期刊，发表高质量的物理研究论文，特别是粒子物理学领域的研究成果。
这项研究为理解希格斯玻色子的性质及其产生机制提供了重要的实验数据，进一步巩固了粒子物理学的标准模型。
512 The ATLAS Collaboration / Physics Letters B 789 (2019) 508–529
the invariant mass of the two leptons, φ
is the azimuthal
angle between the two leptons, and max
m
T
is the larger of
m
i
T
=
2 p
i
T
· E
miss
T
·
1 −cosφ
i
, E
miss
T
, where
i
can be ei-
ther
the leading or the subleading lepton. The “outside lepton veto”
requires the two leptons to reside within the rapidity gap spanned
by the two leading jets, and the “central jet veto” rejects events
with additional jets with p
T
> 20 GeV in the rapidity gap between
the two leading jets. In the N
jet
=1 and N
jet
≥2categories, the
invariant mass of the τ -lepton pair (m
ττ
), calculated using the
collinear approximation [71], is used to veto background from Z →
ττ production. Signal regions (SRs) are defined in each N
jet
cate-
gory
after applying all selection criteria. For both the N
jet
=0 and
N
jet
=1 ggF SRs, eight regions, later used for the fit, are defined by
subdividing in m
at m
< 30 GeV and m
≥ 30 GeV, in p
T
of
the subleading lepton at p
sublead
T
< 20 GeV and p
sublead
T
≥ 20 GeV,
and by the flavour of the subleading lepton. For the categories
with zero jets and with exactly one jet, the discriminating vari-
able
between signal and SM background processes is the dilepton
transverse mass, defined as m
T
=
E
T
+ E
miss
T
2
−
p
T
+ E
miss
T
2
where E
T
=
|p
T
|
2
+m
2
and p
T
is the vector sum of the lep-
ton
transverse momenta. The discriminating variable m
T
is used in
the ggF SRs, with eight bins for the N
jet
=0 and six bins for the
N
jet
=1regions. The bin boundaries are chosen such that approxi-
mately
the same number of signal events is expected in each bin.
The m
T
distributions for the N
jet
=0 and N
jet
=1SRs are shown
in Fig. 3. All figures in this Letter, except Fig. 2, use signal and
background normalisations as fitted by the final statistical analysis
of all signal and control regions, including pulls of statistical and
systematic uncertainty parameters (post-fit). For the N
jet
≥2VBF
selection, a boosted decision tree (BDT) [72]is used to enhance
discrimination power between the VBF signal and backgrounds,
including the ggF process. Kinematic variables of the two lead-
ing
jets ( j) and the two leading leptons () are used as inputs
to the BDT: the invariant masses (m
jj
, m
), the difference be-
tween
the two jet rapidities (y
jj
), and the difference between
the azimuthal angles of the two leptons (φ
). Other variables
used in the BDT training are: m
T
, the lepton η-centrality (
C
,
where C
=|2η
−
η
j
|/η
jj
), which quantifies the positions of
the leptons relative to the leading jets in pseudorapidity [73], the
sum of the invariant masses of all four possible lepton–jet pairs
(
, j
m
j
), and the total transverse momentum (p
tot
T
), which is de-
fined
as the magnitude of the vectorial sum of all selected objects.
The observables providing the best discrimination between signal
and background are m
jj
and y
jj
, and are shown in Fig. 4 after
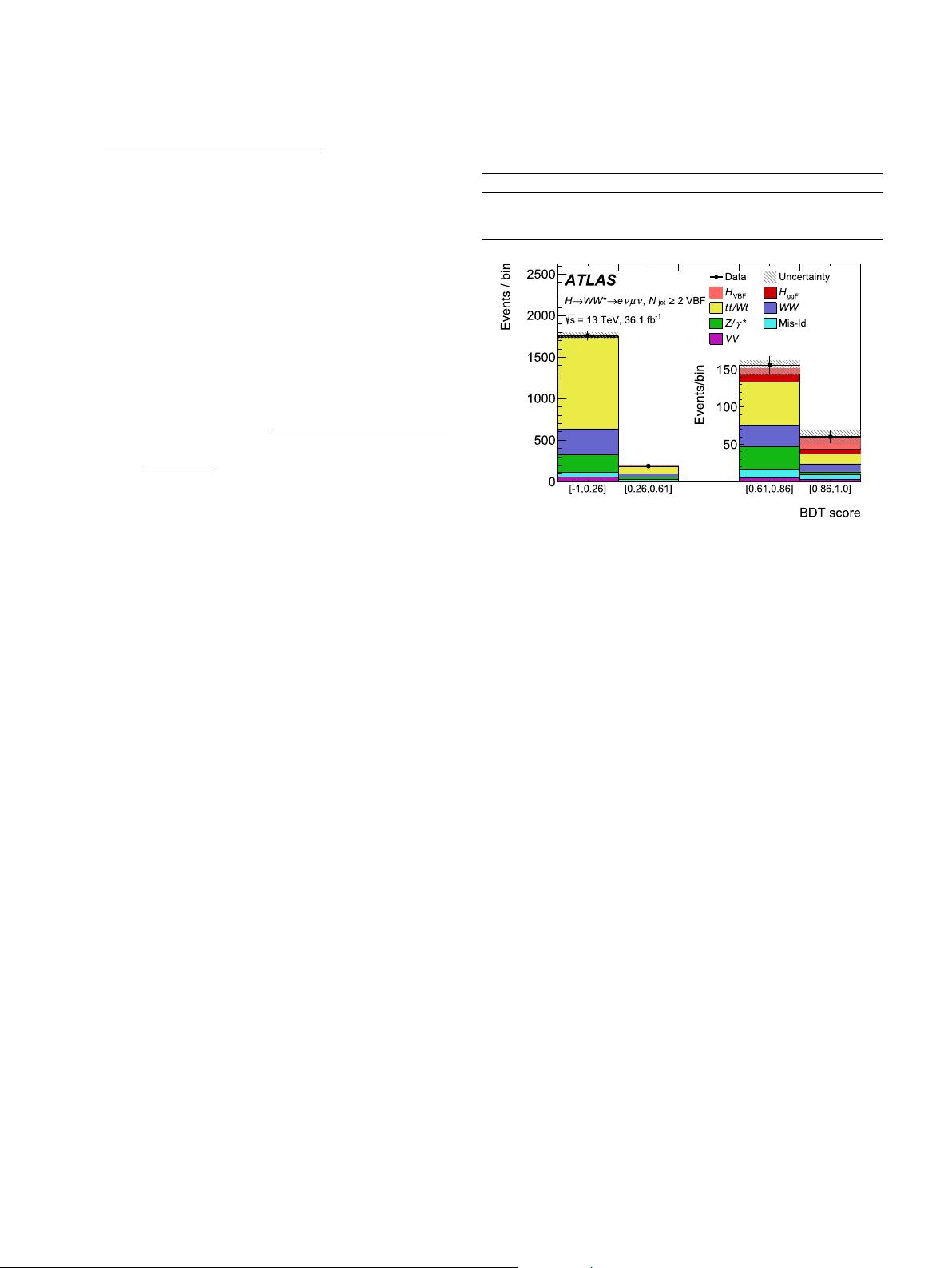
applying all selections. The BDT score reflects the compatibility of
an event with VBF-like kinematics. Signal-like events would tend
to have high BDT score, while background-like events tend to have
low BDT score. The signal purity, therefore, increases at high values
of BDT score. The BDT score is used as the discriminating variable
in the statistical analysis with four bins. The bin boundaries are
chosen to maximise the expected sensitivity for the VBF produc-
tion
mode, resulting in smaller bin widths for larger values of the
BDT score. In the highest-score BDT bin, the expected signal-to-
background
ratio of the VBF signal is approximately 0.6. The BDT
distribution for the VBF-enriched region is presented in Fig. 5.
5. Background estimation
The background contamination in the SRs originates from vari-
ous
processes: non-resonant WW, top-quark pair (t
¯
t) and single-
top-quark
(Wt), diboson (WZ, ZZ, W γ and W γ
∗
) and Drell–Yan
(mainly Z →ττ, hereafter denoted Z /γ
∗
) production. Other back-
Table 4
Post-fit
normalisation factors which scale the corresponding estimated yields in the
signal region; the dash indicates where MC-based normalisation is used. The errors
include the statistical and systematic uncertainties.
Category WW t
¯
t/Wt Z/γ
∗
N
jet,(p
T
>30 GeV)
= 0 ggF 1.06 ± 0.09 0.99 ± 0.17 0.84 ± 0.04
N
jet,(p
T
>30 GeV)
= 1 ggF 0.97 ± 0.17 0.98 ± 0.08 0.90 ± 0.12
N
jet,(p
T
>30 GeV)
≥ 2VBF – 1.01± 0.01 0.93 ± 0.07
Fig. 5. Post-fit BDT score distribution with the signal and the background modelled
contributions
in the VBF signal region. The hatched band shows the total uncer-
tainty
of the signal and background modelled contributions.
ground contributions arise from W +jets and multi-jet production
with misidentified leptons, which are either non-prompt leptons
from decays of heavy-flavour hadrons or jets faking prompt lep-
tons.
Dedicated regions in data, identified hereafter as control re-
gions
(CRs), are used to normalise the predictions of some of the
background processes. CRs are defined for the main background
processes: WW (only for N
jet
≤1 final states), t
¯
t/Wt, and Z /γ
∗
.
Table 3 summarises the event selection for all CRs. For the N
jet
=0
and
N
jet
=1 WW CRs, m
selections orthogonal to those of the
SRs are applied. For the t
¯
t/Wt CRs, the b-veto is replaced with a
b-tag requirement. For the N
jet
=1 and N
jet
≥2VBF Z/γ
∗
CRs, the
m
ττ
selection is inverted, while for the N
jet
=0 Z/γ
∗
CR the φ
selection criterion is inverted. Fig. 6 presents the post-fit m
T
dis-
tributions
in the N
jet
=0 and N
jet
=1CRs.
In
Fig. 7, the post-fit y
jj
distributions in the N
jet
≥2VBF
CRs are shown. Data and simulation are in agreement within un-
certainties
for all the relevant distributions in the different CRs.
The background contributions with misidentified leptons are esti-
mated
using a data-driven technique. A control sample where one
of the two lepton candidates fails to meet the nominal identifica-
tion
and isolation criteria but satisfies looser identification criteria,
referred as an anti-identified lepton, is used. The contribution of
this background in the SRs and CRs is then obtained by scaling
the number of data events, after the subtraction of processes with
two prompt leptons, in the control samples by an extrapolation
factor. The latter is measured in a Z+jets-enriched data sample,
where the Z boson decays to a pair of electrons or muons, and
the misidentified lepton candidate recoils against the Z boson. The
extrapolation factor is defined as the ratio of the numbers of iden-
tified
and anti-identified leptons, and is measured in bins of p
T
and η. Furthermore, a sample composition correction factor is ap-
plied
separately in p
T
< 25 GeV and p
T
> 25 GeV bins, and is
defined in each bin as the ratio of the extrapolation factors mea-
sured
in W +jets and Z +jets MC simulation. The total uncertainty
of the background with misidentified leptons includes uncertain-
ties
due to the difference in sample composition between the
剩余21页未读，继续阅读
点击了解资源详情
点击了解资源详情
点击了解资源详情
2020-03-29 上传
2020-04-16 上传
2020-04-30 上传
2020-04-08 上传
2020-05-01 上传
2020-04-06 上传
weixin_38643401
- 粉丝: 5
- 资源: 953
我的内容管理
展开
最新资源
- 深入浅出：自定义 Grunt 任务的实践指南
- 网络物理突变工具的多点路径规划实现与分析
- multifeed: 实现多作者间的超核心共享与同步技术
- C++商品交易系统实习项目详细要求
- macOS系统Python模块whl包安装教程
- 掌握fullstackJS：构建React框架与快速开发应用
- React-Purify: 实现React组件纯净方法的工具介绍
- deck.js：构建现代HTML演示的JavaScript库
- nunn：现代C++17实现的机器学习库开源项目
- Python安装包 Acquisition-4.12-cp35-cp35m-win_amd64.whl.zip 使用说明
- Amaranthus-tuberculatus基因组分析脚本集
- Ubuntu 12.04下Realtek RTL8821AE驱动的向后移植指南
- 掌握Jest环境下的最新jsdom功能
- CAGI Toolkit：开源Asterisk PBX的AGI应用开发
- MyDropDemo: 体验QGraphicsView的拖放功能
- 远程FPGA平台上的Quartus II17.1 LCD色块闪烁现象解析
