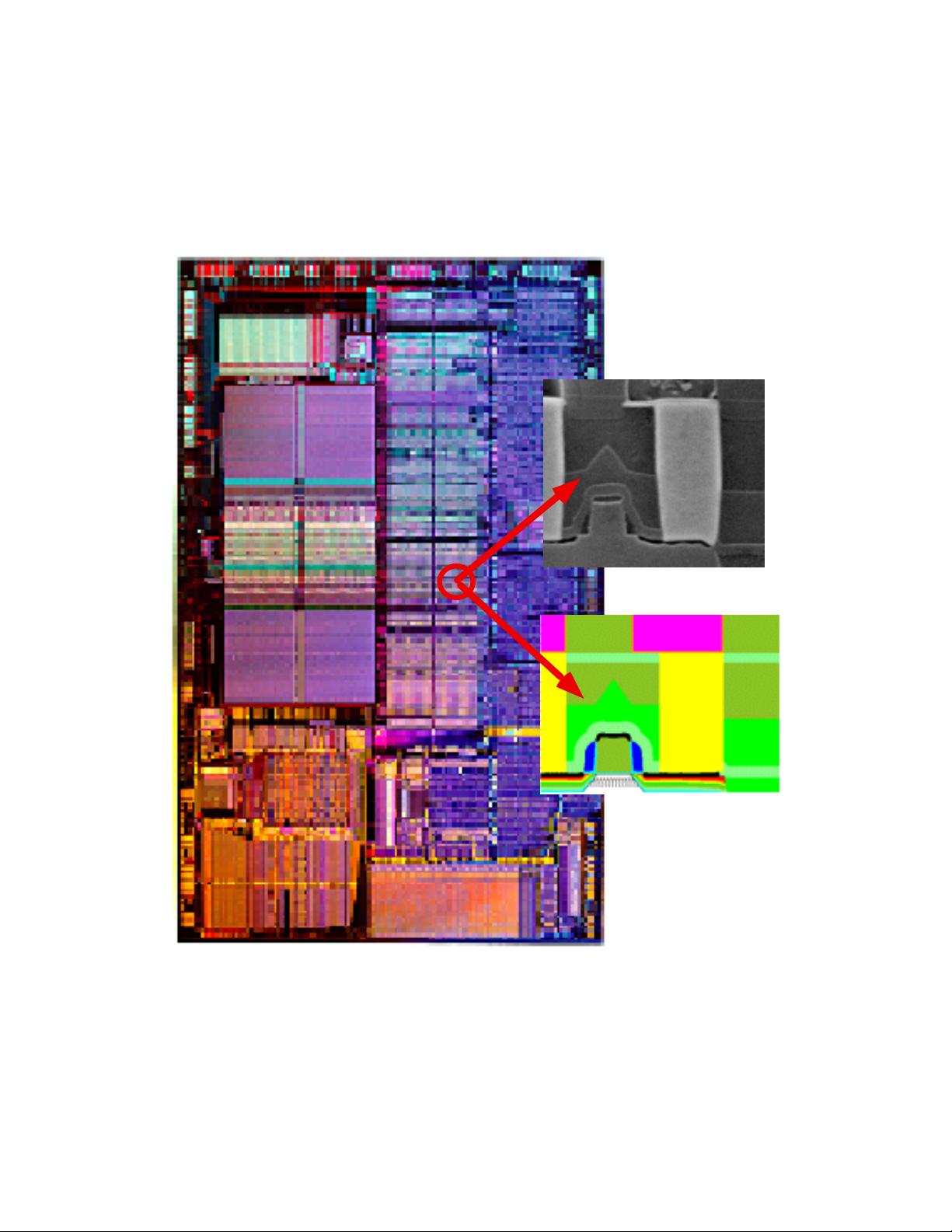
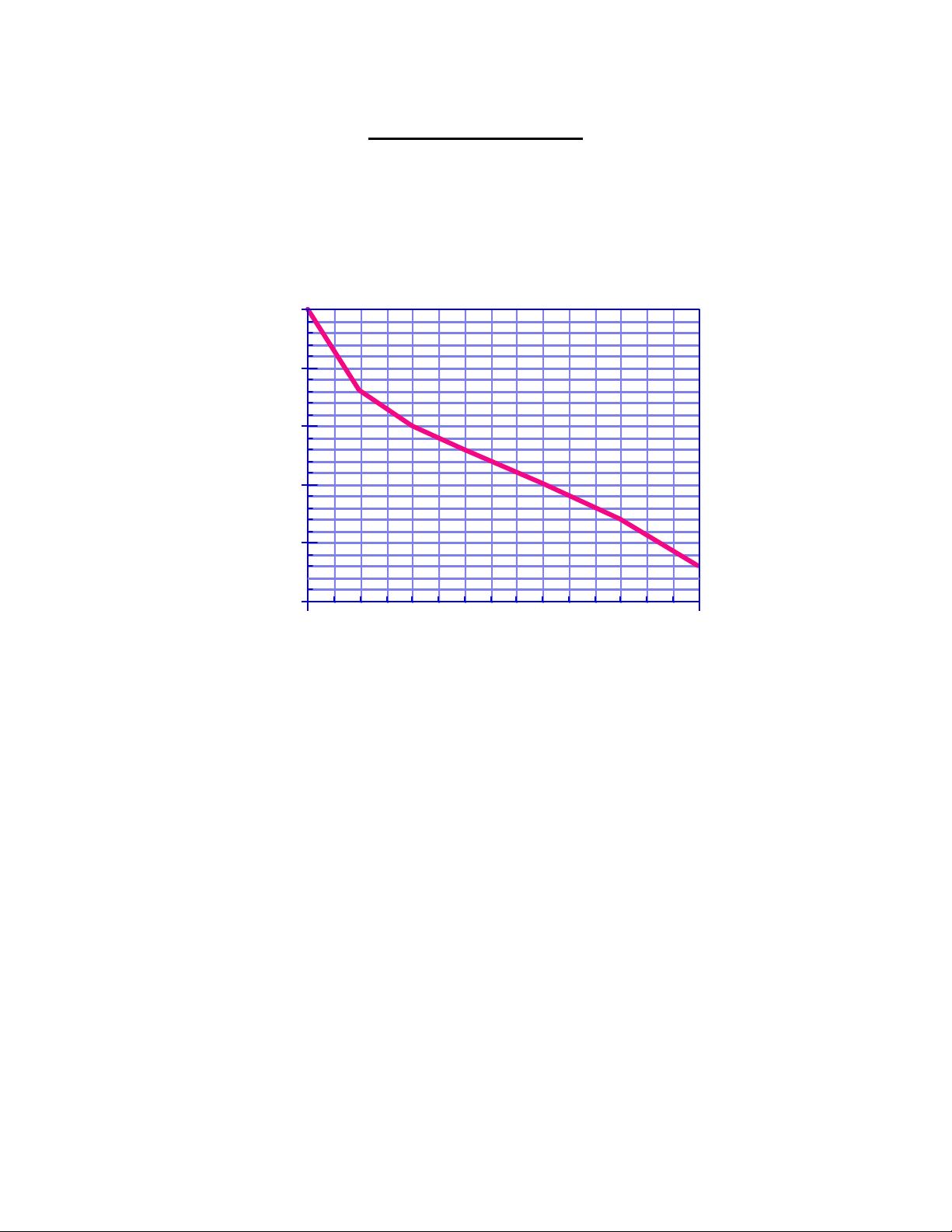
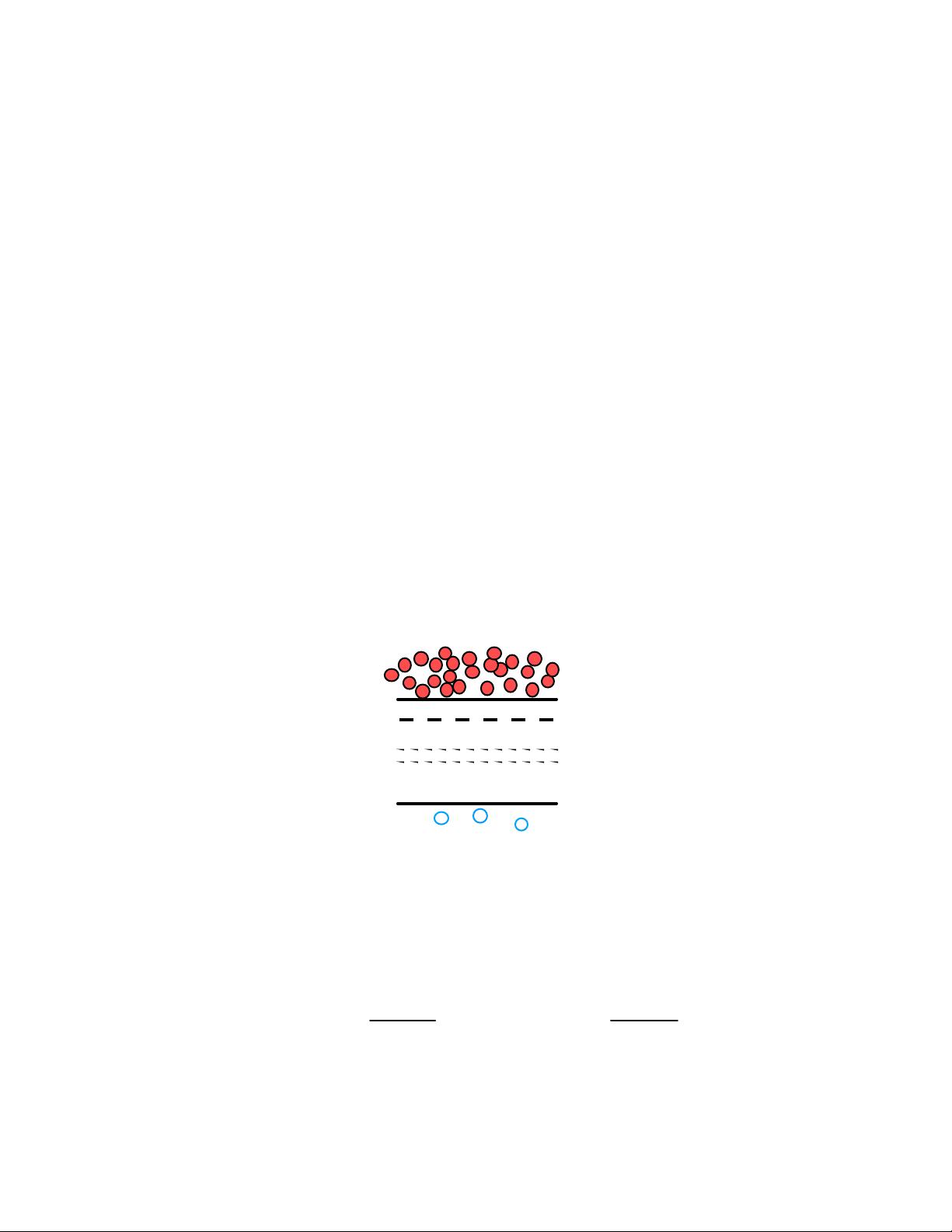
Silicon VLSI Technology_Solutions_Manual_Revised
Berkeley教材Silicon VLSI Technology Fundamemtals, Practice and Modeling的答案,非常全面!
点击了解资源详情
点击了解资源详情
点击了解资源详情
2022-09-22 上传
2019-07-05 上传
2022-09-22 上传
2022-09-21 上传
2022-09-14 上传
2022-09-23 上传
Mith1293073864
- 粉丝: 0
- 资源: 1
最新资源
- windows+2000+双机MSCS安装配置手册
- Java+技能百练.pdf
- jpa 关联映射jpa 关联映射jpa 关联映射
- jdom jdom jdom jdom jdom jdom jdom jdom
- Wrox.Beginning Web Programming with HTML, XHTML, and CSS
- Windows NT 2000 Native API Reference
- Wiley Publishing.Professional Microsoft SQL Server 2008 Administration.2009
- hibernate 用例文档
- Apress.Beginning CSS Web Development From Novice to Professional
- linux0.11内核源代码分析
- Wrox.Beginning Microsoft VisualC# 2008
- Keil与proteus完美结合教程_(PDF格式)
- oracle实验指导书
- Eclipse基本操作手册
- 自动哦不过才怪一分可信的过得答案
- pcb设计规范非常好的资料