Eur. Phys. J. C (2016) 76:424
DOI 10.1140/epjc/s10052-016-4276-5
Regular Article - Theoretical Physics
On factorization of generalized Macdonald polynomials
Ya. Kononov
1,2
, A. Morozov
3,4,5,a
1
Landau Institute for Theoretical Physics, Chernogolovka, Russia
2
Math Department, HSE, Moscow, Russia
3
ITEP, Moscow 117218, Russia
4
Institute for Information Transmission Problems, Moscow 127994, Russia
5
National Research Nuclear University MEPhI, Moscow 115409, Russia
Received: 5 July 2016 / Accepted: 18 July 2016 / Published online: 29 July 2016
© The Author(s) 2016. This article is published with open access at Springerlink.com
Abstract A remarkable feature of Schur functions—the
common eigenfunctions of cut-and-join operators from
W
∞
—is that they factorize at the peculiar two-parametric
topological locus in the space of time variables, which is
known as the hook formula for quantum dimensions of rep-
resentations of U
q
(SL
N
) and which plays a big role in var-
ious applications. This factorization survives at the level of
Macdonald polynomials. We look for its further generaliza-
tion to generalized Macdonald polynomials (GMPs), asso-
ciated in the same way with the toroidal Ding–Iohara–Miki
algebras, which play the central role in modern studies in
Seiberg-Witten–Nekrasov theory. In the simplest case of the
first-coproduct eigenfunctions, where GMP depend on just
two sets of time variables, we discover a weak factorization—
on a one- (rather than four-) parametric slice of the topologi-
cal locus, which is already a very non-trivial property, calling
for proof and better understanding.
Generalized Macdonald polynomials (GMPs) [1–3]play
a constantly increasing role in modern studies of the 6d ver-
sion [4–30] of AGT relations [31–40] and spectral dualities
[41–58]. At the same time they are relatively new special
functions, far from being thoroughly understood and clearly
described. They are deformations of the generalized Jack
polynomials introduced in [59,60]. Even the simplest ques-
tions about them are yet unanswered. In this letter we address
one of them—what happens to the hook formulas for clas-
sical, quantum, and Macdonald dimensions at the level of
GMPs? We find that they survive, but only partly—on a one-
dimensional line in the space of time variables. Lifting to
the entire two-dimensional topological locus remains to be
found.
We begin by recalling that the Schur functions χ
R
{ p},
depend on representation (Young diagram) R and on infinitely
many time variables p
k
(actually, a particular χ
R
depends
a
e-mail: morozov@itep.ru
only on p
k
with k ≤|R|=# boxes in R). They get nicely
factorized on a peculiar two-dimensional topological locus,
p
k
= p
∗
k
≡
1 − A
k
1 − t
k
, (1)
χ
∗
R
(A, t) =
∈R
t
l
()
·
∈R
1 − A · t
a
()−l
()
1 − t
a()+l()+1
. (2)
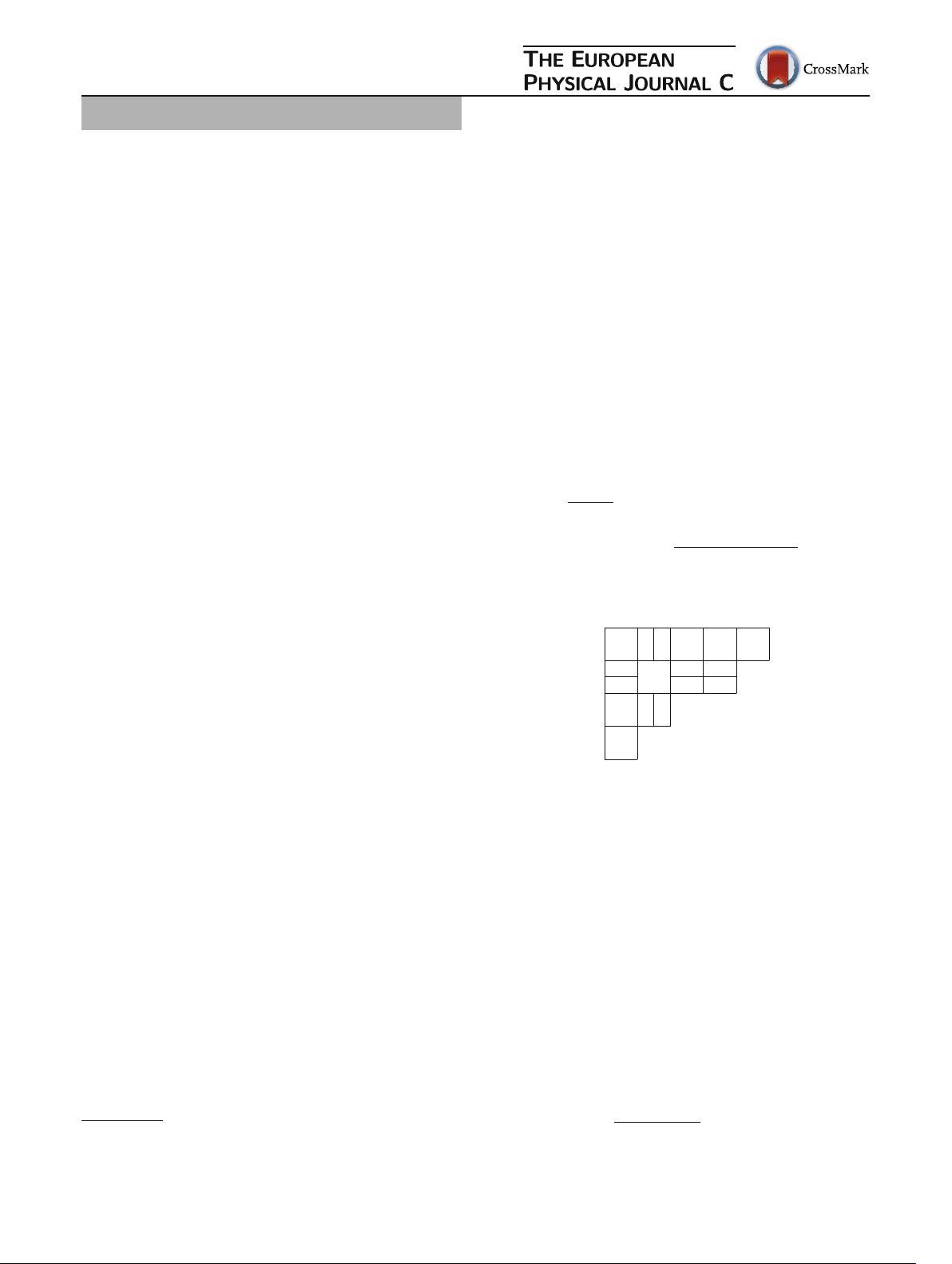
6
-
?
a
l
a
l
Coarm a
and coleg l
are the ordinary coordinates of the
box in the diagram. To keep the notation consistent through-
out the text, in (1) we call the relevant parameter t, not q,
from the very beginning.
It is often convenient to ignore the simple overall coeffi-
cient and substitute the product formulas like (2) by a poly-
nomial expression for a plethystic logarithm
⎛
⎝
∈R
t
l
()
⎞
⎠
−1
· χ
∗
R
= S
•
⎛
⎝
∈R
t
a()+l()+1
− A · t
a
()−l
()
⎞
⎠
(3)
where we use the definition
S
•
( f ) = exp
k
f (t
k
0
,...,t
k
n
)
k
(4)
123









