数字信号处理第四版课后习题2-7章英文答案解析

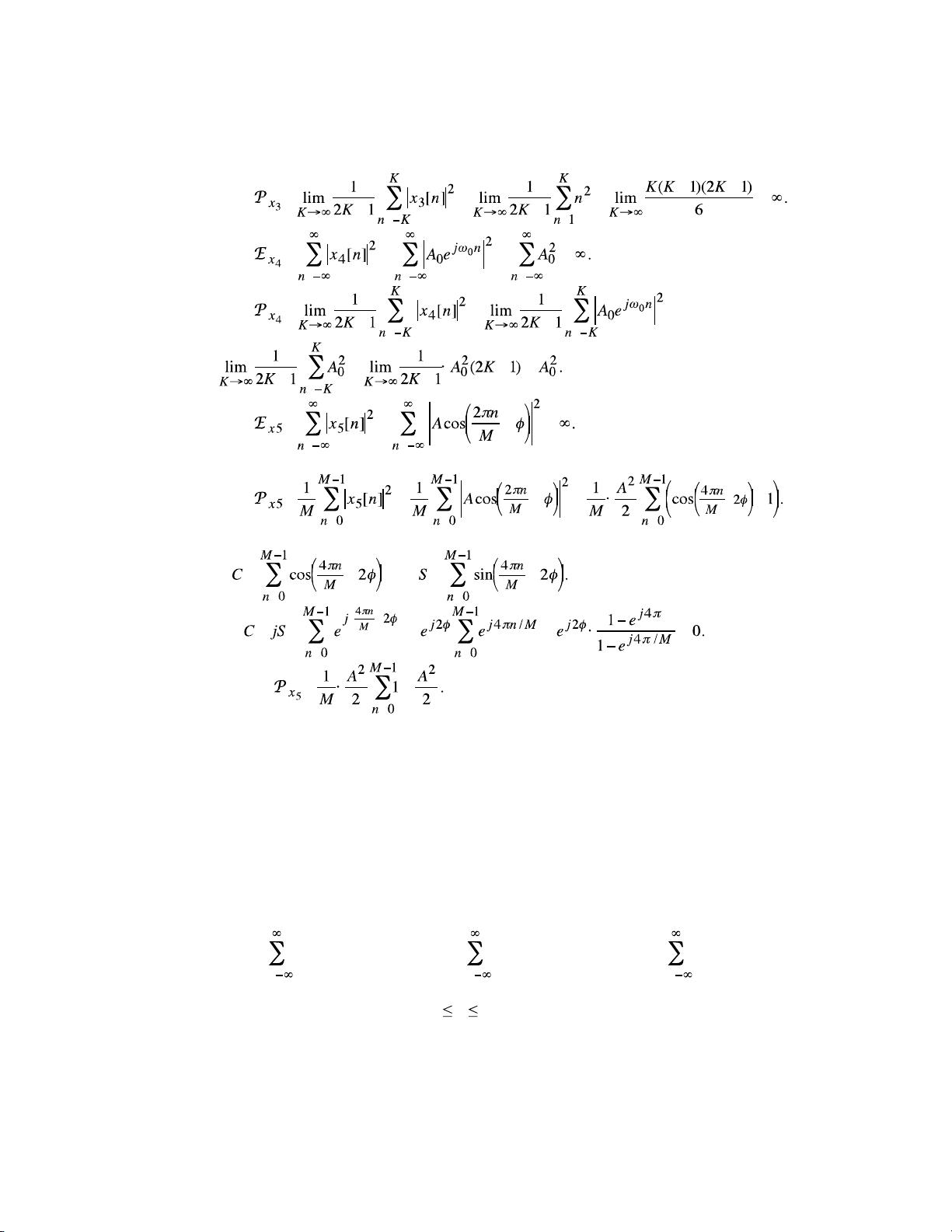
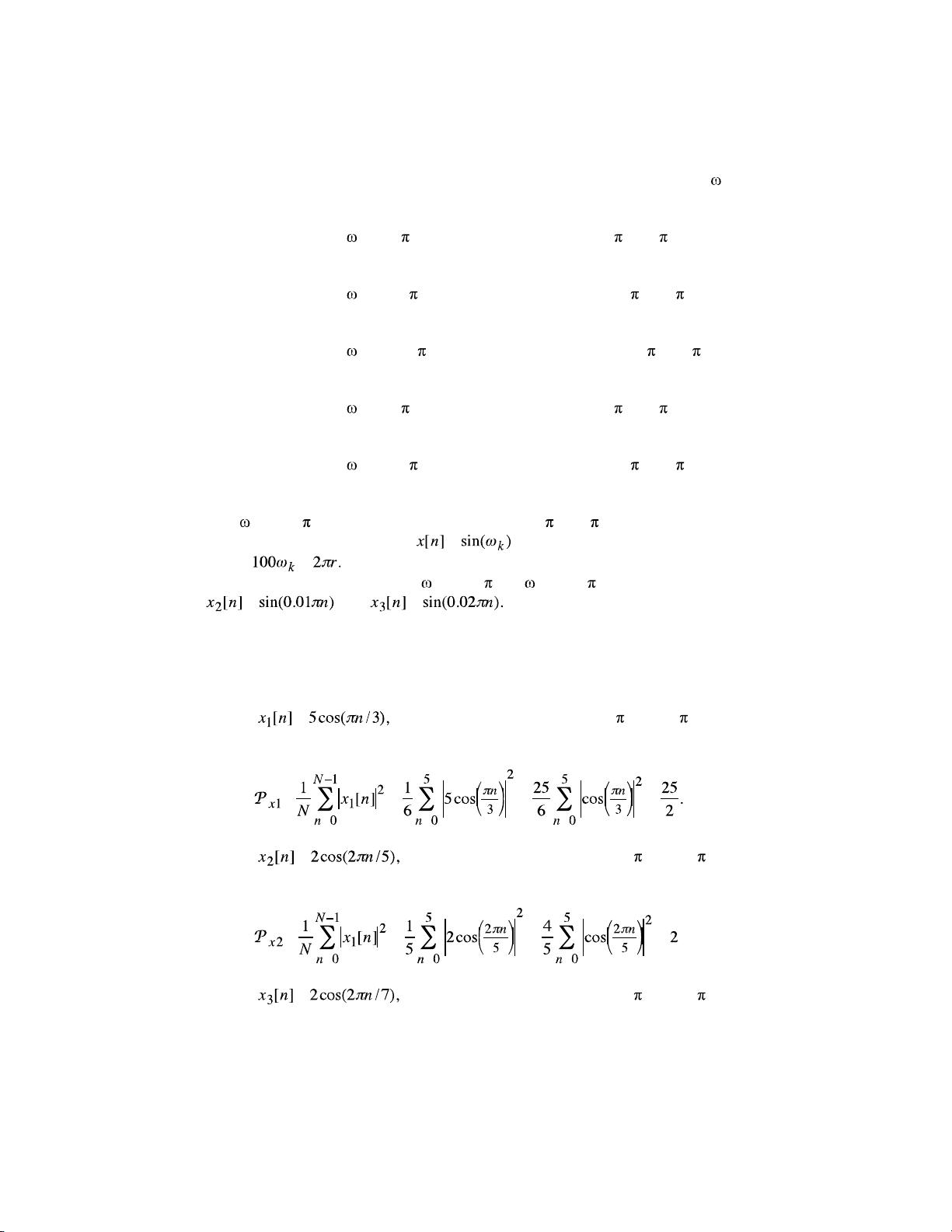
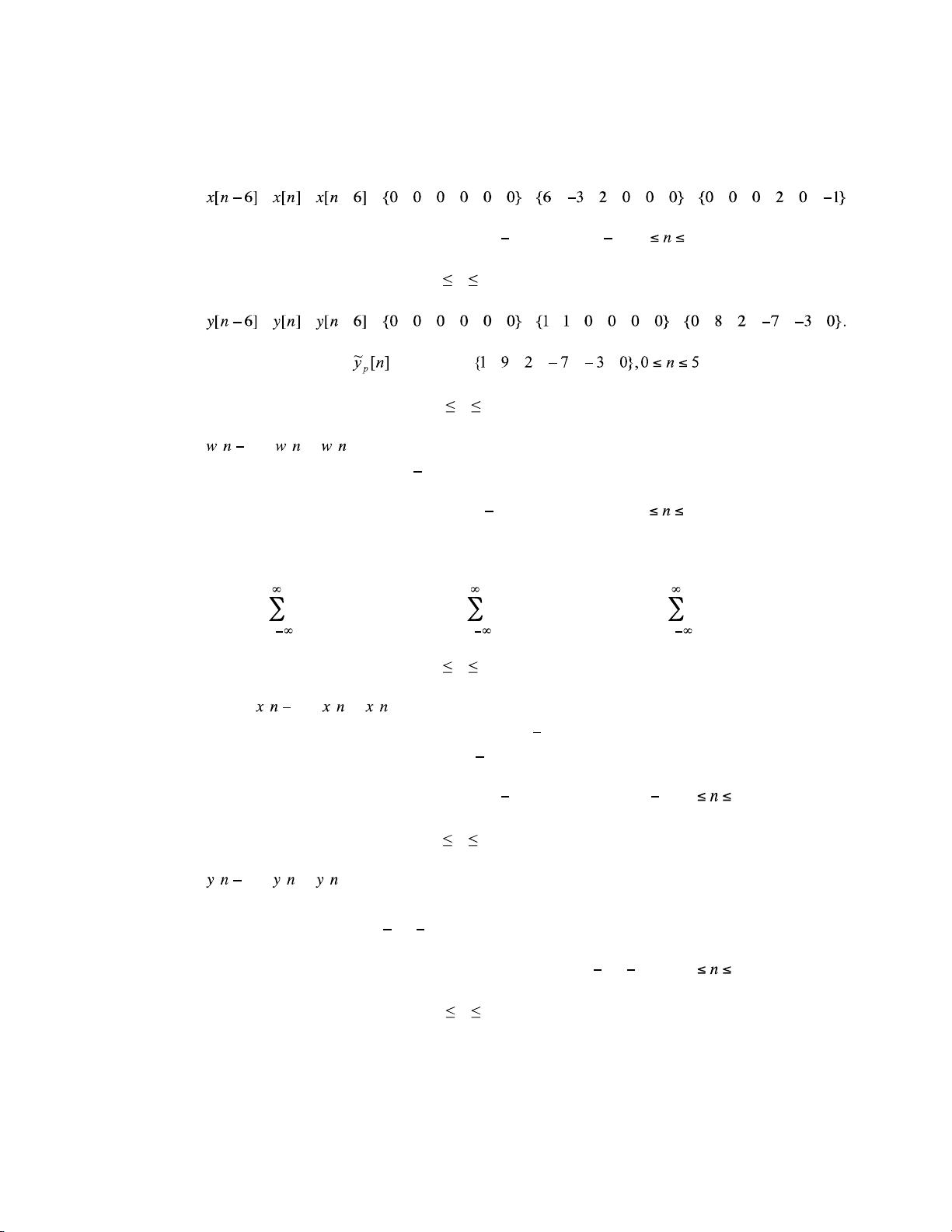
《数字信号处理（第四版）》是一本经典的教材，本资料包含了该书第2-7章的课后习题答案，全英文版。这些章节涵盖了广泛的数字信号处理理论和实践应用。以下是部分内容解析： 2.2节：线性运算与能量计算 利用公式(2.9)，习题要求计算不同信号的线性运算结果。例如，练习涉及求解加权和、平方和以及特定信号的平方根。通过这个部分，学生可以学习如何用数学工具表示和处理数字信号的线性关系，如能量和功率的计算。 2.3节：信号分析和量化 这部分包括对信号的分解和量化操作，如信号的模长、绝对值和幅度计算。例如，习题(a)至(f)展示了对不同信号的这些操作，有助于理解信号在频域和时域中的特性，并熟悉基本的信号分析技巧。 2.7节：滤波器设计与结构分析 在这一节，学生需要处理滤波器的设计问题，如利用给定的信号结构定义滤波器函数。例如，(a)小节中讨论了如何根据中间信号确定滤波器系数，以及滤波器的线性组合形式。通过解决这些问题，学生将学习到滤波器设计的基本原理和结构分析方法。 这些答案不仅提供了具体问题的解答，还揭示了数字信号处理的核心概念，如线性系统理论、信号分析、滤波器设计等。学习者可以通过完成这些习题，加深对数字信号处理算法的理解，提升实际操作能力。值得注意的是，这些内容适合正在学习第四版教材的学生或专业人士查阅和验证他们的理解，不适用于商业销售。