MATLAB线性方程组求解的MATLAB代码示例:深入理解求解过程
发布时间: 2024-06-09 05:45:31 阅读量: 108 订阅数: 43 


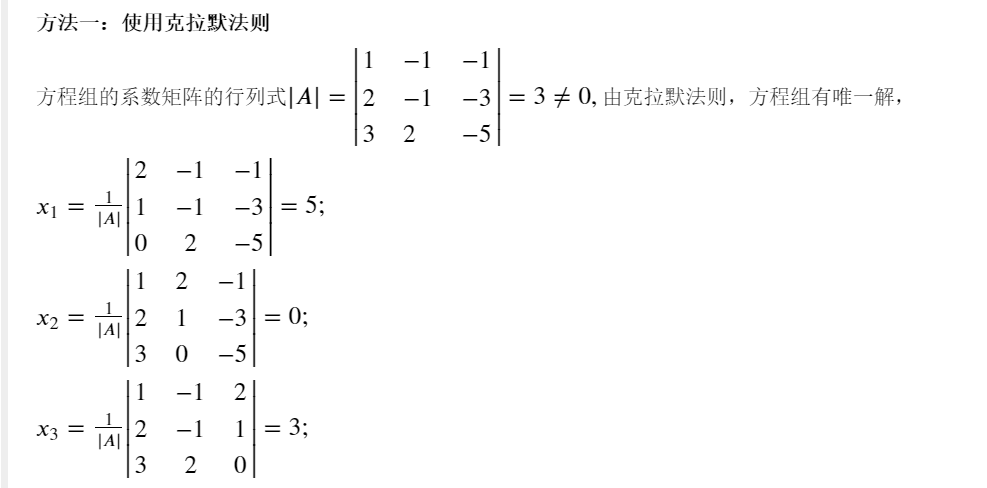
线性方程组求解 matlab代码
# 1. MATLAB线性方程组求解基础
线性方程组求解在科学计算和工程应用中至关重要。MATLAB作为一种强大的数值计算工具,提供了丰富的函数和算法来高效求解线性方程组。本章将介绍MATLAB线性方程组求解的基础知识,包括线性方程组的概念、求解方法以及MATLAB中常用的求解函数。
**1.1 线性方程组的概念**
线性方程组是一组线性方程的集合,每个方程表示为:
```
a_11x_1 + a_12x_2 + ... + a_1nx_n = b_1
a_21x_1 + a_22x_2 + ... + a_2nx_n = b_2
a_mx_1 + a_m2x_2 + ... + a_mnx_n = b_m
```
其中,`a_ij`是系数矩阵中的元素,`x_j`是未知数,`b_i`是常数项。线性方程组的求解目标是找到未知数`x_j`的值,使其满足所有方程。
# 2. MATLAB线性方程组求解理论
### 2.1 线性方程组的数学原理
#### 2.1.1 线性方程组的概念和表示
**线性方程组**是由一组线性方程构成的集合,形式如下:
```
a_11x_1 + a_12x_2 + ... + a_1nx_n = b_1
a_21x_1 + a_22x_2 + ... + a_2nx_n = b_2
a_mx_1 + a_m2x_2 + ... + a_mnx_n = b_m
```
其中:
* `a_ij` 是系数矩阵 `A` 的元素
* `x_j` 是未知数向量 `x` 的元素
* `b_i` 是常数向量 `b` 的元素
* `m` 是方程组的行数
* `n` 是方程组的列数
**矩阵形式**:
线性方程组也可以用矩阵形式表示为:
```
Ax = b
```
其中:
* `A` 是系数矩阵,大小为 `m x n`
* `x` 是未知数向量,大小为 `n x 1`
* `b` 是常数向量,大小为 `m x 1`
#### 2.1.2 线性方程组的求解方法
求解线性方程组的方法有很多,主要分为两类:
* **直接方法**:通过一系列的运算,直接得到未知数的值。
* **迭代方法**:通过不断迭代,逐步逼近未知数的值。
### 2.2 MATLAB线性方程组求解算法
MATLAB 提供了多种求解线性方程组的算法,包括:
#### 2.2.1 Gauss消去法
**Gauss消去法**是一种直接方法,通过一系列行变换将系数矩阵化为上三角矩阵,然后通过回代求出未知数。
**代码块:**
```
% 系数矩阵 A 和常数向量 b
A = [2 1 1; 4 3 2; 8 7 4];
b = [1; 2; 3];
% 使用 Gauss 消去法求解
x = A \ b;
% 输出结果
disp('Gauss 消去法求解结果:');
disp(x);
```
**逻辑分析:**
* `A \ b` 使用 Gauss 消去法求解线性方程组 `Ax = b`。
* `disp(x)` 输出求解结果。
#### 2.2.2 LU分解法
**LU分解法**是一种直接方法,将系数矩阵分解为下三角矩阵 `L` 和上三角矩阵 `U`,然后通过正向和反向替换求出未知数。
**代码块:**
```
% 系数矩阵 A 和常数向量 b
A = [2 1 1; 4 3 2; 8 7 4];
b = [1; 2; 3];
% 使用 LU 分解法求解
[L, U] = lu(A);
y = L \ b;
x = U \ y;
% 输出结果
disp('LU 分解法求解结果:');
disp(x);
```
**逻辑分析:**
* `lu(A)` 将系数矩阵 `A` 分解为 `L` 和 `U`。
* `L \ b` 通过正向替换求解 `Ly = b`。
* `U \ y` 通过反向替换求解 `Ux = y`。
* `disp(x)` 输出求解结果。
#### 2.2.3 QR分解法
**QR分解法**是一种直接方法,将系数矩阵分解为正交矩阵 `Q` 和上三角矩阵 `R`,然后通过求解 `Rx = Q^T b` 得到未知数
0
0





