MIMO-SVD系统在 Ricean 褪色信道中的性能分析：考虑信道估计误差与反馈延迟
173 浏览量
更新于2024-08-27
收藏 703KB PDF 举报
本文主要探讨了多输入多输出（Multiple-Input Multiple-Output, MIMO）系统在瑞利衰落信道中，考虑到信道估计误差和反馈延迟的情况下，分析了比特错误率（Bit Error Rate, BER）和 outage probability 的性能。作者利用了复杂非中心 Wishart 矩阵的无序和有序特征值分布，对基于奇异值分解（Singular Value Decomposition, SVD）的 MIMO 系统进行了深入研究。
SVD 是一种常用的信号处理技术，尤其在无线通信领域中，它能够有效地降低多径效应带来的干扰，提升系统的容量和可靠性。然而，在实际环境中，信道估计并不完美，存在误差，这会影响信号的传输质量。此外，由于无线通信的实时性要求，反馈延迟也是一个不可忽视的因素，它可能导致数据包丢失或接收端解调的不准确。
在瑞利衰落信道中，信号强度会受到路径损耗和多径效应的影响，形成随机变化的幅度。论文通过理论推导，获得了信道估计误差和反馈延迟条件下，系统性能的精确平均表达式，这对于系统设计者来说，可以作为评估系统稳定性和鲁棒性的关键指标。
对于不同类型的调制格式（如QPSK、16QAM、64QAM等），以及任意数量的发射和接收天线配置，这些闭合形式的BER和SINR（Signal-to-Interference-and-Noise-Ratio）近似公式提供了广泛的应用范围。在低至中等的信号到干扰加噪声比（SINR）情况下，这些结果尤为重要，因为它们揭示了系统性能随SINR变化的趋势，有助于优化系统参数以达到最佳性能。
该研究为理解并优化在瑞利衰落环境下具有信道估计误差和反馈延迟的 MIMO-SVD 系统提供了有价值的理论依据，对于无线通信网络的设计、优化和故障分析具有重要意义。实践中，可以通过这些理论结果指导如何选择适当的信道估计方法、设置合理的反馈周期，以及调整系统参数来最小化性能损失，从而实现更高效的通信服务。
AU et al.: ANALYTICAL PERFORMANCE OF MIMO-SVD SYSTEMS IN RICEAN FADING CHANNELS 1317
Letting σ
2
s
|ρ(d)|
2
K+1
σ
2
e
+
1
|ρ(d)|
2
− 1
, t he post-processing
SINR for a particular realization is given by
SINR
i
=
P
i
μ
2
(l
i
)
σ
2
s
m
q=1,q=i
P
q
μ
2
(l
q
)+σ
2
w
(λ
i
+ σ
2
s
).(11)
With equal power allocation, namely P
i
= P
T
/m for all i,
such a realization is simplified as
SINR
i
=
SINR
i
˜
λ
i
(12)
where
˜
λ
i
λ
i
+ σ
2
s
and
SINR
i
=
σ
2
s
m
q=1,q=i
μ
2
(l
q
)
μ
2
(l
i
)
+
mσ
2
w
P
T
μ
2
(l
i
)
−1
. (13)
A. Statistical Characterization of the Post-Processing SINR
To analyze the BER and outage probability performance
of each eigen-subchannel, we require the PDF of SINR
i
for all i, or equivalently, the marginal PDF of a (translated)
ordered eigenvalue
˜
λ
i
of a complex noncentral Wishart ma-
trix. Although there are many results available for complex
central Wishart matrices, there are very few results related
to the noncentral counterpart. Recently, [11] derived new
exact closed-form expressions for marginal CDFs and new
asymptotic first-order expansions for marginal PDFs
1
of the
ordered eigenvalues. In particular, given the first-order ex-
pansion f or the marginal PDF of λ
i
[11, eq. (27)], namely
f
λ
i
(λ
i
), the marginal PDF of
˜
λ
i
can be derived by f(
˜
λ
i
)=
f
λ
i
(
˜
λ
i
− σ
2
s
)
dλ
i
/d
˜
λ
i
. Hence, we generalize their results to
our problem as follows.
Lemma 1: Let ϕ
1
,...,ϕ
r
o
be the non-zero eigenvalues of
K/(K +1)H
u,t
H
H
u,t
with rank r
0
≤ m, and let λ
i
be the
i-th largest eigenvalue of
ˆ
H
t
ˆ
H
H
t
. Then the marginal density
function of
˜
λ
i
= λ
i
+ σ
2
s
is given by
f(
˜
λ
i
)=a
i
˜
λ
i
− σ
2
s
d
i
. (14)
Here, d
i
=(m −i + 1)(n −i +1)−1, and a
i
is given in [11].
In addition to the performance of individual eigen-
subchannels, the average system performance can be derived
by using statistical unordered eigenvalue distributions [7], [8].
In contrast to the first-order expansions for marginal PDFs
of ordered eigenvalues, exact closed-form expressions for
marginal PDFs of unordered eigenvalues are derived in [12].
Thus, given the exact expression for the marginal PDF of
λ
a
[12, Lemma 2], namely, f
λ
a
(λ
a
), the marginal PDF of
˜
λ
a
can be derived by f(
˜
λ
a
)=f
λ
a
(
˜
λ
a
− σ
2
s
)
dλ
a
/d
˜
λ
a
.
Lemma 2: Let φ
m−r
u
+1
,...,φ
m
be the non-zero eigen-
values of KH
u,t
H
H
u,t
with rank r
u
≤ m, and let
λ
a
be an arbitrary (unordered) non-zero eigenvalue of
ˆ
H
t
ˆ
H
H
t
, with a being randomly chosen from {1,...,m}.
Then the marginal PDF of
˜
λ
a
= λ
a
+ σ
2
s
is given
by (15) where κ = e
−
m
l=m−r
u
+1
φ
l
m(τ!)
m−1
m−r
u
l=1
(l −
1)!
m
l=m−r
u
+1
φ
m−r
u
l
m−r
u
+1≤k<l≤m
(φ
l
− φ
k
)
−1
, τ =
1
It is worth noticing that exact marginal PDFs can be trivially obtained
from the exact marginal CDFs by differentiation [9]. However, the resulting
expressions, which are in terms of Nuttall Q-functions [13], are very cum-
bersome to work with.
n − m,
˜
D
i,j
is the (i, j)-th cofactor of an m × m matrix
˜
A
shown in (16) with [s]
t
=(s + t − 1)!/(s − 1)! being the
Pochhammer symbol and
p
F
q
(s
1
,...,s
p
; t
1
,...,t
q
; x) being
the generalized hypergeometric function.
III. B
IT ERROR RAT E ANA LYS I S
For an M-ary constellation, the conditional BER of the i-
th eigen-subchannel is given in the following generic form.
P
E
i; μ(l
i
)
= E
˜
λ
i
C
1
(M)
v
2
v=v
1
c
2
c=c
1
C
2
(v, c, M )
Q
C
3
(c, M)
SINR
i
˜
λ
i
(17)
where v
1
, v
2
, c
1
, c
2
, C
1
(M), C
2
(v, c, M ), C
3
(c, M) are
constellation-dependent parameters and Q(·) is the Gaussian
Q-function. The main advantage of using (17) is its ease
of application to various kinds of modulations such as M-
ary quadrature amplitude modulation (MQAM) and
√
M-ary
pulse amplitude modulation (PAM) [14]. However, for M -
ary phase shift keying (MPSK), where M>4, the exact
expression in (17) reduces to a tight closed-form approxima-
tion [15]
2
. Given (14), we now analyze the BER performance
of an individual eigen-subchannel as follows
3
.
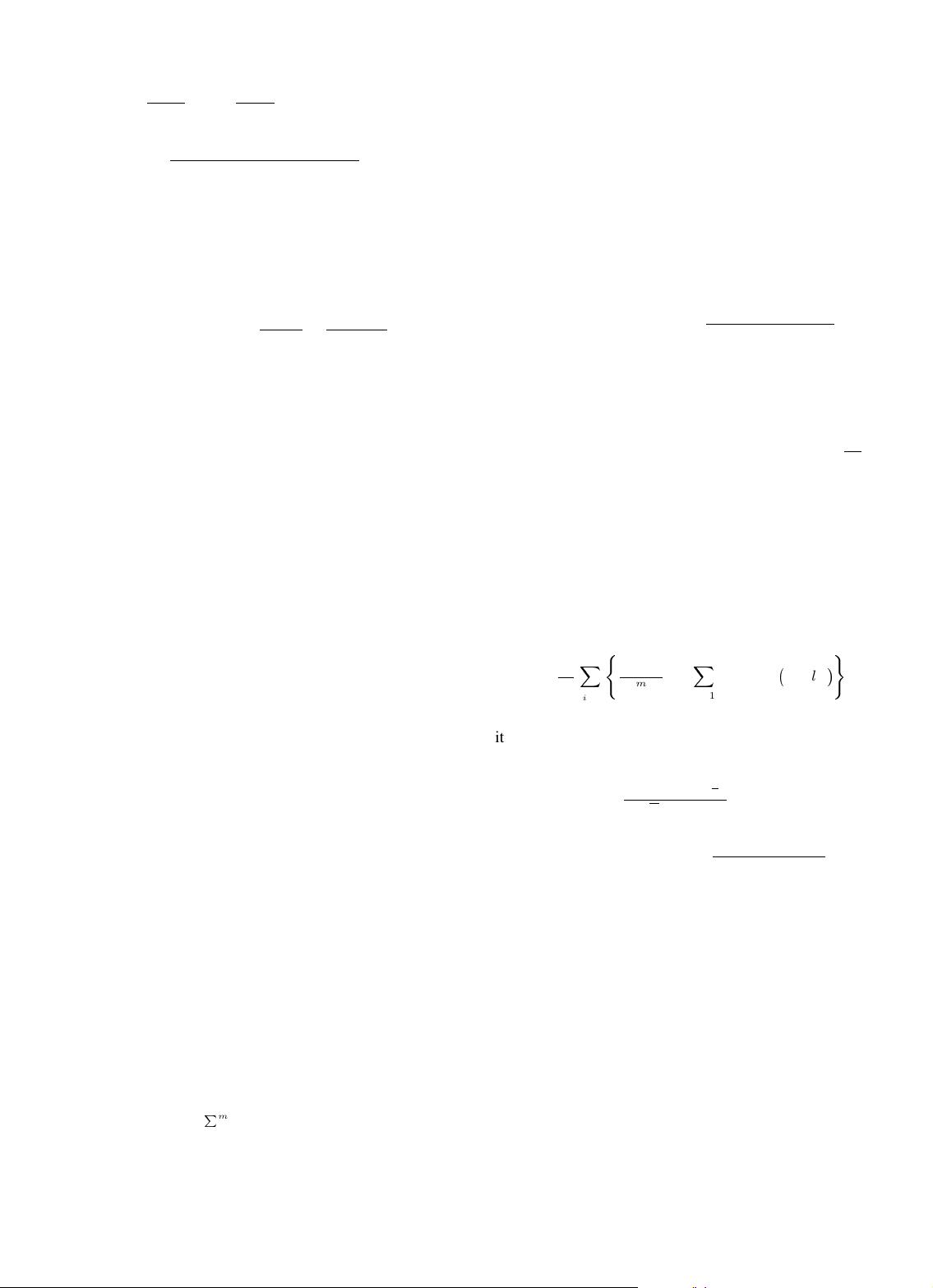
Theorem 1: The BER of the i-th largest eigen-subchannel
of MIMO-SVD systems in Ricean fading, with channel es-
timation error and feedback delay, is given by the following
closed-form high-SINR approximation
P
(∞)
E
(i) ≈
1
M
M
l
i
=1
1
M
m−1
M
l
q
=1,
q=1,...,m,q=i
P
(∞)
E
i; μ(l
i
)
(18)
with P
(∞)
E
i; μ(l
i
)
being the high-SINR conditional BER
approximation
P
(∞)
E
i; μ(l
i
)
≈
a
i
2
d
i
Γ(d
i
+
3
2
)
√
π(d
i
+1)
C
1
(M)
v
2
v=v
1
c
2
c=c
1
C
2
(v, c, M )
C
3
(c, M)
SINR
i
K +1
−d
i
−1
(19)
where Γ(z)=
∞
0
x
z−1
e
−x
dx is the Gamma function [16].
Proof: Given the first-order expansion for the marginal PDF
of an ordered eigenvalue in Lemma 1, the high-SINR con-
ditional BER approximation (19) is obtained by using [20,
Proposition 1] along with [11, Section I V.A] and Appendix III.
Then, (18) can be obtained by un-conditioning (19) over the
amplitudes of constellation symbols of all m − 1 cochannel
interferences followed by averaging over μ(l
i
) of all M
constellation symbols of the i-th eigen-subchannel.
In addition to the individual eigen-subchannels, it is also
important to analyze the average system performance so as
to gain a more thorough insight. It has been shown in [17]
that a significant performance improvement can be obtained
2
For M =2and 4, i.e., BPSK and QPSK, exact expressions can be
obtained by considering them as binary PAM and 4QAM, respectively.
3
It is noteworthy that unequal power allocation can be straight-forwardly
employed in Theorem 1. However, in order to have a fair comparison with the
average BER derived by using marginal unordered eigenvalue distributions in
Theorem 2, we restrict the analysis to systems with uniform power allocation.
剩余10页未读，继续阅读
2021-01-20 上传
2015-06-23 上传
2009-03-24 上传
2023-04-12 上传
2023-06-13 上传
2023-05-13 上传
2023-04-01 上传
2023-05-28 上传
2023-04-25 上传
weixin_38631042
- 粉丝: 4
- 资源: 926
我的内容管理
展开
最新资源
- 汇编语言 实验一 广东工业大学
- PID matlab
- jsp在线考试论文规范
- C programming language
- protal主题皮肤开发
- cognos 中文 REPORT STUDIO专业创建用户指南
- 8051单机片教程（大学专用）
- ActionScript 3.0
- 系统分析员备考之UML篇
- YF2410- II 型开发板使用手册
- PureMVC_Implementation_Idioms_and_Best_Practices_cn.pdf
- A Guide to MPEG Fundamentals and Protocol Analysis
- SQL server 2005安装问题汇总
- 开始→运行→输入的命令集锦
- Zend+Framework+入门指南.pdf
- SQL语句的良好编写方法
