IEEE Journal of Solid-State Circuits: August 2019 Issue Overview

需积分: 16 185 浏览量更新于2024-07-16 收藏 94.79MB PDF 举报

"这篇文章来自于《IEEE固态电路期刊》(JSSC-2019-8)，该期刊主要关注固态电路领域的广泛内容，特别是集成电路的晶体管级设计。文章经过同行评审，遵循IEEE PSPB操作手册的规定，采用单盲审稿制度，确保评审的公正性。此外，所有接受的文章在发表前都会进行抄袭检查。本期刊的2019年8月刊包含了多篇研究论文，涉及电气振荡器注入锁定理论、宽带CMOS射频前端、干扰鲁棒检测器、红外超宽带CMOS收发器以及高效SiGe-BiCMOS E带功率放大器等主题。" 在《IEEE固态电路期刊》2019年8月刊中，我们可以深入探讨几个关键的技术知识点： 1. 注入锁定与电气振荡器拉伸理论：分为两部分，由B. Hong和A. Hajimiri撰写。第一部分介绍时间同步建模和注入波形设计，讨论如何通过控制注入信号影响振荡器的行为。第二部分则聚焦于LC振荡器的幅度调制、瞬态行为和频率分频，提供了更深入的理论分析。 2. 宽带CMOS射频前端：J. Fang等人提出的设计适用于直接采样卫星接收机，展示了CMOS技术在射频领域的新应用，提高了接收机的频率范围和性能。 3. 干扰鲁棒检测器-零功耗唤醒接收器：J. Moody等人设计的接收器能够在几乎零功耗状态下工作，同时具有对干扰的抵抗能力，这在低功耗无线通信系统中具有重要意义。 4. 红外超宽带CMOS收发器：G. Lee等人开发的系统适用于高数据速率、低功耗且短距离的通信，利用了CMOS技术实现高速、低功耗的通信方案。 5. 高效率SiGe-BiCMOS E带功率放大器：E. Rahimi等人展示了利用共基级电流钳位技术提高功率放大器效率的方法，这对于射频和微波通信中的高性能功率输出至关重要。这些论文揭示了固态电路和集成电路设计的最新进展，涵盖了从理论到实践的多个层面，对于理解现代电子设备中的核心组件及其优化有着重要的参考价值。

2118 IEEE JOURNAL OF SOLID-STATE CIRCUITS, VOL. 54, NO. 8, AUGUST 2019

Fig. 14. Measured lock ranges of several ring oscillators. The 3-stage and 17-stage rings are single-ended inverter-chain ring oscillators, where a capacitor

loads the output of each inverter and the injection is applied at one of the outputs. The measured free-running oscillation frequencies are 1.32 GHz for the

6-stage differential ring and 1.09 GHz for both the 3-stage and 17-stage single-ended rings.

Fig. 15. Measured lock ranges of a couple relaxation oscillators. The schematic of the differential NMOS astable multivibrator is shown for reference. The

measured free-running oscillation frequencies are 11.9 MHz for the Bose oscillator and 874 MHz for the astable multivibrator.

Fig. 16. Die photograph of the chip, with dimensions 1 × 1mm

various sinusoidal injection amplitudes.

The predicted lock

range is therefore given by (35).

Measurement results for a short, a medium, and a long

ring oscillator are presented in Fig. 14, whereas measure-

ment results for two different relaxation oscillators are shown

in Fig. 15. Observe how the accuracy of the theoretically

predicted lock range (and therefore the assumption of linearity)

still prevails even for a broad range of practical injection

strengths, even those that are comparable to I

max

.Furthermore,

it is noteworthy that the way in which the measured and

predicted lock ranges deviate for larger injection amplitudes

To account for possible measurement error, each oscillator was measured

three separate times. Error bars depicting the entire range of measurements

for each data point are shown in black. (The error bars for most data points

are not noticeable.)

Fig. 17. Simpliﬁed schematic showing an example of how the injection

circuitry’s bias current I

bias

, which dictates the power consumption, is con-

verted into the injected current i

inj

. Since the instantaneous injection current

inj

(t) is bound (in magnitude) by the tail current, so too is its rms amplitude:

rms

≤ I

bias

Fig. 18. For a ﬁxed injection power I

rms

, the injection waveform that

optimizes the lock range is one whose shape matches that of the ISF.

is reproducible in simulation as well [48]. A die micrograph

of the fabricated oscillators is shown in Fig. 16.

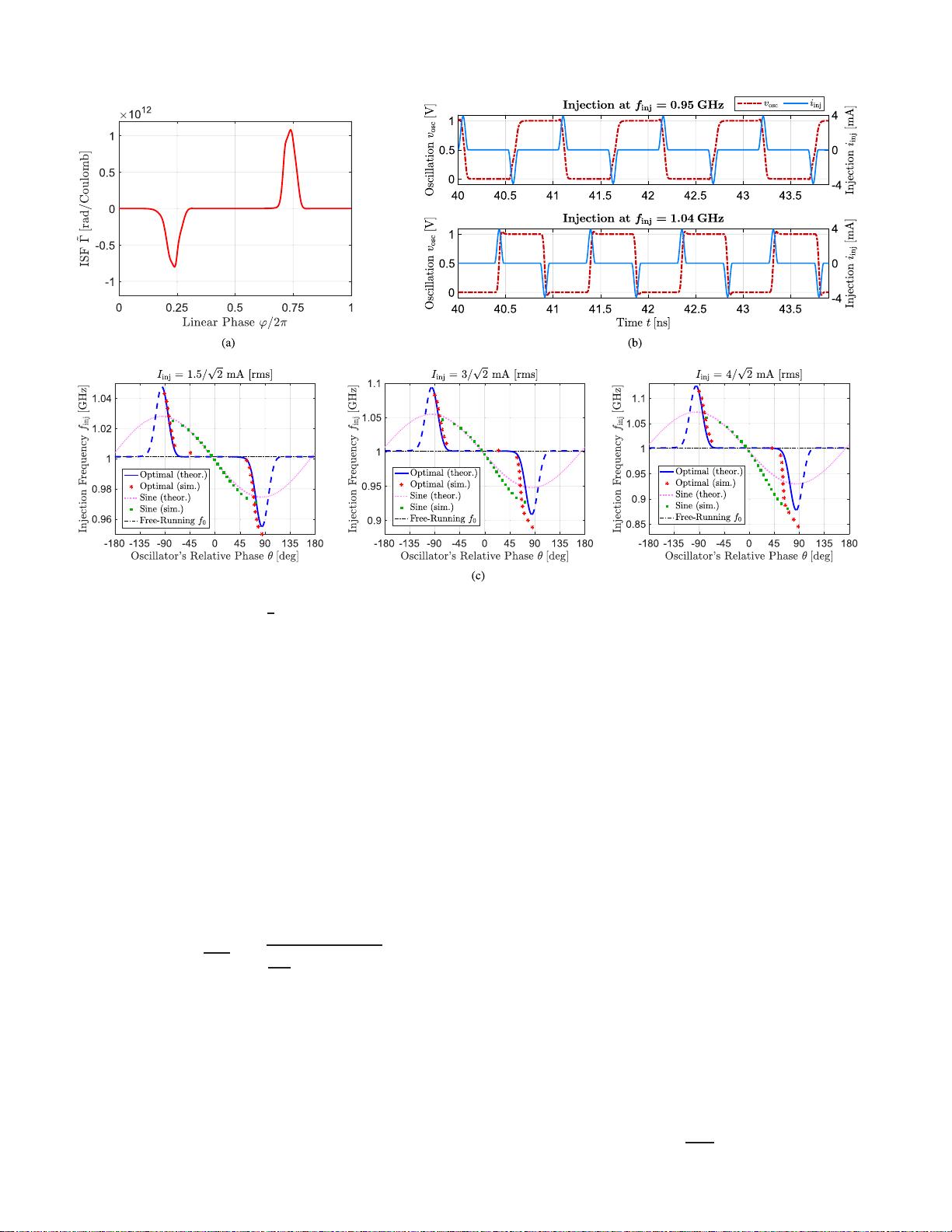
HONG AND HAJIMIRI: GENERAL THEORY OF INJECTION LOCKING AND PULLING IN ELECTRICAL OSCILLATORS—I 2119

Fig. 19. Enhancing the lock range by injecting a waveform which matches the shape of the ISF. (a) ISF. (b) Time-domain plot at the lower and upper edges

of the lock range (with I

rms

= 1.5/

√

2 mA). (c) Lock characteristics with increasing injection strength. The simulated and theoretical lock characteristics for

both a sinusoidal injection and the pulsed injection shown in (b) are plotted. Note that I

max

= 0.72 mA.

VI. DESIGN IMPLICATIONS—SHAPING THE INJECTION

As alluded to in Fig. 7, the shape of the injection seems

to play a role in the frequency shift that is affected. In this

section, we will explore how this phenomenon can be used

to optimize the lock range. However, since the lock range

increases with the injection strength, we must ﬁrst con-

strain the size of the injection current in a meaningful way.

Although this may be straightforward to do for relatively

simple waveforms like sine or square waves, it becomes

difﬁcult to ascertain the injection “amplitude” when a com-

plicated assortment of harmonics is present. A more universal

measure of the injection size which accounts for power in

all harmonics is the root-mean-square (rms) of the injection

current

rms

≡





inj





inj



inj

(t)

dt. (43)

At ﬁrst glance, the rms injection current might also seem to

represent the average power injected into the oscillator, but

this assumes a ﬁxed load, which is rarely the case in practice

for the input impedances of actual oscillators. However, from

a different and more practical perspective, I

rms

actually serves

as a good measure of the average power consumption of the

injection circuitry itself.

To understand why, consider the differential transistor pair

in Fig. 17, which commutates a static tail bias current I

bias

In the most efﬁcient scenario, the differential injected cur-

rent i

inj

strictly alternates between ±I

bias

. This injection

current has an rms amplitude of I

rms

= I

bias

,whichis

proportional to the static power consumption of the injec-

tion circuit: I

bias

. In reality, however, the circuit cannot

transition between ±I

bias

instantaneously, resulting in time

periods where the circuit is injecting less current. Thus,

the average power consumption of the injection circuitry is

usually at least I

rms

In summary, the rms injection current is a meaningful

metric to consider because of its physical signiﬁcance from

a design standpoint—the minimum average power drawn

by the injection circuitry scales with I

rms

—and because it

serves as an unambiguous deﬁnition of the injection amplitude

regardless of the shape of the injection waveform. With this

in mind, we are now in a position to think about how we

can broaden the lock range by shaping the injection current

for a ﬁxed “injection power,” or more precisely, a ﬁxed rms

amplitude I

rms

One can show using the Cauchy–Schwarz inequality that the

maximum lock range is obtained when the injection waveform

is proportional to the ISF [49]. Thus, the optimal injection

waveform i

∗

inj,0

is given by

∗

inj,0

(x) =±

rms



rms

(x) (44)

2120 IEEE JOURNAL OF SOLID-STATE CIRCUITS, VOL. 54, NO. 8, AUGUST 2019

where the positive solution optimizes the upper lock range

and the negative solution optimizes the lower lock range.

The optimal (absolute) lock range is therefore easily

calculated to be

∗

= I

rms



rms

. (45)

Effectively, the lock range is maximized when the injec-

tion has the same shape as the ISF. This idea is illustrated

conceptually in the cartoon of Fig. 18. After all, the ISF

is a measure of the sensitivity of the oscillator’s phase to

external disturbances as a function of when the disturbance

is applied. Hence, an injection current which “looks like” the

ISF is more active at points along the oscillation cycle that

are more sensitive to the injection. We can also extend this

intuition to a more physical level. For a given injection node,

the oscillator’s phase is more impressionable—and the ISF

is larger—when the voltage at that node is changing more

rapidly.

It therefore makes sense that displacing charge at

the injection node during those times will advance or retard

the oscillation more effectively.

Fig. 19 demonstrates this principle in action for a 17-stage

single-ended ring oscillator. As we can see, the ISF consists

of two tall, narrow pulses, which correspond to the sharp

upward and downward transitions in the ring’s node voltages.

By emulating this shape in the injection waveform, the lock

range is almost doubled compared to a sinusoidal injection of

the same power. In particular, Fig. 19(b) shows how the pulses

in the injection target the transitions in the oscillation voltage

at the edges of the lock range. At the lower edge, for example,

notice how the injection current makes the voltage transitions

less steep, thereby slowing down the oscillation.

In closing, we point out that there undoubtedly are scenarios

where it is instead desirable to minimize the lock range in order

to reduce coupling effects between oscillators, for example.

This problem, which may warrant further investigation, can

also be attacked with the framework developed in this paper.

VII. C

ONCLUSION

This paper presented a time-synchronous theory of injec-

tion locking and pulling in electrical oscillators, applicable

to oscillators of any topology and periodic injections of

arbitrary shape. A general mathematical characterization of

autonomy and periodic time variance—the modus operandi

of any oscillator—was used to derive a ﬁrst-order differential

equation for the time-domain behavior of the phase of a

periodically disturbed oscillator. The framework revealed that

the lock range is enhanced by matching the shape of the

injection waveform to that of the oscillator’s ISF. Various

simulation and measurement results support the proposed

theory.

CKNOWLEDGMENT

The authors would like to thank P. Khial and R. Fatemi of

the California Institute of Technology (Caltech) for technical

To the ﬁrst order, this is embodied by the numerator of the orthogonal-

state-variable-based formula for the ISF [42, eq. (36)], [48, eq. (4.6)].

discussions, M. Gal-Katziri and A. White of Caltech for

extensive assistance with measurements, and M. Gal-Katziri

for his design of the comparator used as the Schmitt trigger

in the implementation of the fabricated Bose oscillator.

EFERENCES

[1] B. Hong and A. Hajimiri, “A general theory of injection locking and

pulling in electrical oscillators—Part II: Amplitude modulation in LC

oscillators, transient behavior, and frequency division,” IEEE J. Solid-

State Circuits, vol. 54, no. 8, pp. 2122–2139, Aug. 2019.

[2] J. Lee and M. Liu, “A 20-Gb/s burst-mode clock and data recovery

circuit using injection-locking technique,” IEEE J. Solid-State Circuits,

vol. 43, no. 3, pp. 619–630, Mar. 2008.

[3] M. Hossain and A. C. Carusone, “CMOS oscillators for clock distribu-

tion and injection-locked deskew,” IEEE J. Solid-State Circuits, vol. 44,

no. 8, pp. 2138–2153, Aug. 2009.

[4] S. Shekhar et al., “Strong injection locking in low-Q LC oscillators:

Modeling and application in a forwarded-clock I/O receiver,” IEEE

Trans. Circuits Syst. I, Reg. Papers, vol. 56, no. 8, Aug. 2009.

[5] H. R. Rategh and T. H. Lee, “Superharmonic injection-locked frequency

dividers,” IEEE J. Solid-State Circuits, vol. 34, no. 6, pp. 813–821,

Jun. 1999.

[6] H. Wu and A. Hajimiri, “A 19 GHz 0.5 mW 0.35 μm CMOS fre-

quency divider with shunt-peaking locking-range enhancement,” in IEEE

Int. Solid-State Circuits Conf. (ISSCC) Dig. Tech. Papers, Feb. 2001,

pp. 412–413.

[7] A. Imani and H. Hashemi, “Distributed injection-locked frequency

dividers,” IEEE J. Solid-State Circuits, vol. 52, no. 8, pp. 2083–2093,

Aug. 2017.

[8] W. L. Chan and J. R. Long, “A 56–65 GHz injection-locked frequency

tripler with quadrature outputs in 90-nm CMOS,” IEEE J. Solid-State

Circuits, vol. 43, no. 12, pp. 2739–2746, Dec. 2008.

[9] E. Monaco, M. Pozzoni, F. Svelto, and A. Mazzanti, “Injection-locked

CMOS frequency doublers for μ-wave and mm-wave applications,”

IEEE J. Solid-State Circuits, vol. 45, no. 8, pp. 1565–1574, Aug. 2010.

[10] A. Rofougaran, J. Rael, M. Rofougaran, and A. Abidi, “A 900 MHz

CMOS LC-oscillator with quadrature outputs,” in IEEE Int. Solid-State

Circuits Conf. (ISSCC) Dig. Tech. Papers, Feb. 1996, pp. 392–393.

[11] P. Kinget, R. Melville, D. Long, and V. Gopinathan, “An injection-

locking scheme for precision quadrature generation,” IEEE J. Solid-State

Circuits, vol. 37, no. 7, pp. 845–851, Jul. 2002.

[12] L. Romanò, S. Levantino, C. Samori, and A. L. Lacaita, “Multiphase

LC oscillators,” IEEE Trans. Circuits Syst. I, Reg. Papers, vol. 53, no. 7,

pp. 1579–1588, Jul. 2006.

[13] M. Raj, S. Saeedi, and A. Emami, “A wideband injection locked

quadrature clock generation and distribution technique for an energy-

proportional 16–32 Gb/s optical receiver in 28 nm FDSOI CMOS,” IEEE

J. Solid-State Circuits, vol. 51, no. 10, pp. 2446–2462, Oct. 2016.

[14] R. A. York, P. Liao, and J. J. Lynch, “Oscillator array dynamics with

broadband N-port coupling networks,” IEEE Trans. Microw. Theory

Techn., vol. 42, no. 11, pp. 2040–2045, Nov. 1994.

[15] R. A. York and T. Itoh, “Injection- and phase-locking techniques for

beam control,” IEEE Trans. Microw. Theory Techn., vol. 46, no. 11,

pp. 1920–1929, Nov. 1998.

[16] B. Razavi, “A study of injection locking and pulling in oscillators,” IEEE

J. Solid-State Circuits, vol. 39, no. 9, pp. 1415–1424, Sep. 2004.

[17] J. H. Vincent, “On some experiments in which two neighboring main-

tained oscillatory circuits affect a resonating circuit,” Proc. Phys. Soc.

London, vol. 32, no. 1, pp. 84–91, 1919.

[18] E. V. Appleton, “The automatic synchronization of triode oscillators,”

Cambridge Phil. Soc., vol. 21, no. 3, pp. 231–248, 1922.

[19] B. van der Pol, “Forced oscillations in a circuit with non-linear resis-

tance. (Reception with reactive triode),” London, Edinburgh, Dublin

Philosoph. Mag. J. Sci., vol. 3, no. 13, pp. 65–80, Jan. 1927.

[20] R. Adler, “A study of locking phenomena in oscillators,” Proc. IRE,

vol. 34, no. 6, pp. 351–357, Jun. 1946.

[21] R. D. Huntoon and A. Weiss, “Synchronization of oscillators,” Proc.

IRE, vol. 35, no. 12, pp. 1415–1423, Dec. 1947.

[22] H. L. Stover, “Theoretical explanation for the output spectra of unlocked

driven oscillators,” Proc. IEEE, vol. 54, no. 2, pp. 310–311, Feb. 1966.

[23] M. Armand, “On the output spectrum of unlocked driven oscillators,”

Proc. IEEE, vol. 57, no. 5, pp. 798–799, May 1969.

[24] K. Kurokawa, “Injection locking of microwave solid-state oscillators,”

Proc. IEEE, vol. 61, no. 10, pp. 1386–1410, Oct. 1973.

HONG AND HAJIMIRI: GENERAL THEORY OF INJECTION LOCKING AND PULLING IN ELECTRICAL OSCILLATORS—I 2121

[25] S. Verma, H. R. Rategh, and T. H. Lee, “A uniﬁed model for injection-

locked frequency dividers,” IEEE J. Solid-State Circuits, vol. 38, no. 6,

pp. 1015–1027, Jun. 2003.

[26] L. J. Paciorek, “Injection locking of oscillators,” Proc. IEEE, vol. 53,

no. 11, pp. 1723–1727, Nov. 1965.

[27] A. Mirzaei, M. E. Heidari, R. Bagheri, S. Chehrazi, and A. A. Abidi,

“The quadrature LC oscillator: A complete portrait based on

injection locking,” IEEE J. Solid-State Circuits, vol. 42, no. 9,

pp. 1916–1932, Sep. 2007.

[28] B. Hong and A. Hajimiri, “A phasor-based analysis of sinusoidal

injection locking in LC and ring oscillators,” IEEE Trans. Circuits Syst.

I, Reg. Papers, vol. 66, no. 1, pp. 355–368, Jan. 2019.

[29] A. Mirzaei and H. Darabi, “Mutual pulling between two oscillators,”

IEEE J. Solid-State Circuits, vol. 49, no. 2, pp. 360–372, Feb. 2014.

[30] X. Lai and J. Roychowdhury, “Capturing oscillator injection locking

via nonlinear phase-domain macromodels,” IEEE Trans. Microw. Theory

Techn., vol. 52, no. 9, pp. 2251–2261, Sep. 2004.

[31] X. Lai and J. Roychowdhury, “Automated oscillator macromodelling

techniques for capturing amplitude variations and injection locking,” in

Proc. IEEE/ACM Int. Conf. Comput.-Aided Des. (ICCAD), Nov. 2004,

pp. 687–694.

[32] G. R. Gangasani and P. R. Kinget, “Time-domain model for injection

locking in nonharmonic oscillators,” IEEETrans.CircuitsSyst.I,Reg.

Papers, vol. 55, no. 6, pp. 1648–1658, Jul. 2008.

[33] H. A. Tanaka, A. Hasegawa, H. Mizuno, and T. Endo, “Synchronizability

of distributed clock oscillators,” IEEE Trans. Circuits Syst. I, Fundam.

Theory Appl., vol. 49, no. 9, pp. 1271–1278, Sep. 2002.

[34] P. Maffezzoni, “Analysis of oscillator injection locking through phase-

domain impulse-response,” IEEE Trans. Circuits Syst. I, Reg. Papers,

vol. 55, no. 5, pp. 1297–1305, Jun. 2008.

[35] T. Nagashima, X. Wei, H.-A. Tanaka, and H. Sekiya, “Locking range

derivations for injection-locked class-E oscillator applying phase reduc-

tion theory,” IEEE Trans. Circuits Syst. I, Reg. Papers, vol. 61, no. 10,

pp. 2904–2911, Oct. 2014.

[36] M. A. Tiebout, “A CMOS direct injection-locked oscillator topology

as high-frequency low-power frequency divider,” IEEE J. Solid-State

Circuits, vol. 39, no. 7, pp. 1170–1174, Jul. 2004.

[37] C.-C. Chen, H.-W. Tsao, and H. Wang, “Design and analysis of CMOS

frequency dividers with wide input locking ranges,” IEEE Trans. Microw.

Theory Techn., vol. 57, no. 12, pp. 3060–3069, Dec. 2009.

[38] R. J. Betancourt-Zamora, S. Verma, and T. H. Lee, “1-GHz and

2.8-GHz CMOS injection-locked ring oscillator prescalers,” in Symp.

VLSI Circuits Dig. Tech. Papers, Jun. 2001, pp. 47–50.

[39] J. C. Chien and L. H. Lu, “Analysis and design of wideband injection-

locked ring oscillators with multiple-input injection,” IEEE J. Solid-State

Circuits, vol. 42, no. 9, pp. 1906–1915, Sep. 2007.

[40] A. Mirzaei, M. E. Heidari, R. Bagheri, and A. A. Abidi, “Multi-

phase injection widens lock range of ring-oscillator-based frequency

dividers,” IEEE J. Solid-State Circuits, vol. 43, no. 3, pp. 656–671,

Mar. 2008.

[41] D. Dunwell and A. C. Carusone, “Modeling oscillator injection locking

using the phase domain response,” IEEETrans.CircuitsSyst.I,Reg.

Papers, vol. 60, no. 11, pp. 2823–2833, Nov. 2013.

[42] A. Hajimiri and T. H. Lee, “A general theory of phase noise in electrical

oscillators,” IEEE J. Solid-State Circuits, vol. 33, no. 2, pp. 179–194,

Feb. 1998.

[43] A. Hajimiri and T. H. Lee, “Design issues in CMOS differential LC

oscillators,” IEEE J. Solid-State Circuits

, vol. 34, no. 5, pp. 717–724,

May 1999.

[44] A. Hajimiri, S. Limotyrakis, and T. H. Lee, “Jitter and phase noise

in ring oscillators,” IEEE J. Solid-State Circuits, vol. 34, no. 6,

pp. 790–804, Jun. 1999.

[45] A. Hajimiri and T. H. Lee, The Design of Low Noise Oscillators.

Norwell, MA, USA: Kluwer, 1999.

[46] S. Levantino, P. Maffezzoni, F. Pepe, A. Bonfanti, C. Samori, and

A. L. Lacaita, “Efﬁcient calculation of the impulse sensitivity function

in oscillators,” IEEE Trans. Circuits Syst. II, Exp. Briefs, vol. 59, no. 10,

pp. 628–632, Oct. 2012.

[47] J. A. Sanders, F. Verhulst, and J. Murdock, Averaging Methods in

Nonlinear Dynamical Systems. New York, NY, USA: Springer, 2007.

[48] B. Hong, “Periodically disturbed oscillators,” Ph.D. dissertation, Dept.

Elect. Eng., California Inst. Technol., Pasadena, CA, USA, 2018. doi:

10.7907/W0A7-4258.

[49] A. W. Naylor and G. R. Sell, Linear Operator Theory in Engineering

and Science. New York, NY, USA: Springer, 2000.

Brian Hong (S’11) received the B.S. degree (summa

cum laude) in electrical engineering from the Uni-

versity of California at Los Angeles (UCLA),

Los Angeles, CA, USA, in 2013, and the M.S.

and Ph.D. degrees in electrical engineering from

the California Institute of Technology (Caltech),

Pasadena, CA, USA, in 2014 and 2018, respectively.

His research interests include the mathematical

and physical modeling of various electronic systems,

with a particular emphasis on the theory of injection

locking and pulling in electrical oscillators.

Dr. Hong was a recipient of the JPL Undergraduate Scholarship in 2008,

the Rose Hills Foundation Fellowship in 2013, and the Analog Devices

Outstanding Student Designer Award in 2014.

Ali Hajimiri (S’94–M’98–SM’09–F’10) received

the B.S. degree in electronics engineering from the

Sharif University of Technology, Tehran, Iran, in

1994, and the M.S. and Ph.D. degrees in electrical

engineering from Stanford University, Stanford, CA,

USA, in 1996 and 1998, respectively.

From 1993 to 1994, he was with Philips Semicon-

ductors, Sunnyvale, CA, USA, where he worked on a

BiCMOS chipset for Global System for Mobile com-

munications (GSM) and cellular units. In 1995, he

was with Sun Microsystems, Sunnyvale, working on

the UltraSPARC microprocessors cache RAM design methodology. In 1997,

he was with Lucent Technologies (Bell Labs), Murray Hill, NJ, USA, where

he investigated low-phase-noise integrated oscillators. In 1998, he joined

the faculty of the California Institute of Technology (Caltech), Pasadena,

CA, USA, where he is currently a Bren Professor of electrical engineering

and medical engineering, the Director of the Microelectronics Laboratory,

and a Co-Director of the Space Solar Power Project. In 2002, he was a

Co-Founder of Axiom Microdevices Inc., Irvine, CA, USA, whose fully inte-

grated CMOS PA shipped close to 400 million units and which was acquired

by Skyworks Inc. in 2009. He is the author of The Design of Low Noise

Oscillators (Boston, MA, USA: Springer), and he has authored or coauthored

more than 200 refereed journal and conference technical articles. He holds

more than 100 U.S. patents and has many more pending applications. His

research interests include high-speed and high-frequency integrated circuits

for applications in sensors, photonics, biomedical devices, and communication

systems.

Dr. Hajimiri is a fellow of the National Academy of Inventors. He has

served as a member of the Technical Program Committee for the IEEE

International Solid-State Circuits Conference (ISSCC) and the International

Conference on Computer-Aided Design (ICCAD). He was selected to the

TR35 top innovator’s list (formerly TR100) in 2004. He has received a

number of teaching awards from Caltech, including the Richard P. Feynman

Prize for Excellence in Teaching, the Graduate Student Council Teaching

and Mentoring Award, and the Associated Students of the California Institute

of Technology (ASCIT) Undergraduate Excellence in Teaching Award. He

was the Gold Medal winner of the National Physics Competition, Iran,

and the Bronze Medal winner of the 21st International Physics Olympiad,

Groningen, the Netherlands. He has been recognized as one of the top-

10 contributors to ISSCC. He was a co-recipient of the IEEE Journal of Solid-

State Circuits Best Paper Award of 2004, the ISSCC Jack Kilby Outstanding

Paper Award, the RFIC Best Paper Award, and the CICC Best Paper Award

(twice). He has received the National Science Foundation CAREER Award

and the Okawa Foundation Award, and he is a three-time winner of the IBM

Faculty Partnership Award. He has served as an Associate Editor for the

IEEE J

OURNAL OF SOLID-STATE CIRCUITS and the IEEE TRANSACTIONS

CIRCUITS AND SYSTEMS II: EXPRESS BRIEFS,aGuestEditorofthe

IEEE T

RANSACTIONS ON MICROWAVE THEORY AND TECHNIQUES,and

a member of the Guest Editorial Board for the Transactions on Electronics

of the Institute of Electronics, Information and Communication Engineers

(IEICE), Japan. He has also served as a Distinguished Lecturer for the IEEE

Solid-State and Microwave Societies.

2122 IEEE JOURNAL OF SOLID-STATE CIRCUITS, VOL. 54, NO. 8, AUGUST 2019

A General Theory of Injection Locking and Pulling

in Electrical Oscillators—Part II: Amplitude

Modulation in LC Oscillators, Transient

Behavior, and Frequency Division

Brian Hong , Student Member, IEEE, and Ali Hajimiri , Fellow, IEEE

Abstract—A number of specialized topics within the theory of

injection locking and pulling are addressed. The material builds

on our impulse sensitivity function (ISF)-based, time-synchronous

model of electrical oscillators under the inﬂuence of a periodic

injection. First, we show how the accuracy of this model for

LC oscillators under large injection is greatly enhanced by

accounting for the injection’s effect on the oscillation amplitude.

In doing so, we capture the asymmetry of the lock range as well

as the distinct behaviors exhibited by different LC oscillator

topologies. Existing LC oscillator injection locking and pulling

theories in the literature are subsumed as special cases. Next, a

transient analysis of the dynamics of injection pulling is carried

out, both within and outside of the lock range. Finally, we show

how our existing framework naturally accommodates locking

onto superharmonic and subharmonic injections, leading to sev-

eral design considerations for injection-locked frequency dividers

(ILFDs) and the implementation of a low-power dual-modulus

prescaler from an injection-locked ring oscillator. Our theoretical

conclusions are supported by simulations and experimental data

from a variety of LC, ring, and relaxation oscillators.

Index Terms— Adler’s equation, amplitude perturbation func-

tion (APF), dual-modulus prescaler, impulse sensitivity function

(ISF), injection locking, injection pulling, injection-locked fre-

quency divider (ILFD), lock characteristic, lock range, oscillator.

I. INTRODUCTION

HIS paper deals with several advanced topics arising out

of the ISF-based, time-synchronous model of injection

locking, and pulling introduced in the preceding companion

paper [1]. Although a number of authors have addressed

various, speciﬁc aspects of these topics [2]–[24], absent thus

far is a unifying framework capable of casting a wide net

over all of them with the level of generality achieved by

our model. The material is organized as follows. Section II

introduces notation and reviews the time-synchronous model

presented in [1]. Section III examines the issue of amplitude

Manuscript received November 17, 2018; revised February 20, 2019;

accepted March 25, 2019. Date of current version July 23, 2019. This paper

was approved by Associate Editor Pietro Andreani. This work was supported

by the Air Force Ofﬁce of Scientiﬁc Research (AFOSR) under MURI Grant

FA9550-16-1-0566. (Corresponding author: Brian Hong.)

The authors are with the Department of Electrical Engineering,

California Institute of Technology, Pasadena, CA 91125 USA (e-mail:

bhong@caltech.edu; hajimiri@caltech.edu).

Color versions of one or more of the ﬁgures in this paper are available

online at http://ieeexplore.ieee.org.

Digital Object Identiﬁer 10.1109/JSSC.2019.2908763

modulation caused by the injection, leading to signiﬁcant

improvements in our model’s predictive power for LC oscil-

lators under large injection. Section IV generalizes the math-

ematical structure of the framework to allow for an arbitrary

rational relationship between the injection and the locked

oscillation frequencies (e.g., superharmonic and subharmonic

locking). Section V focuses on transient behavior: issues such

as the pull-in process and the dynamics of injection pulling

are covered. Next, Section VI introduces a new ﬁgure of

merit that enables meaningful comparisons between the lock

ranges of different oscillators, and Section VII explores several

techniques for enhancing an oscillator’s ability to serve as

an injection-locked frequency divider (ILFD). Finally, these

techniques are used in Section VIII to design a low-power,

ring-oscillator-based prescaler whose division ratio can be

dynamically toggled between 2 and 3 over a broad range of

reference frequencies.

II. R

EVIEW OF THE TIME-SYNCHRONOUS MODEL

A. Basic Deﬁnitions

The oscillation voltage of an oscillator under injection can

generally be expressed as

osc

(t) =[1 + A(t)]·v

[ω

t + φ(t)] (1)

where v

(·) is a 2π-periodic oscillation waveform and ω

≡

2π/T

is the (noiseless) free-running (angular) frequency

of oscillation. Disturbances in the waveform, A(t),andin

the phase, φ(t), are caused by the injection of a periodic

current

inj

(t) ≡ i

inj,0

(ω

inj

t) (2)

where i

inj,0

(·) is a 2π-periodic injection waveform and

inj

≡ 2π/T

inj

is the injection frequency.

In injection locking and pulling scenarios, it is more suitable

to represent the argument of the oscillation waveform—known

as the oscillator’s total phase ϕ(t)—in the frame of reference

of the injection signal:

ϕ(t) ≡ ω

t + φ(t) ≡ ω

inj

t + θ(t) (3)

where θ(t) is the relative phase of the oscillator with respect

to the injection.

See http://www.ieee.org/publications_standards/publications/rights/index.html for more information.

剩余255页未读，继续阅读

netshell

粉丝: 11
资源: 185

IEEE Journal of Solid-State Circuits: August 2019 Issue Overview

IEEE Journal of Solid-State Circuits: Transistor-Level IC Design and Peer Review

2019年欧洲固体电路会议特辑：先进7nm FinFET V带隙参考等创新技术

2019年IEEE固态电路期刊特刊精华：微电子器件与设计突破

JSSC-2019-11

JSSC-2019-10

JSSC-2019-9

JSSC-2019-12

JSSC-2019-2

JSSC-2019-3

JSSC-2019-1

最新资源