正态分布乘积等于正态分布的证明_正态分布的乘积

正态分布乘积

1星需积分: 47 119 浏览量更新于2023-03-16 5 收藏 179KB PDF 举报

身份认证购VIP最低享 7 折!

领优惠券(最高得80元）

资源详情

资源推荐

Tina Memo No. 2003-003

Internal Report

Products and Convolutions of Gaussian Probability Density

Functions

P.A. Bromiley

Last updated

14 / 8 / 2014

Imaging Sciences Research Group, Institute of Population Health,

School of Medicine, University of Manchester,

Stopford Building, Oxford Road,

Manchester, M13 9PT.

Products and Convolutions of Gaussian Probability

Density Functions

P. A. Bromiley

Imaging Sciences Research Group, Institute of Population Health,

School of Medicine, University of Manchester,

Manchester, M13 9PT, UK

paul.bromiley@manchester.ac.uk

Abstract

It is well known that the product and the convolution of Gaussian probability density functions (PDFs)

are also Gaussian functions. This document provides proofs of this for several cases; the product of

two univariate Gaussian PDFs, the product of an arbitrary number of univariate Gaussian PDFs, the

product of an arbitrary number of multivariate Gaussian PDFs , and the convolution of two univari-

ate Gaussian PDFs. These results are useful in calculating the eﬀects of smoothing applied as an

intermediate step in various algorithms.

1 The Product of Two Univariate Gaussian PDFs

Let f(x) and g(x) be Gaussian PDFs with arbitrary means µ

and µ

and standard deviations σ

and σ

f(x) =

√

2πσ

−

(x−µ

)

2σ

and g(x) =

√

2πσ

−

(x−µ

)

2σ

Their product is

f(x)g(x) =

2πσ

−



(x−µ

)

2σ

(x−µ

)

2σ



Examine the term in the exponent

β =

(x − µ

)

2σ

(x − µ

)

2σ

Expanding the two quadratics and collecting te r ms in powers of x gives

β =

(σ

+ σ

− 2(µ

+ µ

)x + µ

+ µ

2σ

Dividing through by the coeﬃcient of x

gives

β =

− 2

+µ

+σ

x +

+µ

+σ

This is again a quadratic in x, and so Eq. 2 is a Gaussian function. Compare the terms in Eq. 5 to a the usual

Gaussian form

P (x) =

√

2πσ

−

(x−µ)

2σ

√

2πσ

−

−2µx+µ

)

2σ

Since a term ǫ that is independent of x can be added to complete th e square in β, this is suﬃc ent to complete the

proof in cases where the normalisation can be ignored. The product of two Gaussian PDFs is proportional to a

Gaussian PDF with a mean that is half the coeﬃcient of x in Eq. 5 and a standard deviation that is the square

root of half of the denominator i.e.

+ σ

and µ

+ µ

+ σ

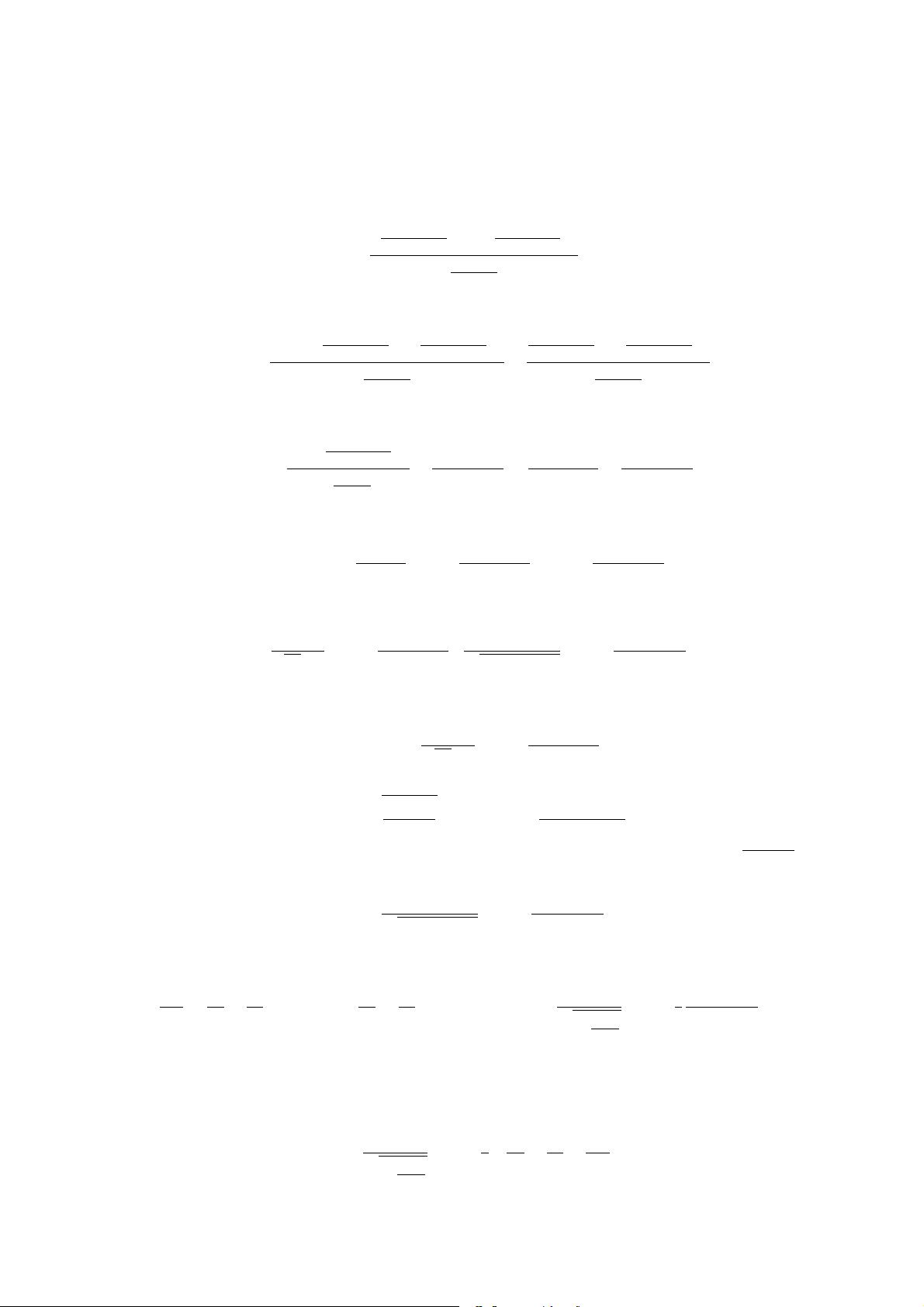
i.e. the variance σ

is twice the harmonic mean of the individual variances σ

and σ

, and the mean µ

is the

sum of the individual means µ

and µ

weighted by their variances. In general, the product is not itself a PDF

as, due to the presence of the scaling factor, it will not have the corr e ct nor malis ation.

The product f(x)g(x) can now be written in the usual Gaus s ian form directly, with an unknown scaling constant

(this may be suﬃcient in cases where renormalisation can be applied). Alternatively, proceeding from Eq. 5,

suppose that ǫ is the term required to complete the square in β i.e.

ǫ =



+µ

+σ



−



+µ

+σ



2σ

(σ

+σ

)

= 0

Adding this term to β gives

β =

− 2x

+µ

+σ



+µ

+σ



2σ

(σ

+σ

)

+µ

+σ

−



+µ

+σ



2σ

(σ

+σ

)

After some manipulation, this reduces to

β =



x −

+µ

+σ



+σ

(µ

− µ

)

2(σ

+ σ

)

(x − µ

)

2σ

(µ

− µ

)

2(σ

+ σ

)

Substituting back into Eq. 2 gives

f(x)g(x) =

2πσ

exp

−

(x − µ

)

2σ

exp

−

(µ

− µ

)

2(σ

+ σ

)

Multiplying by σ

/σ

and rearranging gives

√

2πσ

exp

−

(x − µ

)

2σ

2π(σ

+ σ

)

exp

−

(µ

− µ

)

2(σ

+ σ

)

Therefore, the product of two Gaussians PDFs f(x) and g(x) is a scaled Gaussian PDF

f(x)g(x) =

√

2πσ

exp

−

(x − µ

)

2σ

where

+ σ

and µ

+ µ

+ σ

(1)

and the scaling factor S is itself a Gaussian PDF on both µ

and µ

with standard deviation

+ σ

2π(σ

+ σ

)

exp

−

(µ

− µ

)

2(σ

+ σ

)

These can be wr i tt en more conveniently as

, µ

and S

2π

f g

exp

−

(µ

− µ

)

(2)

It is much easier to generate a proof by induction for the scaling factor of products of larger numbers of Gaussians

if it is written in the form of a sum of terms, each of which involves a single subscript i.e. the parameters of a

single Gaussian PDF. Appendix A provides the necessary proof, giving

2π

f g

exp

−

(3)

剩余12页未读，继续阅读

huangkai222

粉丝: 2
资源: 1

会员权益专享

正态分布乘积等于正态分布的证明

Gaussian09 + Caussian View +海量资源

python 高斯分布二维高斯分布代码

Gaussian98

分散と平均が異なる正規分布の積の分布は​正規分布となるのか。:具有不同方差和均值的正态分布的乘积分布通过数值实验得到阐明。-matlab开发

n个相对独立并服从正态分布的乘积

二维正态分布的不相关性和独立性

用r求正态分布的最大似然估计

多元正态分布的似然函数

isserlis定理证明

建立X是p元标准正态分布，Y是0，1，回归系数b是（1，2，3…p的参数最大似然估计优化模型Porbit模型的具体表达式

noise = 0.05 * (math.sqrt(-2 * math.log(u1)) * math.cos(2 * math.pi * u2))什么意思

最大似然估计 窃电数据

随机向量 x服从 p 元正态分布 ，回归系数b 从上述模型中产生独立同分布观测样本 .用最小二乘估计b的 python代码（不使用minize函数和optimistic包并且产生结果）

likelihood = prod(normpdf(C, data(2:end, 3), data(2:end, 4)));

用贝叶斯求后验分布 matlab

解析这段代码：y = np.sin(x) + 0.1 * np.random.randn(100)

pi_pdf = @(x) normpdf(x,mu,sigma); 当x=rand(9,3),时怎么计算？

会员权益专享

最新资源

分散と平均が異なる正規分布の積の分布は正規分布となるのか。:具有不同方差和均值的正态分布的乘积分布通过数值实验得到阐明。-matlab开发

最大似然估计窃电数据

随机向量 x服从 p 元正态分布，回归系数b 从上述模型中产生独立同分布观测样本 .用最小二乘估计b的 python代码（不使用minize函数和optimistic包并且产生结果）